This article is a runner up in the general public category of the Plus new writers award 2008

Georg Cantor
Modern ideas about infinity provide a wonderful playground for mathematicians and philosophers. I want to lead you through this garden of intellectual delights and tell you about the man who created it — Georg Cantor.
Cantor was born in Russia in 1845.When he was eleven years old his family moved to Germany and he suffered from a wistful homesickness for the rest of his life. At school he had a great talent for the violin, but his real gift and passion was for mathematics. As a university student in Berlin he was president of the mathematical society and met his friends every week in a wine house...to discuss mathematics! In 1872 he was appointed extraordinary professor at Halle, and began his life-long study of infinite sets.
The simplest infinite set is the set of natural numbers
![\[ \mathbb {N} = \{ 1,2,3,...\} . \]](/MI/0717a0a108f7a46756062a627db77257/images/img-0001.png) |
![\[ \{ 101,102,103,104,...\} . \]](/MI/b00f46a16e9ddd742576f584aacb45c6/images/img-0001.png) |
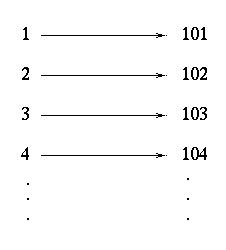
The diagram shows that there is a one-to-one correspondence, or bijection, between the two sets. Since each element in 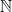 pairs off with one element in
pairs off with one element in 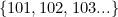 and vice versa, the sets must have the same "size", or, to use Cantor's language, the same cardinality.
and vice versa, the sets must have the same "size", or, to use Cantor's language, the same cardinality.
Using a bijection to compare the size of two infinite sets was one of Cantor's most fruitful ideas. The cardinality of 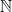 is not of course an ordinary number, since
is not of course an ordinary number, since 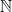 is infinite. It's nevertheless a mathematical object that deserves a name, so Cantor represented it by the first letter in the Hebrew alphabet,
is infinite. It's nevertheless a mathematical object that deserves a name, so Cantor represented it by the first letter in the Hebrew alphabet, 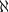 ,
(pronounce "aleph") with a subscript of zero:
,
(pronounce "aleph") with a subscript of zero: 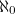 ,
,
Infinite infinities
In 1872 Cantor started to think about those numbers that can be represented as fractions, known as rational numbers. These include the natural numbers since every whole number n can be written as the fraction n/1. What's more, between any two rational numbers, no matter how close, there are infinitely many other rational numbers. And then, between any two of these new and more closely packed rational numbers there is again an infinite number of rational numbers — this seems to show that there are hugely more rational numbers than natural numbers, but Cantor proved that in fact they are equally numerous. An ingenious way to see this is to write down the fractions in an array:

Take a moment to convince yourself that the array contains every single positive rational number. Numbers occur more than once as equivalent fractions, for example 1= 1/1 = 2/2, etc. Now we create a list of fractions by starting in the top left corner of the array and moving up and down diagonally:
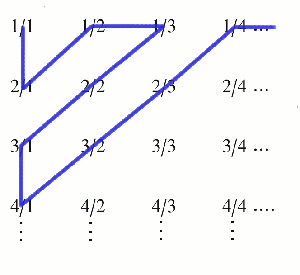
If we omit those rationals we have met before, this gives the list
![\[ 1, 2, \frac{1}{2}, \frac{1}{3}, 3, 4, \frac{3}{2}, \frac{2}{3}, \frac{1}{4},... \]](/MI/f9eec2549b798bd0852225fac652db35/images/img-0001.png) |
Now associate to the first fraction on the list the number 1, to the second fraction on the list the number 2, to the third fraction the number 3 and so on. This map is a bijection, proving that the set of positive rational numbers has the same cardinality as the set of natural numbers. This can then be extended to show that the set of all rational numbers has the same cardinality as the natural numbers.
Any infinite set which can be listed is called countable because (given an infinite amount of time) you could go down the list and simply count "one, two, three...". Because of this simple bijection with the natural numbers, all infinite countable sets have cardinality 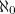 .
.
The second question Cantor considered in 1873 was the cardinality of the set of real numbers. The real numbers are all the points on the continuous number line, including irrational numbers such as 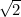 ,
, ![$\sqrt[7]{5}$](/MI/da85bed559c851336b19b618db4a17d6/images/img-0002.png) and
and 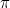 . The set of real numbers contains both the natural numbers and the rational numbers, but is it really "bigger" than both these sets? Cantor proved
that it is. To see this assume that the real numbers between zero and one are listable. Imagine such a list:
. The set of real numbers contains both the natural numbers and the rational numbers, but is it really "bigger" than both these sets? Cantor proved
that it is. To see this assume that the real numbers between zero and one are listable. Imagine such a list:
- 0.12
- 0.4
- 0.39878519436
- 0.777777...
- 0.141569...
Now change each digit of this new number, for example by adding 1. This gives the new number 0.21987... .This new number is not the same as the first number on the list, because their first decimal digits are different. Neither is it the same as the second number on the list, because their second decimal digits are different. Carrying on like this shows that the new number is different from every single number on the list, and so it cannot appear anywhere in the list.
But we started with the assumption that every real number between zero and one was on the list! Cantor proved that the only way to avoid this contradiction is to admit that our original assumption — that the set of real numbers and the set of natural numbers have the same cardinality — is false.

Leopold Kronecker: philosophy and theology, but no mathematics.
This famous diagonal argument shows that there are so many real numbers that they can't be listed, even with an infinitely long list, and so they cannot be counted, even in an infinitely long time. Because the real numbers are associated with the points on a continuous line, their cardinality is called  , the cardinality of the continuum.
, the cardinality of the continuum.
The diagonal argument shows that  represents a higher order of infinity than
represents a higher order of infinity than 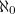 . Cantor adapted the method to show that there are an infinite series of infinities, each one astonishingly bigger than the one before.
. Cantor adapted the method to show that there are an infinite series of infinities, each one astonishingly bigger than the one before.
Today this amazing conclusion is honoured with the title Cantor's theorem, but in his own day most mathematicians did not understand it. Some vehemently criticised his work. His old professor, Leopold Kronecker said
Squared infinities
In January 1874 Cantor wondered if the points along a line of length 1 could be put into one-to-one correspondence with the points in a square of side length 1. Initially he thought that the answer was obviously "no", but he wanted a proof one way or the other. He was still working on this problem when he married Vally Guttmann in August 1874 — and spent most of his honeymoon discussing it with his friend and fellow mathematician Richard Dedekind.
Three years later Cantor finally realised that there is a bijection between the points on a line and those in a square. To see this, interweave the coordinates of each point in the square, to get a corresponding point on the line. For example to the point with co-ordinates (0.216, 0.750) associate the number 0.271560. It's not hard to show that this rule gives a bijection between the points in the square and the points on the line, forcing the counter-intuitive conclusion that the cardinality of the set of points on the square is the same as that of the points on the line.
It is easy to extend this argument to show that the points in a cube (or even in an n-dimensional hypercube) have the same cardinality as the points in a segment of the real line.
Cantor himself was so surprised by this discovery that he wrote:
Cantor's final struggles

David Hilbert: one of the mathematicians who recognised Cantor's genius.
From 1884 onwards, Cantor suffered from bouts of depression during which he could not concentrate on mathematics. He was disappointed that his ideas were not widely accepted, his relationship with Kronecker grew worse and his long friendship with Dedekind suffered. At his lowest points he was given leave from the University, but during his lucid periods he continued to have brilliant ideas
about the ordering of infinite sets. Cantor proved that there were a whole series of infinite cardinal numbers which could be listed in increasing order. The first of these is 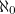 , the cardinality of the natural numbers, and the others are denoted by
, the cardinality of the natural numbers, and the others are denoted by 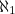 ,
,  ,
, 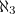 and so forth.
and so forth.
One question arising from this was which of these cardinal numbers was equal to  , the cardinality of the continuum. Cantor had shown that
, the cardinality of the continuum. Cantor had shown that  was greater than
was greater than 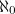 — but was it equal to the next cardinal number on the list,
— but was it equal to the next cardinal number on the list, 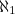 ? Cantor felt strongly that this should be the case. If not, then there was another infinity
that was "bigger" than that of the natural numbers but "smaller" than that of the real numbers. The assertion that
? Cantor felt strongly that this should be the case. If not, then there was another infinity
that was "bigger" than that of the natural numbers but "smaller" than that of the real numbers. The assertion that 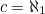 became known as the continuum hypothesis. Cantor spent the last years of his life struggling to find a proof one way or the other.
became known as the continuum hypothesis. Cantor spent the last years of his life struggling to find a proof one way or the other.
We now know that Cantor's mathematical toolkit was simply inadequate to answer the question. In 1940 (22 years after Cantor's death) Kurt Gödel showed that the continuum hypothesis cannot be disproved using standard mathematics, and after another 23 years Paul Cohen showed that neither can it be proved. Today mathematicians and logicians are still debating this fascinating question.
In 1899, after his youngest son and his younger brother died, Cantor's mental health and mathematical ability rapidly deteriorated. His last letters are to his wife Vally, written from a mental hospital, pleading to be allowed home. He died of a heart attack on the 6th of January 1918.
In 1904 The Royal Society of London honoured Cantor's life-long work laying the foundations of set theory and unravelling the mysteries of infinite sets by awarding him the Sylvester Medal, its highest award for mathematical research.
Further reading
You can find out more about Cantor's work in the Plus articles Infinite investigators: part I and Infinite investigators: part II.
About the author

Peter studied mathematics and physics at the University of St. Andrews, taught in Cyprus, studied at Manchester University, and gained a PhD from Strathclyde University for research in marine dynamics.
When not exploring remote corners of Scotland, or practising ShotoBudo karate, he teaches mathematics at James Watt College in Greenock.
He lives on the Firth of Clyde with his wife and a large (but finite) number of children. He is a believer in the goodness and grace of God, and a lay preacher of the Christian Gospel.
Plus would like to thank the London Mathematical Society and the Maths, Stats and Operational Research Network, as well as the journal Nature for their kind support of this competition.
 |
 |
Comments
Good summary
I just looked this up after listening to A Brief History Of Mathematics from the BBC.
Nice level of detail!
Cantor couldn't count
Let's take a simple look at the method of mapping counting numbers to rationals:
https://www.aplusclick.org/t.htm?q=7897
You can't do it with a finite limit N, and its gets WORSE as N gets bigger. Then suddenly, and magically, infinity makes it all work itself out. NO! With convergent series things getter better as N gets bigger. With divergent series things get worse. This is a divergent problem handled badly.
Another Cantor related subject is the Cantor set, generated by taking the number line between 0 and 1 and removing the open inner third part an infinite number of times. The question is then asked as to what, if anything, is left: http://lesliegreen.byethost3.com/articles/counting.pdf