Rabbit and string solution
Rabbit and string
A shaggy rabbit story
This is a story about rabbits. It doesn't have to be, it's just that I have a suitable photo and I've been told rabbits make excellent mathematicians (apparently they're very good at multiplying).
Buster is a very large rabbit. One day he is given a ball to play with. This ball happens to be the planet Earth - Buster is, after all, a very large rabbit. He's also given a piece of string which we could, if it didn't confuse matters greatly, call a cosmic string. Being a clever sort of rabbit, Buster decides to wrap the string around the ball and is pleased to discover that it fits neatly round the fattest part of the planet - let's call it the equator for convenience - with a tiny bit left over. This tiny extra bit is exactly 1 metre long.
Now, let's make some assumptions. Let's assume that Buster, being a very large rabbit, is also very cunning and can seamlessly join the two ends of the piece of string to make a large loop. Lets also assume that the planet Earth is not a slightly squashed ball with a bumpy surface but rather a perfectly smooth sphere - this is, after all, how it appears to big Buster.
Now, supposing Buster evens out the gap between the equator and his piece of string (which is, remember, only 1 metre longer than the equator - which is about 40,000 kilometres long), how big will the gap between them be?
Work quickly now or Buster may well have eaten the piece of string before you've finished!
The solution
As most readers realised, this is a "shaggy rabbit story" disguising a very simple fact - if you increase the circumference of a circle by a certain amount, you increase its radius by 0.16 units of length, regardless of the size of the circle you start out with.
This is easy to see: Suppose you start with a circle of circumference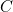 
and radius 
and then add 1 unit length to the circumference. Since 
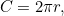we have
![\[ C + 1 = (2\pi r) + 1 = 2\pi (r + 1/2\pi ), \]](/MI/3dcac96e80a54d9ac0e5ab1e5f582aec/images/img-0004.png) |
, which is approximately 0.16. This figure does not depend on the value of .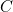
So the solution to the puzzle is that the string can be held out from the equator by about 16cm all along its length.
It seems surprising - at least to us here at Plus! - that a metre's slack gives you the same amount of clearance, whether you are wrapping string around the equator of the globe or just a basketball!
Back to main puzzle page