There's a beautiful connection between sunflowers and maths. When you look at the edge of a sunflower's seed head, you can usually spot two families of spirals, one running clockwise and the other anticlockwise. When you count how many spirals there are in each family, you'll most probably get two consecutive numbers from the famous Fibonacci sequence. The sequence starts with a 1 followed by a 2, and all other terms are made by adding the two preceding ones, giving:
1, 2, 3, 5, 8, 13, 21, 34, 55, 89, ...

At the edge of this picture of a sunflower, if you count those curves of seeds spiralling to the left as you go outwards, there are 55 spirals. At the same point there are 34 spirals of seeds spiralling to the right. The numbers 34 and 55 are consecutive numbers of the Fibonacci sequence. Image: R. Knott from our article about the Fibonacci numbers. Knott also has a beautiful website exploring this topic.
There are also spiral families appearing further towards the centre of the seed head, going in both directions, and counting them will probably reveal a smaller pair of the Fibonacci sequence.
These number patterns appear magical at first, but they are there for a reason. People have suggested that the Fibonacci pattern has evolved to ensure that as many seeds as possible are fitted into the seed head as the plant grows (see this article for more detail). There are mathematical models that simulate the growing process. If you run them on a computer you'll get an idealised version of the pattern you see in the sunflowers.
But do sunflowers really always stick to those stark mathematical rules when they are growing, or is there more to it? If you want to answer this questions you first need to look at lots and lots of sunflowers and that's a lot of effort. This is why Jonathan Swinton and Erinma Ochu have enlisted the power of citizen science. To celebrate the centenary of the famous mathematician Alan Turing (who gave some thought to sunflowers and other plants exhibiting the Fibonacci sequence) Manchester's Museum of Science and Industry launched a project called Turing's Sunflowers in 2012. It invited people to grow their own sunflowers and count the spirals. The project engaged people with science and maths, and it also resulted in the largest sunflower spiral study ever.

A very happy-looking sunflower.
Swinton and Ochu obviously weren't able to look at all the sunflowers that were grown in person, so instead they analysed their photographs. The Fibonacci sequence clearly dominated the results. When simply counting the number of spirals in a single family on a seed head (disregarding the partner family that goes in the other direction) the researchers found that in 74% of the 768 families counted, the number of spirals was a number from the Fibonacci sequence. When they looked at pairs of spirals on a seed head, one going clockwise and one going anticlockwise, they found that 66.6% of these were a pair of numbers from the Fibonacci sequence.
But that wasn't all. The traditional Fibonacci sequence starts with a 1 and a 2, which means the following numbers are 1+2 = 3, 2+3=5, and so on. But you could also start with a 1 and a 3, getting the Lucas sequence
1, 3, 4, 7, 11, 18, 29, 47, 76, 123,....
Or you could start with a 1 and a 4, getting the sequence known as F4
1, 4, 5, 9, 14, 23, 37, 60, 97, .....If you double all the numbers in the ordinary Fibonacci sequence you get
2, 4, 6, 10, 16, 26, 42, 68, 110, ...
which also follows the rules that every number is the sum of the preceding two.
The researchers found that another 67 of the individual spiral families (that's around 9%) had a number of spirals coming from one of these generalised Fibonacci sequences, and they found that another 7% of spiral pairs were pairs of numbers from one of these generalised sequences. This is still in line with the mathematical models, which don't say that the pattern evolving on a growing plant has to exhibit the Fibonacci sequence: a generalised one is fine too.

In this example there are 68 clockwise spirals and 42 anticlockwise ones. The numbers 42 and 68 are consecutive numbers in the double Fibonacci sequence. Image © 2016 Jonathan Swinton and Erinma Ochu. Published by the Royal Society under the terms of the Creative Commons Attribution License.
But if those models are correct, then what about the sunflowers in the study that did not match the Fibonacci pattern? As you'd expect, quite a few of those are nearly Fibonacci — after all, nature is rarely exact. Interestingly, numbers of the form Fibonacci - 1 featured significantly more often than number of the form Fibonacci + 1. That's not something you'd expect if the deviations were just random, so something worth explaining might be going on there.
There were also samples, however, whose beautifully ordered spirals did not follow a Fibonacci pattern. An example is sunflower 502:
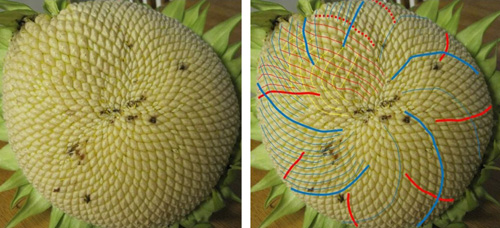
While the relatively unambiguous anticlockwise 56 count might be interpreted as close to the Fibonacci number 55, the equally unambiguous clockwise 77 count is far from Fibonacci. Image © 2016 Jonathan Swinton and Erinma Ochu. Published by the Royal Society under the terms of the Creative Commons Attribution License.
And other samples were too disordered to give a clear count. Sunflower 667 has a clear family of spirals running clockwise (in blue):

For this sunflower it's easy to count the clockwise spirals (there are a total of 50), but it's hard to count the anticlockwise ones. Image © 2016 Jonathan Swinton and Erinma Ochu. Published by the Royal Society under the terms of the Creative Commons Attribution License.
But when you try and trace the anti-clockwise spirals you end up with a mess of competing families. In such cases, whether you come out with something Fibonacci-like or not may depend on your personal choice of how to count spirals.
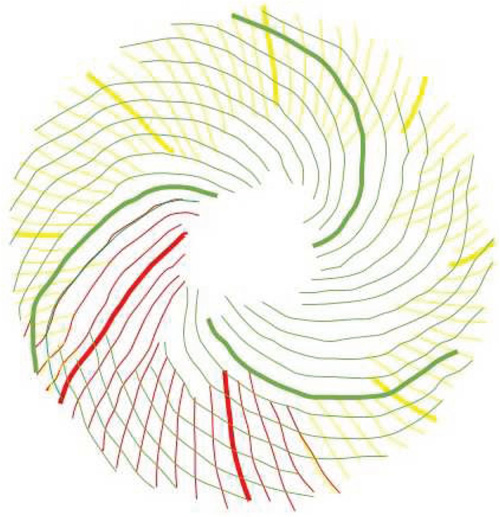
This image shows competing anticlockwise families. Image © 2016 Jonathan Swinton and Erinma Ochu. Published by the Royal Society under the terms of the Creative Commons Attribution License.
Some of those non-Fibonacci phenomena might be dealt with by using different rules for counting the spirals, and others might be easily explained by existing mathematical models. But taken together, the researchers believe, the observations pose a challenge for further research. One step towards better understanding the Fibonacci phenomenon, which appears not only in sunflowers, but in other plants too, might be to construct mathematical models that, rather than following an exact path, can represent the "noisiness" of the developmental process. But whatever the outcome of future research, a large part of the credit will go to the volunteers who grew all those sunflowers in their homes.
You can find a detailed breakdown of the sunflower results in Swinton and Ochu's paper Novel Fibonacci and non-Fibonacci structure in the sunflower: results of a citizen science experiment, which has been published in Royal Society Open Science.
Comments
Sunflower spirals
Consider once again sunflower 502. The author says its anticlockwise spiral count of 56 "might be interpreted as close to the Fibonacci number 55", in contrast to the clockwise 77 count which is "far from Fibonacci". Nevertheless these two numbers lead to a couple of intriguing sequences. Subtract 56 from 77 and you get the Fibonacci 21. Then subtract 21 from 56 and we get the "close" 35. However these last two numbers, 21 and 35, aren't in the traditional Fibonacci order. Let's swap them round so they are, and get the sequence 0 7 7 14 21 35 56 91 . . . which are of course the ordinary Fibonacci numbers multiplied by 7. Maybe the sunflower's "deviation" was initially one of order leading to a deviation in quantity.
Thanks Chris G
Hi Chris G, thanks for taking the time to read about our work (I'm one of the authors of the original paper). I'm pleased that you found the sequences you derive intriguing, but it's important to remember that it is very easy to find apparently meaningful sequences from pairs of integers once you allow yourself a few "close"s and "swap"s and "multiplied by"s. If you haven't done so already you might find reading our paper and some of the references it cites useful in articulating your ideas in ways that help guard against this pitfall.
Swaps and multiples
OK, let's run my argument again from a different starting point - by considering a septuple Fibonacci, bearing in mind that examples of the double Fibonacci have already been observed. This would normally go: 0 7 7 14 21 35 56 91 147 . . . This means we don't have to allow ourselves a "close" since 56 is already perfectly correct here. I then allow myself just one other tweak, that is swapping round the 21 and 35, so that as long as we stick with the same recurrence relation, at least in a rightwards direction, the sequence now goes . . . 35 21 56 77 . . . The next term would be 133, though admittedly it doesn't look like sunflower heads ever run to this quantity of spiral arms.
The other two Fibonacci generalisations considered in the article, Lucas and F4, can also be regarded as the result of swapping round two terms in the original Fibonacci sequence and adding them together. In Lucas those are the 2 and the 1, in F4 they're the 3 and the 1, going 3 1 4 5 9 etc. Why not swap round terms further along?
I'm also very interested in those Fibonacci generalisations with different recurrences, such as Narayana's Cows (OEIS A000930), and wonder if they too crop up in natural structures and processes such as sunflower seed heads. Projects such as yours are obviously very important for this, and I will study your paper closely.
Spirals
Dear Marianne I have never knowingly taken hallucenogenic drugs but in Mexico a Shaman gave me.PTSD by showing me.spirals as he said it was another dimension could it be that there is a scientific explanation for what he did?
Deep learning
It seems this problem of sunflower irregularity could be a good application of deep learning. Has anyone talked to any grad sudents in AI to see if someone wants to do a dissertation on sunflower fibonacci irregularites? They could develop a neural network and train on sunflower pictures and be able to instantly identify regular, semi-regular and irregular spiral patterns. I think with a little work the system could produce the above red and yellow diagrams showing what parts of flowers were regular and irregular. Its fanscinating that nature is so irregular but until this study I had never heard that sunflower patterns were irregular. Humans are so simplistic and love to think nature is like clockwork. Reminds me of Wolframs's 'new science' work. Excellent work of the sunflowers everyone. Thank you.
SITE OWNER - I literally had to do 20 captchas to post this. Maybe relax your requirements on that facism. I doubt the math-bots are that troublesome.