A proof with a hole 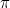solution

Share this page
Submitted by plusadmin on 1 September, 2006
September 2006

A proof with a hole: $\pi $ equals 2

Forget all that business about 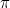$\pi $ being an irrational number with infinitely many decimal places: I can prove conclusively that 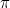$\pi $ is equal to 2.

First of all, let's recall that 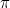$\pi $ is defined to be the ratio between the circumference and the diameter of a circle, which is the same regardless of the size of the circle. So, using the notation of the diagram below, we have 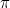$\pi $ = c/d.

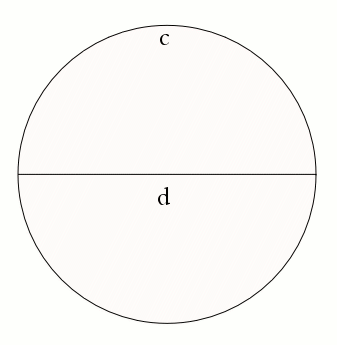
Now let's start with a circle of circumference 2, and only consider one half of it, as shown in the figure. Since it's exactly one half of the circle, the length of this semi-circle is 1. Now let's divide in half the diameter d of the circle, and draw a new, smaller semi-circle on each of the two halves. Since the ratio between diameter and circumference is the same for any circle, you can work out that the two smaller semi-circles — which are built on half the diameter of the larger one — have circumference half that of the larger one. In other words, the length of each of the two smaller semi-circles is 1/2.

Now continue in the same manner: divide the original diameter d into 4 equal pieces and draw on each of them a semi-circle of length 1/4; then divide it into 8 equal pieces and draw on each of them a semi-circle of length 1/8, etc, etc. After n steps you have 2n semi-circles, each of length 1/2n.

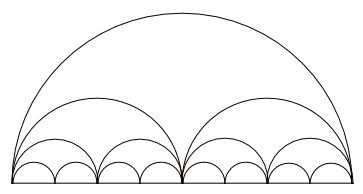
Obviously, the semi-circles get smaller and smaller at each stage, and after a great number of steps, your string of semi-circles will hardly be distinguishable from the straight line which forms the diameter of the largest circle. The string of semi-circles approximates the diameter d, and the approximation gets better and better the more steps you take. This means that the lengths of the semi-circles all added up approximate d. In fact, d is the limit of this sum as the number of steps n tends to infinity:

d = limn→∞ 2n×1/2n = 1.
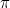We know that the circumference c of the large circle is 2, so $\pi $ = c/d = 2/1 = 2, which proves my claim. Or have I made a mistake?

The solution

What's wrong here? Well, it is definitely true that after n steps there will be 2n semi-circles, each of length 1/2n. It is also true that the diameter of the largest circle is in some sense a limit of the strings of semi-circles: by making n large enough, you can ensure that the nth string of semi-circles squeezes as closely to the diameter d as you like.

The mistake lies in the next step: the assumption that the lengths of the strings of semi-circles tend to the length of the diameter. This is false! What happens in fact is that while at each stage we replace the semi-circles involved by smaller ones, these also become more numerous, so that the replacement makes no difference at all to the overall length. Every string of semi-circles has length 1, since

2n×1/2n = 1,

for any n. We've simply replaced a number of big wriggles by a greater number of smaller wriggles without changing the length.

The lesson is, when it comes to things like length, don't be fooled by appearance. A curve may look like a straight line, but it may be so wriggly at a small scale that in fact it's a lot longer than the line. Even worse, a set may look like a decent curve that should have finite length, but may in fact be infinitely long. And what exactly do we mean by "length" anyway? For an example of a curve with infinite length, check out the Koch curve in Plus article Jackon's fractals. And to learn about notions of length read Plus article Measure for measure.


Back to main puzzle page