
Mathematical mysteries: The gentlemen from Basle and the Petersburg Paradox
The difficult Bernoulli family

Daniel Bernoulli
Just over 220 years have passed since the death of one of the most distinguished mathematicians in history: Daniel Bernoulli, who died on March 17th, 1782. The name of Bernoulli asks for precision since the family from Basle produced no fewer than eight outstanding mathematicians within three generations. As the same Christian names kept being used in the family, there is a numbering system used to tell fathers, brothers, sons and cousins apart. It starts with Jakob I and his brother Johann I (the third brother, Nikolaus, being an artist who wasn't given a number). Then there are, in the next generation, Nikolaus I and Johann's three sons Nikolaus II, Daniel and Johann II. Finally, the two sons of Johann II, called Johann III and Jakob II, followed in the footsteps of their brilliant ancestors. (Johann's third son, Daniel, didn't get any further than being deputy professor at the University of Basle. Hence he wasn't given a number, which is why his famous uncle and namesake didn't need one either.)
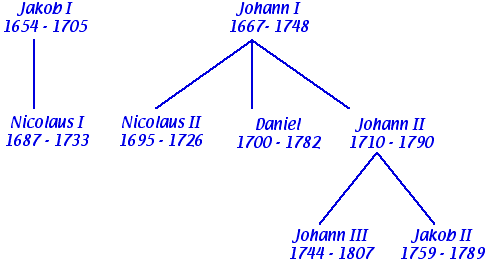
The mathematicians of the Bernoulli family
Together with Isaac Newton, Gottfried Wilhelm Leibniz, Leonhard Euler and Joseph-Louis Lagrange, the Bernoulli family dominated mathematics and physics in the 17th and 18th century. The family members were interested in differential calculus, geometry, mechanics, ballistics, thermodynamics, hydrodynamics, optics, elasticity, magnetism, astronomy and probability theory. For more than thirty years the National Funds of Switzerland has been supporting work on an edition of the complete works by Jakob I, Johann I and Daniel. The complete edition will comprise 24 volumes. A further 15 volumes including a selection out of their 8,000 letters is to follow.
Brotherly hate

Jakob I
Unfortunately, the gentlemen from Basle were as conceited and arrogant as they were brilliant. This resulted in rivalry, jealousy, rows and reproaches made in public. Yet everything had started idyllically. Jakob I, who had acquired his knowledge in the natural sciences by himself and went on to teach experimental physics at the University of Basle, secretly introduced his younger brother to the mysteries of mathematics. This was very much against the will of their parents, who wanted the younger brother to embark on a career in commerce after the elder son had refused to enter the clerical career they had planned for him.
But the harmony between the two highly gifted brothers soon turned into bitter arguments. The conflict began when Jakob, annoyed by Johann's bragging, claimed in public that the works of his former student were but copies of his own results. Next Jakob I - who by then held the chair of mathematics at the University of Basle - plotted successfully against his brother's appointment to his department. So Johann I had to teach at the University of Groningen before finally being offered a chair in Basel - the chair of Ancient Greek. But fate decided otherwise, and just when Johann set off for his native town, Jakob died. Thus his (not too grief-stricken) brother was, after all, offered the chair of mathematics in Basle. Jakob's most important opus, his Ars Conjectandi (The Art of Conjecture) which appeared after his death, formed the basis of probability theory.
But don't believe that Johann I learnt anything from this sad story. In educating his own sons he committed exactly the same mistakes as his father had done before him. Claiming that mathematics couldn't provide a living, Johann tried to bully the most gifted of his three sons, Daniel, into a career in commerce. When this attempt proved unsuccessful, he allowed him to study medicine - in order to prevent his son from becoming a competitor. But the sons followed the example of their ancestors, and Daniel, while studying medicine, took lessons in mathematics from his older brother Nikolaus II. In 1720 he travelled to Venice in order to work as a physician. However, his heart still belonged to physics and mathematics, and during his stay he acquired such a great reputation in these field that Peter the Great offered him a chair at the Academy of Science in St. Petersburg.
Fulfilment in the Russian capital

Daniel's companion Leonhard Euler
In 1725 Daniel travelled together with his brother Nikolaus II, who was also offered a professorship for mathematics at the Academy, to the capital of the Russian empire. Their joint sojourn didn't last for long. Hardly eight months after their arrival Nikolaus II fell ill with a fever and died. Daniel, who possessed more sense of family than his father, was very distressed and wanted to return to Basle. But Johann I didn't want to have his son back home. Instead he sent one of his pupils to St. Petersburg to keep Daniel company. This was an extremely lucky coincidence, since the pupil was none other than Leonhard Euler, the only contemporary who could compete with the Bernoullis when it came to mathematical talent. A close friendship developed between the two Swiss mathematicians in exile. The six years they spent together in St. Petersburg were the most productive time of Daniel's life.
After Daniel had returned to Basle, the quarrels within the family started anew when he won the prize of the Parisian Academy of Science with a paper on astronomy - jointly with his father. Johann I didn't act like a proud father. On the contrary, he kicked his son out of the house! (Daniel won the great prize of the Academy nine times.) And there was worse to come: In 1738 Daniel published his magnum opus, Hydrodynamica. Johann I read the book, hurriedly wrote one of his own with the title Hydraulica, dated it back to 1732 and claimed to be the inventor of fluid dynamics. The plagiarism was soon uncovered, and Johann was ridiculed by his colleagues. His son never recovered from the blow.
A pound isn't always worth a pound
In 1713 Daniel's cousin Nikolaus posed a question about the following game:
- Toss a coin.
- If it shows heads you get £2. Otherwise, if it shows tails, you toss again.
- If the coin now shows heads you get £4, and so on. Whenever you toss tails the prize is doubled.
- After n tosses the player gets £2n if heads appear for the first time.

How much would you pay? [Image freeimages.co.uk]
After 30 tosses this is a sum of more than one billion pounds - a gigantic prize. Now the question: how much would a gambler pay for the right to play this game? Most people would offer between £5 and £20 - but is this fair? On the one hand, the chance of winning more than £4 is just 25%. On the other hand, the prize could possibly be enormous, because the probability of tossing a very long series of tails before that first toss of heads, although very small, is by no means zero. The huge prize that could be won in this case compensates for the very small probability of success - Nikolaus Bernoulli found that the expected prize is infinite! (The expected prize is calculated by multiplying all the possible prizes by the probability that they are obtained and adding the resulting numbers.)
And therein lies the paradox: If the expected prize is infinite, why is noone willing to pay £100,000, £10,000 or even £1,000 to play the game?
The explanation of this mysterious behaviour touches the areas of statistics, pyschology and economics. Daniel Bernoulli and another Swiss mathematician, Gabriel Cremer, suggested a solution. In a scientific article they postulated that £1 isn't always of the same use to its owner. A beggar can make much better use of £1 than a millionaire. Whereas owning an additional pound could mean for the former that he does not have to go to bed hungry, the latter would hardly notice the increase of his fortune by £1. In a similar manner, the second billion one could win when the 31st toss shows tails again wouldn't be of the same use as the first billion. The utility of two billion pounds just isn't twice the utility of one billion pounds.
And this explains the mystery. The important quantity is the expected utility of the game (the utility of the prize multiplied by its probability) which is far less than the expected prize. Daniel Bernoulli's treatise was published in the Commentaries of the Imperial Academy of Science of St. Petersburg and this surprising insight was henceforth called the St. Petersburg paradox.
The roots of the insurance business
Around 1940, the idea of the utility function was taken up by two immigrants from Europe working at the Institute of Advanced Studies in Princeton. John von Neumann (1903-1957), one of the outstanding mathematicians of the 20th century, was Jewish and had been forced to flee his native Hungary when the Nazis invaded the country. The economist Oskar Morgenstern (1902-1976) had left Austria because he loathed the National Socialists.
In Princeton the two immigrants worked together on what they thought would be a short paper on the theory of games. But the treatise kept growing until it finally appeared in 1944 as an opus of 600 pages, under the title Theory of Games and Economic Behavior. This pioneering work was to have a profound influence on the further development of economics. In the book, the utility function of Bernoulli and Cramer was used as the axiom of the behaviour of the proverbial "economic man". However, it was soon noticed that test candidates often made a decision that contravened the postulated axioms when they found themselves in a situation where the probability was very low, but the amount of money was very high. The economists didn't let themselves be swayed by this. They insisted that the theory was right and that many people simply acted irrationally.

Money down the drain [Image DHD photo gallery]
Nevertheless, utility theory has had one far-reaching consequence. The explanation offered by Bernoulli and Cramer to account for the St. Petersburg paradox formed the theoretical basis of the insurance business. The existence of a utility function means that most people prefer having £98 cash to gambling in a lottery where they could win £70 or £130 each with a chance of 50% - although the lottery has the higher expected prize of £100. The difference of £2 is the premium most of us would be willing to pay for insurance. That many people pay for insurance to avoid any risk, yet at the same time spend money on lottery tickets in order to take a risk of a different kind, is another paradox, which is still waiting to be explained.
About this article
This article first appeared in German, in the form of two short articles entitled "Daniel Bernoulli and his difficult family" and "A pound isn't always worth a pound - how the St. Petersburg paradox became the basis of insurance business", in the newspaper "NZZ am Sonntag", on March 17th and June 2nd 2002, respectively. They are reprinted here by kind permission of the author and copyright holder, George Szpiro (g.szpiro@nzz.ch), and NZZ am Sonntag.
The articles were translated for Plus by Peter and Kirsten Mörters.
Comments
Anonymous
I enjoyed reading your article, thanks.
Anonymous
The article is amazing.even for those not interested in maths ,its a great read.