
Outer space: A matter of gravity

Isaac Newton
When Isaac Newton reflected upon his law of gravitation — the famous "inverse square law" which states that the force of gravitational attraction between two masses whose centres are separated by a distance r is inversely proportional to r2 — he realised there was a big gap in his arguments. Filling that gap delayed the publication of his momentous Principia until 1687. He had been assuming that the gravitational force exerted by a sphere is exactly the same as that exerted by an idealised point of the same mass located at its centre — let's call it the spherical property. He had assumed that this was always true, but maybe it wasn't. It certainly made life easier to assume it to be true. Planets could be thought of as mere points feeling the gravitational pull of a point-like Sun as they traced out their oval orbits in his notebook, just as they did around the solar system.
Eventually, in the first part of the Principia, Newton was able to show that his assumption had been a good one, but along the way he discovered some other things that are at least as surprising. If the law of gravitational force between two masses separated by a distance r is proportional to rn then the only values of n for which the spherical property holds are n = -2, which gives the inverse square law, and n = +1, giving the so-called "harmonic law". The other possible laws with different values of n wouldn't allow us to replace a sphere by just a single point of any mass and get the same results. Newton was no doubt relieved to discover that his intuition had been right all along.
Pierre-Simon Laplace
Newton studied the n = +1 law because it was easy to solve but he discarded it as being of no scientific interest for the study of gravity because it required forces between masses to increase as their separation grew in space. He didn't know of any natural forces that behaved like that. Gravity certainly didn't. Nearly 125 years later, Pierre Laplace went a step further and showed
(what Newton likely knew) that the most general possible force law that has the spherical property is just the sum of any n = -2 law and any n = +1 law. So a mass m is accelerated by another mass M by a gravitational force equal to
where G is the Newtonian gravitational constant. Here, I have equipped one of the constants of proportionality, -GMm which gives Newton's law of gravity, with a minus sign because gravity is attractive. The other, λ, will be repulsive if λ is positive, and so it appears with a plus sign. The second term (λr) is strange and, like Newton before him, Laplace ignored it in matters of gravity.
The λ term remained forgotten and useless until 1915, reappearing in an unexpected way only when Einstein created the general theory of relativity — his remarkable new theory of gravity which superseded Newton's seventeenth-century masterpiece. Yet, although Einstein's theory superseded Newton's, it didn't make it obsolete. When gravitational fields are weak and motions occur at speeds much less than that of light, Einstein's theory just becomes more and more like Newton's theory. Newton's gravity is a limiting case of Einstein's gravity and holds with very high accuracy on Earth today. This is why engineers build bridges using Newton's theory rather than Einstein's. If students are studying gravity in 1000 years time, they will still be studying Newton's theory and using it in the same way.

Albert Einstein
Einstein's theory had an unusual feature that even now, many textbooks present as a oddity that had no motivation in Newton's description of the world. But our story so far allows us to see where it comes from. Einstein's theory allowed gravity to have two parts. One of them was a more complicated version of Newton's inverse square law, which it became when gravity was weak; the other was just the repulsive λr term that Newton had first identified. So, the overall force law when gravity was weak was just as Laplace had found: the one and only force law with the spherical property.
Einstein was very interested in λ at first, and called it "the cosmical constant", because he thought he could use it to avoid predicting that the Universe is expanding (actually Einstein's original "cosmical" or "cosmological" constant was denoted by a capital lambda, Λ, and is equal to 3λ/c2 where c is the velocity of light). He forced his mathematical universe to be a static one by setting λr equal to GM/r2 so the net gravitational force in the Universe is zero and the radius, r, of the universe is constant.

The supernova 1994D in galaxy NGC 4526 (the supernova is the bright spot on the lower left). As the name suggests, this supernova was first observed in 1994. This image was taken by the Hubble Space Telescope and appears here courtesy of NASA.
Einstein gave up the λr term and never used it again after the early 1920s. Astronomers searched for its accelerating effects on the expansion of the universe for 70 years and found none. The reason they didn't give up searching was that more powerful telescopes might find galaxies accelerating away from us if they looked at fainter images from more distant parts of the Universe. As r gets bigger and we look at more and more distant stars and galaxies we might eventually reach a distance where a λr repulsion exceeds the falling force of attraction -GM/r2. The observational limits on λ became so strong — λ could not be bigger than about 10-34 — that, generally, cosmologists assumed that λ was equal to zero for some deep unknown reason that we would eventually discover.
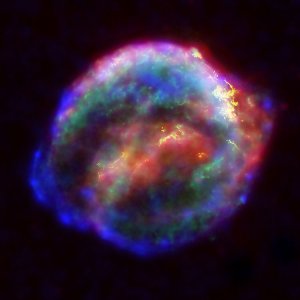
The remnant of the supernova 1604, also known as Kepler's Supernova, as it was first observed by Johannes Kepler in 1604. This supernova is in our own galaxy, only about 20,000 light years from Earth. This is a composite image constructed from images of the Spitzer Space Telescope, the Hubble Space Telescope, and the Chandra X-ray Observatory. Image courtesy of NASA.
Today, astronomers are faced with the puzzle of an accelerating universe driven by a repulsive λr force of gravity on very large scales in the Universe, but over the smaller dimensions of galaxies and galaxy clusters the attractive inverse square force of Newton holds sway and binds them together. What is this mysterious λ? Particle physicists believe that it is the quantum vacuum stress of the Universe but have no idea why it has the tiny numerical value of about 10-34 that is observed — a value that ensured that it took control of the expansion of the universe, changing it from deceleration to acceleration, when it was about three-quarters of its present extent. If it was just ten times bigger, still a tiny 10-33, then everything would have been different. Acceleration would have begun too early in the Universe's history and there would have been no galaxies, no stars, no us — and no Plus.
Did you manage to answer the puzzle posed in Outer space: a collector's piece? If not, you can find the answer here!
Comments
Anonymous
Wow I learned this in science last year with my teacher! it is so amazing on how Mr.Newton was this smart! im vry glad we have people like this and teachers to teach us this =)