At last month's Cambridge Science Festival we decided to try and bring maths to the masses using one of our favourite puzzles. Our aim was to deliver a hands-on (or rather feet-on) activity that's fun and brings across the creative aspects of maths, but also links up to cutting edge mathematical research. We were really pleased with how it went, so we thought we'd share our game for others to put on at their own science or maths event.

A young mathematician in action. (Photo by Alice Boagey, copyright University of Cambridge)

The game is based on a puzzle known as the Bridges of Königsberg which, unsurprisingly, originated in the Prussian city of Königsberg (now Kaliningrad) in the 18th century. Königsberg was divided by a river containing two islands, with the various land masses connected by seven bridges (see the image below). The task the people of Königsberg posed each other was to find a walk through the city which crossed each bridge once and only once.

At the science festival we recreated the lay-out of land, river and bridges using coloured yoga mats you could walk around on and challenged people to find the path the Königsberg people had been after. The challenge (and the colourful yoga mats) proved irresistible to many passing punters, especially the younger ones. The task is easy to explain and it seems easy to solve — as people watched person after person try but fail to cross all seven bridges, many decided to have a go themselves. You could see their brains ticking over as they were searching for that clever lateral thought that would give the solution.

The perhaps slightly cruel thing is that a solution does not actually exist! There is no path that crosses every bridge exactly once. But thankfully, the beautifully simple explanation of why the puzzle is impossible to solve quickly makes up for the shock of the announcement. Any piece of land, unless it's the one you start or finish your walk on, needs to have an even number of bridges attached to it. That's because whenever you enter the piece of land via one bridge you need to leave it by another, so you need two bridges for every visit. Therefore, if more than two pieces of land have an odd number of bridges attached to them, the puzzle is impossible to solve (the two pieces that are allowed to have an odd number of bridges would have to contain the starting or finishing points of the path). And as you can easily establish with help from the youngest audience members, in the bridges of Königsberg problem, all pieces of land have an odd number of bridges attached to them — so the puzzle is impossible to solve.

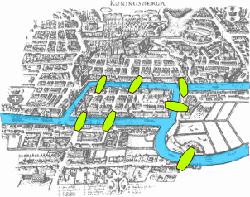
The seven bridges of Königsberg. Image: Bogdan Giuşcă.

This elegant argument, so simple yet so clever, inspired real eureka moments and did a great job at bringing across the beauty and power of creative mathematical thought. You can illustrate it further by "blowing up" a bridge to make the problem possible. We asked people which bridge they'd like to have blown up and took that one away. With the correct number of land pieces now having an even number of bridges attached to them, the problem becomes not only possible but also easy, so even the youngest audience member can have a go.

When the great mathematician Leonhard Euler first came up with this argument in 1735 he laid the foundation for a field of mathematics known as graph theory. A graph is a collection of nodes (in our case pieces of land) connected together by links (in our case bridges). It's really just a network, and since networks permeate modern life, from road or rail networks to social networks and the internet, Euler's ingenious solution laid the foundation for an area of maths that couldn't be more relevant today.

The game is really easy to put on, here is how we did it.

• Use 6 yoga mats of two different colours: we used 4 green mats for the land masses and 2 purple mats chopped up for the bridges. (You can buy yoga mats quite cheaply online). Cut the mats into pieces: four pieces to represent the landmasses and seven for the bridges. The land pieces don't have to have the same shape as the ones shown in the map of Königsberg above: the important thing is not their shape but how they are connected by the bridges.
• Tape the land pieces to the floor and draw or tape a cross on one side of each bridge piece. Lay the pieces out (with the crossed side facing down) to re-create the Königsberg problem and wait for people to get curious.
• As people try and find a path through this mini-maze, turn over the bridges they have used so that the cross shows, indicating they can't cross that bridge again.
• After a few people had a go tell your audience the secret (it's impossible) and explain why. Ask people to nominate a bridge they want blown up, take the corresponding mat away, and let people find the now possible path.
• If appropriate give a brief talk explaining the relevance of graph theory to modern life (see this article for some information.
• Have fun!

This activity was inspired by content on our sister site Wild Maths, which encourages students to explore maths beyond the classroom and designed to nurture mathematical creativity. The site is aimed at 7 to 16 year-olds, but open to all. It provides games, investigations, stories and spaces to explore, where discoveries are to be made. Some have starting points, some a big question and others offer you a free space to investigate.