December 2009
Trisecting the angle using origami — proof
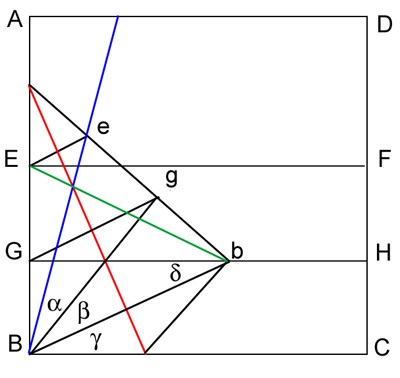
In the image on the right the angle between the blue line and the bottom edge is the angle to be trisected, and we must show that .
The red line is the crease resulting from the fold in step 4 of the folding sequence. Now look at the triangle . We know that the length of the line segment is equal to the length of the line segment , and we also know
that the line segment , which is the height of the triangle , meets the line segment at a right angle. In other words, the height of the triangle divides the opposite side into half. This means that the triangle is isosceles.
The mirror image of the triangle when reflected in the red crease line is the triangle , which is therefore also isosceles.
The height of
the triangle extending from the point therefore divides the angle at into half. This shows that . By mirror symmetry we have that is equal to the angle of the triangle . And since the line is parallel to the bottom edge , we have that . This proves that .
Return to main article