Twelve's Company, Thirteen's a Crowd

In 1694, a famous discussion between two of the leading scientists of the day - Isaac Newton and David Gregory - took place on the campus of Cambridge University. Their dispute concerned the "kissing problem." But don't get your hopes up. The term kissing in this context has nothing to do with the gesture of affection: here the verb kiss refers to the game of billiards, where it signifies two balls that just touch each other.

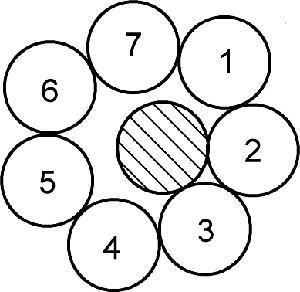
Heptagon: No touching and no kissing

Newton and Gregory argued about the number of spheres of the same radius that could be brought into contact with a central ball. On a straight line two balls can kiss a ball in the centre, one on the left and one on the right. On a billiard table, at most six balls can touch a central ball. There's no room for a seventh, as anyone can verify by rolling the balls around a bit. The reason for this is that a heptagon (seven-cornered polygon) whose sides have length 2 (i.e., two balls) is too large to fit tightly around a circle with radius 1. So far everything is clear. But let's move off the green felt and up into the realms of space.

In the 1950s, H. W. Turnbull, an English school inspector, was doing research on the life of Isaac Newton. Working his way through the numerous papers, letters, and notes, he came across two documents that would provide the basis for the kissing problem: a memorandum of a discussion that the two scientists had at Cambridge, and an unpublished notebook at Christ Church at Oxford in which Gregory had jotted down some notes. The two men had been discussing the distribution of stars of various magnitudes that revolve around a central sun. In the course of their deliberations the question arose whether a sphere can be brought into contact with 13 others of the same size. And that's where opinions diverged.

How many white billiard balls can kiss a black billiard ball in three-dimensional space? Kepler stated in his Six-cornered Snow that twelve spheres could touch a central sphere, and then went on to describe two possible ways to arrange the balls. But maybe 13 spheres can be brought into contact with a central sphere? Initially we may think this clearly impossible, since Kepler's arrangement is completely rigid. All the balls touch the central ball and they also touch each other. So how could a further ball be squeezed in between? The question is not quite trivial because the hexagonal packing - three balls below the central sphere, six around it, and three above - is not the only arrangement of "12 around 1". We already saw that the cubic packing - four balls below the central sphere, four on each side, and four above - is another display of "12 around 1". As Kepler pointed out, however, these two arrangements are identical, and any apparent differences are merely the result of looking at the balls from different perspectives. But maybe there is a truly different arrangement that brings a dozen white balls into contact with the black ball?

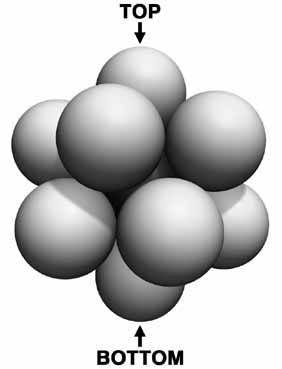
The icosahedral arrangement

There is - and not just one. Put one sphere on the bottom, then arrange five balls in a pentagon around the central ball, just below its equator, place another five balls more or less in the interstices of the lower five balls (this puts them slightly above the equator of the central ball), and finally top it off with the twelfth sphere on the pinnacle. So there you have it: another arrangement of a dozen spheres around the central ball. You may note that the spheres sit more or less on the vertices of an icosahedron, which is why this configuration is called the icosahedral arrangement.

If we now take a close look, a very surprising fact emerges: this arrangement is not rigid. Sufficient space is left over in the interstices between the twelve balls, that they can roll around a bit on the surface of the central ball. You may ask yourself, as did Gregory, can the balls be moved in such a way that sufficient space opens up for an additional sphere? Maybe the free spaces can be combined so that a thirteenth ball can be squeezed in? This may seem absurd, but Gregory did have a point and we will give a mathematical demonstration that "13 around 1" is at least conceivable.

Superball

Consider a number - as yet unknown - of spheres that kiss a central sphere, all of radius 1. Deposit the whole arrangement into a super-ball with a radius of 3. Imagine a lamp at the centre of the central ball that casts shadows of the surrounding balls onto the inside surface of the super-ball. These circular shadows cannot overlap. It is shown in the Appendix that each shadow has a surface area of 7.6; the total surface of the super-ball is 113.1. So how many shadows can fit onto the super-ball's surface? Divide 113.1 by 7.6 and you get 14.9. The inescapable conclusion is that there is room for nearly 15 balls! Definitely there is sufficient surface, at least theoretically, for 14 balls, and so 13 balls certainly should be considered a possibility.

Isaac Newton

Isaac Newon

Let us get back to the dramatis personae. Isaac Newton was one of the foremost scientists of all times. He was born on January 4, 1643, in Woolsthorpe, in Lincolnshire, a tiny, weak baby that was not expected to survive for even a week. However, Isaac proved doctors and midwives wrong and extended his lifetime to another 84 years. He never knew his father, an uneducated, illiterate man who died three months before his birth. After the death of her husband, Isaac's distraught mother Hannah, née Ayscough, was badly in need of the church's compassion and the minister of the nearby village was only too happy to oblige. The reverend took his comforting duties very seriously and after a proper period of mourning the two got married. With this, little Isaac became superfluous and was shipped off to his grandparents, who weren't too pleased with the sudden appearance of a two-year old boy. Isaac did not have a happy childhood with them. There was no love lost between him and his grandfather, James Ayscough. The old man even excluded him from his will. Isaac was furious. About his mother and stepfather he fantasized that he would "burn [them] and the house over them."

Newton's aim at college was to get - what else - a law degree. At Cambridge that included studying the antiquated texts of Aristotle, which still put the Earth at the centre of the universe and described nature in qualitative instead of quantitative terms. But revolutionary ideas can't be repressed, they just float around at centres of learning, and sometime during his undergraduate days Newton discovered René Descartes' natural philosophy. Descartes viewed the world around him as particles of matter and explained natural phenomena through their motion and mechanical interactions. Through Descartes' writings Newton was led to study - without anyone's knowledge - the important mathematical texts of the time. Then his genius began to emerge. All by himself he created revolutionary advances in mathematics, optics, and astronomy. Single-handedly he invented a new mathematical method to describe motion and forces, which he called the "method of fluxions," eventually known as calculus. To his greatest regret later in life, he never published an account of the method that allowed the computation of areas, lengths of curves, tangents, and maxima and minima of functions. He probably thought that the new technique was too radical a departure from traditional mathematics, and that discussions about it would detract the readers of his astronomy texts from the main results. Therefore he may have deliberately covered his tracks by keeping the invention of differential calculus secret. The treatise in which he eventually described the new method, De methodis Serierum et Fluxionum (On the methods of series and fluxions), written in 1671, would only be published 65 years later, ten years after his death.

At age 53, Newton decided on a career change: he became Warden of the Royal Mint, and three years later, until his death, he was its Master. Since he received a commission on all the coins that were struck, he managed to do quite well for himself. He also pursued counterfeiters relentlessly and with ferocity.

In 1703 Newton was elected president of the Royal Society of London. He was re-elected every year until his death 24 years later. Apart from dozing off during the meetings towards the end of his life, he used his presidency quite to his advantage, as we skissing see. Sir Isaac devoted the last 25 years of his life to a priority dispute with Gottfried Wilhelm von Leibniz over the discovery of calculus - Newton's method of fluxions. The stakes were high: calculus changed mathematics in a fundamental way, and its inventor would forever be remembered for this feat. As President of the Royal Society he appointed an "unprejudiced committee" to decide who had been the first to invent calculus. Of course the unprejudiced committee members knew exactly what was expected of them and didn't even dream of letting Leibniz off the hook. The gentleman from Germany was never even asked for his version of the events. Just to be on the safe side, Newton secretly wrote the committee's final report himself. And to top it off, he also wrote a very favourable review of the report - again anonymously - for the Transactions of the Royal Society.

Nowadays it is generally accepted that Newton deserves priority, having invented calculus in 1665 and 1666. Leibniz apparently re-invented the method, which he called the "method of differences," ten years later. Isaac Newton died on March 31, 1727, in London.

David Gregory

David Gregory

The other participant in the debate on the kissing number was David Gregory, the nephew of the even more famous scientist James Gregory. Newton's junior by 16 years, Gregory was born 1659 in Aberdeen, Scotland, to a very fertile family: he was one of his father's twenty-nine children (by two wives). A precocious child, he started his university education at the tender age of twelve. But the fast start did not last. Rather it ended as fast as it started and Gregory concluded his days as a student without a degree. This did not stop the University of Edinburgh from appointing him, at age 24, professor of mathematics. Gregory was an early supporter of Newton's. In fact, he was the first university lecturer to teach the cutting edge theories that no other university had yet adopted. In 1690, when unrest set in in Scotland, he left for Oxford. Thankful for Gregory's endorsement of his theory, Newton arranged for his appointment as Savilian Professor of Astronomy.

The discussion on kissing numbers began when the 51-year old Newton was between jobs: he already had one foot in the Mint in London, but had not yet removed the other foot from the ivory tower of Trinity College. On May 4, 1694, Gregory paid him a visit. His stay at Cambridge lasted several days, during which the two men talked nonstop about scientific matters. It was a rather one-sided conversation, with Gregory, the dutiful disciple, making notes of everything the great master uttered. He had to hurry because Newton freely related his thoughts, jumping from one subject to another. From editorial corrections to the Principia, he went to the curvature of geometric objects, the "smoke" issuing from a comet, speeds of different colors of light, conic sections, the interaction between Saturn and Jupiter, etc. All this happened at lightning speed, with Gregory attempting to take it all down. When he was not quite able to follow, Newton just took the pad from his friend's hands and scribbled his own remarks into the notebook.

One of the points discussed, number 13 in Gregory's memorandum of that day, was how many planets revolve around the sun. The discussion then went off on a tangent, to the question of how many spheres of equal size could rotate around a central ball of the same size. It deviated again to the distribution of stars of various magnitudes around a central sun. Finally Gregory asked the question: can a rigid material sphere be brought into contact with 13 other spheres of equal size?

In one of the notebooks found by H. W. Turnbull there is a passage in which Gregory discusses the packing of circles that are placed in concentric rings around a central circle, i.e., the two-dimensional problem. He correctly pointed out that six spheres can surround a central sphere in the innermost ring. This is the problem that was proved by Fejes-Toth in 1940. He also remarked that the next rings contain 12 and 18 spheres. Gregory then goes on to discuss the question for three dimensions. How many spheres can be placed in concentric layers so that they all touch the central ball? It is here that he made a claim that sparked the debate and the 250-year search for a final answer. Gregory stated - without further ado - that in three-dimensional space the first layer surrounding a central ball contains thirteen spheres.

Newton, on the other hand, had written in A table of ye fixed Starrs for ye yeare 1671 that there exist thirteen stars of the first magnitude, and in Gregory's report on the discussion of May 4, 1694, we read that in order "to discover how many stars there are of first, second, third etc. magnitude, [Newton] considers how many spheres, nearest, second from them, third etc. surround a sphere in space of three dimensions: there will be 13 of the first magnitude." Of course, Newton meant a total of 13 spheres, including the central one.

So Gregory and Newton did not agree on the number of spheres that can kiss a central ball. If you were to place a bet, whose side would you take? Do you believe in "12 around 1" or in "13 around 1"? Newton would be a safe wager. While Gregory is considered a fair but by no means outstanding mathematician, Newton was correct in most things he ever said or did. But he was not infallible. And - in Gregory's favor - there is enough space for nearly 15 balls, as we saw above.

It turns out that Newton was right: "13 around 1" is impossible. That's why the highest number of balls that can touch a central ball is nowadays often called the Newton number. But during their lifetimes the two men were never to know the correct answer, and after whom kissing numbers would be named. And anyway, being correct is only half the fun in mathematics. The other half is finding the proof, and headway on that track - though no definite progress - was only made 180 years after Newton and Gregory formulated their controversy. The final proof had to wait an additional 80 years and was only formulated in 1953.

About this article

This article is an abridged chapter from George Szpiro's forthcoming book "Kepler's conjecture: How Some of the Greatest Minds in History Helped Solve One of the Oldest Math Problems in the World" (to be reviewed in Issue 25 of Plus). The book will be published by John Wiley & Sons in February 2003.

You can buy "Kepler's Conjecture" from Amazon.co.uk or Amazon.com. You can contact George Szpiro at g.szpiro@nzz.ch.