It might not be immediately obvious why, in a closed population with the same number of men and women, the average number of sexual partners for men must equal the average number of sexual partners for women. Here's a proof.

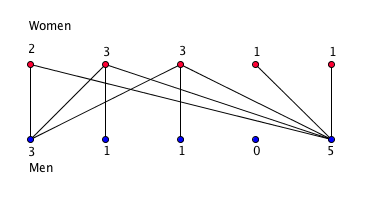
Suppose there are men and women and imagine lining them up in two rows, the women facing the men. Now draw a line between a man and a woman if they have been sexual partners. The number of lines emanating from a woman is the number of sexual partners she has had. Therefore, the total number of lines emanating from the women ( in the picture) divided by the total number of women in the population gives you the average number of sexual partners per woman (which is in the picture).

By the same argument, the total number of lines emanating from the men divided by the total number of men in the population gives you the average number of sexual partners per man. But since each line that emanates from a woman connects to a man, we have Therefore, we have

so the two averages are equal.

Back to the main article