
Making a racket: the science of tennis

As London is heading for the 2012 Olympics, it's not just athletes who are gearing up for action. Engineers, too, are working hard to produce the cutting-edge sporting equipment that guarantees record performances. If you're a tennis player, your most important piece of equipment is your racket. Over recent decades new materials have made tennis rackets ever bigger, lighter and more powerful. So what kind of science goes into designing new rackets?
Until the 1960s tennis rackets were made from wood. Typically they had a long handle and a small, teardrop-shaped head. The racket head had to be small because larger frames would not be stiff or strong enough to support the required tension in the strings without buckling or breaking.
In the 1970s, however, wooden frames were replaced by metal alloy ones. Aluminium alloys were the most popular, but some steel frames were also used. Towards the end of the decade new continuous fibre composites were introduced consisting of a combination of fibreglass (glass fibre reinforced plastic, GFRP) and graphite (carbon fibre reinforced plastic, CFRP). Modern rackets now have graphite as a main component, mixed with a wide variety of materials such as Kevlar fibres, boron nitride ceramic fibres, or more recently, titanium fibres. Most professional players have a least some CFRP in their racket.
Weighty issues

An old-fashioned wooden racket.
In order to be sufficiently stiff and durable, the traditional wooden racket had to be fairly heavy and typically weighed between 370-430g. The move to aluminium alloys and composites enabled manufacturers to maintain the stiff structures but to produce much lighter rackets, with some weighing a little over 200g.
However, a racket with a bigger mass can be useful because of the effect the mass has on the speed of the outgoing ball. We can demonstrate this using the law of conservation of momentum. The momentum of a moving object is the product of its mass and its velocity. The conservation of momentum law effectively states that the total momentum of ball and racket are the same after impact as they were before. We express this using the following equation:
The equation shows that, assuming a similar racket speed for different rackets, increasing the mass of the racket will increase the outgoing ball speed (a measure of racket power). However, the model we used here is only an approximation. In reality the relationship between racket mass and outgoing ball velocity is not quite linear and in practice a large change in mass only results in a small change in outgoing ball speed (see the graph in figure 1 below).
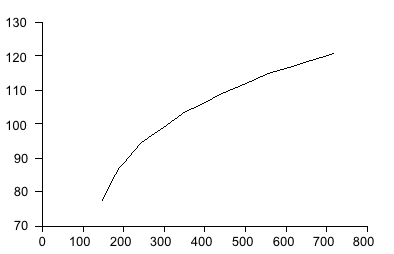
Figure 1: A graph showing the effect of racket mass on ball speed: speed (in kmh) on the vertical axis versus mass (in g) on the horizontal axis.
Moreover, the heavier the racket the harder it is to swing and therefore to control. In comparison, a lighter racket is more manoeuvrable and will permit a much faster swing. Lightweight rackets are great for junior and amateur players, as they are less tiring to use, but many top professional tennis players use relatively heavy rackets — some high street shops sell rackets as light as 200g yet professionals such as Andy Roddick opt for rackets of around 300g and heavier.
Bending stiffness
Another important property of a racket is its bending stiffness, which impacts on the collision of the ball and the racket. A flexible racket will bend more on impact, whereas a stiff racket will bend little. Flexible rackets therefore absorb more of the energy from impact, with more of the energy going into bending of the material. In comparison, stiff rackets are generally more powerful as less energy is lost in frame bending and consequent vibrations and more energy can be returned to the ball via the strings.
So how do you know what material to use for your racket to maximise bending stiffness? A key quantity in this context is called the modulus of elasticity, also known as Young's modulus. As you apply a tensile stress to a material (that is you subject it to a pulling or stretching force), the material elongates as a result. The stress is defined as the load per unit area and is measured using the same units, pascals, as pressure. The ratio between the resulting change in length and the original length is what's known as the strain.
Clearly, the more stress you apply, the greater the strain. The relationship can be visualised by plotting stress versus strain for a given material. When the material is behaving elastically (i.e. the material returns to its former length when you remove the tensile stress, or load) the result is a straight line. The slope of the line, which encapsulates the relationship between stress and strain for the given material, is the Young's modulus
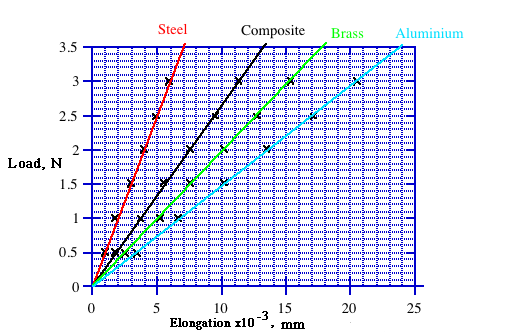
Figure 2: Tensile load plotted versus elongation in the elastic regime for different materials.
The Young's modulus can be measured by hanging loads from the end of a wire of known cross sectional area and measuring the change in length. Figure 2 shows the change in gradient of tensile load versus elongation in the elastic regime for steel, a CFRP composite, brass and aluminium. Steel has the largest gradient, and hence highest Young's modulus.
Designers often look for materials with high stiffness and strength at low density, in order to build lightweight rackets or to increase the size of a racket head while keeping the mass constant. The type of load typically experienced by tennis rackets is bending, that is the racket is held rigid at one end while the ball hits the other end. For high bending stiffness at low density designers look for materials with a high value of
Table 1 shows that wood is a very favourable material for high bending stiffness at minimum weight. However, the lower value of
| Young's modulus E in GPa | Density ρ in mg/m3 | √E /ρ | Strength in megapascals (1MPa=1 million Pa) | |
| CFRP | 90 | 2.0 | 4.7 | 500 |
| Steel | 210 | 7.8 | 1.9 | 400 |
| Al alloy | 71 | 2.7 | 3.1 | 300 |
| Wood (Ash) | 10 | 0.5 | 6.3 | 50 |
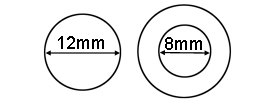
Figure 3: A hollow rod has higher bending stiffness than a solid rod with the same mass. Having the same mass means that the area of the cross section on the left is the same as the area of the ring-shaped region formed by the two diameters on the right.
The use of metals and composites also allows the designers to make hollow frames — it is extremely difficult to make hollow wood components. The bending stiffness of a hollow structure is greater than that for a solid section, for constant mass, hence giving the manufacturers a further opportunity to reduce frame weight whilst maintaining stiffness.
Bending stiffness is proportional to
The sweet spot
The term sweet spot is often used by tennis manufacturers when marketing their rackets, for example, they may advertise that their racket has a larger or better-positioned sweet spot – but what is a sweet spot and where is it located on the racket? There are in fact three separate sweet spots found on a racket face.
The centre of percussion
The centre of percussion is the impact location of the ball where the force (that is the twist) felt by your hand is at a minimum. Consider a racket vertically suspended and at rest. If a ball strikes this racket at its centre of mass, the entire racket will recoil in the direction of the incoming ball in order to conserve momentum. However, if the ball were to strike elsewhere on the racket, the racket would still recoil but also rotate around the centre of mass. If you were holding the racket, this would result in a twisting force felt by your hand. The centre of percussion is the point on the racket at which this twist force is zero. When you're holding the racket its location depends on the weight distribution of the racket and the location of the hand on the handle.
The node
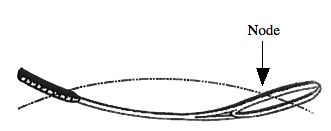
Figure 4: Vibration of a racket showing the fundamental mode.
The node is the ball impact location that produces the lowest level of vibration in the racket. When a ball hits a tennis racket, a large amount of energy involved in the collision goes into deformation of the ball, the strings and the racket frame. Some of this energy returns to the ball in the form of kinetic energy, whilst some becomes energy of vibration causing both the strings and racket to oscillate. Figure 4 on the left shows the fundamental mode of vibration for a tennis racket.
The fundamental frequency, that is the frequency at which the racket naturally vibrates when struck, depends largely on its stiffness and mass of its frame. Usually it is somewhere between 120-200 Hz for modern rackets and at about 90 Hz for traditional wooden rackets. As figure 4 shows, the largest amplitudes in the racket's vibration are seen at the middle and the two ends of the racket. There are two points, called nodes, at which the amplitude is zero. One of the nodes occurs on the racket head — limited vibration occurs when the ball hits this node. The farther from the node the ball hits, the larger the vibration amplitude generated. The nodal point is therefore commonly referred to as the sweet spot due to the vibration-free feeling experienced by the player.
The power spot
In tennis the term power refers to the speed of the outgoing ball relative to the effort the player has exerted in swinging the racket. As the name suggests, the power spot is the location on the strings that provides the maximum power during a shot. You can find this spot by working out a quantity called the coefficient of restitution (COR). The COR is proportional to the ratio between the outgoing ball speed and the incoming ball speed. A perfectly elastic collision has a COR value of 1, as all the kinetic energy of the colliding objects before the collision remains as kinetic energy after the collision, so that the outgoing ball speed is the same as the incoming ball speed.
Obviously, the higher the COR the further the ball will travel for the same racket speed. A simple way of determining the COR is to drop a tennis ball onto the face of a racket from a 1m height and measuring its rebound height. The COR is then found from the ratio of rebound height to drop height. The power spot is found at the point where the coefficient of restitution (COR) is at its maximum.
The sweet spots are influenced by the racket material and design, as well as the tension of the strings. Manufacturers often design rackets so that the sweet spots are in certain locations, for example at the centre of the racket. This is suitable for most players, but for professionals the situation is more complex, as there can be more than one sweet spot.
Smart materials
The latest development in the evolution of the tennis racket has been the inclusion of smart materials in the handle that are capable of reducing frame vibration. The materials used, called piezoelectric ceramics, generate an electric charge when they are under a mechanical force (or stress), and, inversely, produce a mechanical force (or displacement) when an electric charge is applied. When used in tennis rackets, the piezoelectric ceramics convert mechanical vibrations into electrical energy, which, in combination with a microchip, converts that electrical energy back into a mechanical force that stiffens the racket frame and reduces vibration. The majority of players don't like vibrations, as they often result in a loss of control, fatigue of the player and an unsatisfactory feeling when the ball is hit. It is important, however, that not all vibration is eliminated as this is provides feedback to the player about the quality of their tennis shot. The Head sporting goods company have led the way with these new style tennis rackets and players such as Andre Agassi and Sebastien Grosjean have already used them in tournaments.
But is it all good?
There are, however, some disadvantages to the dramatic developments in sporting equipment we described in this article. Over the years the style of tennis has changed considerably and many spectators have been complaining that the pace of the game and, more specifically, the pace of the serve, are making tennis boring to watch. This is particularly true of fast surfaces like the grass courts used at Wimbledon where the pace of a game is significantly quicker than those seen on slower clay surfaces.
Let's compare a serve of 115mph (approximate average 1st serve speed of men's serve at Wimbledon) and one of 155mph (the current record for the fastest men's serve), both travelling approximately 23.77m (the length of the tennis court). For the purpose of this comparison, the calculation assumes that the ball loses no speed during its trajectory and bounce. (In reality the ball will slow and therefore increase the time for the receiver to react.)
The speed of 115mph is equivalent to 51.4 metres per second. Therefore it takes the ball travelling at that speed 23.77/51.4=0.462 second to reach the other side of the tennis court. The same calculation for the speed of 155mph gives 0.343 seconds for the ball to cross the court.
Thus, an increase of 40mph reduces the amount of time a player has to react to a serve by 0.119 seconds. This may not sound like a lot, but in such a fast paced game fractions of a second can really count. When a serve comes to the receiver at increasingly higher speeds, their reaction time will gradually diminish until a physical limit is reached (an athletes reaction time to an external stimulus is about 0.1 seconds).
The International Tennis Federation (ITF), keen to "preserve the nature of the game", have approved three different kinds of ball, a slow one to be used on fast court surfaces, a fast one to be used on slow court surfaces and a medium for the in-between case. It is hoped that the slower balls make the game easier to learn, more fun to play and more stimulating to watch.
About this article
This article first appeared in Physics Review, a magazine for A level physics students. It is reproduced here with kind permission.

Claire Davis
Professor Claire Davis is a chartered engineer and works in the School of Metallurgy and Materials at the University of Birmingham, where she teaches materials science, metallurgy and sports science and materials technology students. Her research interests are focused on microstructure-property relationships in metals.
Elizabeth Swinbank is a physicist who, following a PhD at Cambridge, has taught at both school and university level. She is a Fellow in Science Education at the University of York where she is involved in a variety of science curriculum initiatives. Her current interests include directing the Salters Horners Advanced Physics project, developing resources for the new Extended Project qualification and chairing the editorial board of Physics Review magazine.
Comments
Anonymous
This and other texts I have read concentrate on the elasticity of the racquet. As a tennis player ( and civil engineer ) my experience strongly suggests that the string tension and modulus of elasticity are just if not more important. Many players use different string type in the longitudinal as opposed to the cross strings while others use different tensions. there seems to be little science involved. My inclination is to attempt to share the impact force of the ball equally between the longitudinal and cross strings by using the one string type and a higher tension in the longitudinal strings.
At this stage the mathematics is beyond me so its trial and error. Best results so far are a tension difference of 5 lbs.
Would appreciate any advice.
Garth Wenck . grwenck@gmail.com
Anonymous
helped me do my physics assignment :D thnx alot whoever wrote this!! :D
Andros
It's October 2019 ....
If the ITF were truly interested in "preserving the nature of the game" then they should have dealt with the "polyester trap", i.e. the proliferation of these unsafe-to-the-body polyester strings in tennis. These strings have warped the traditional multi-dimensional game of tennis into an essentially one-dimensional base-line-rally-frenzy-game, promoting an uber-power game, gone (or going) are the "touch" and "style" aspects of the tennis game. The ITF should ban polyester strings (at least the 100% polyester ones) and only allow the traditional tennis string materials such as natural gut, nylon, zyex, polyethylene, polyolefin, polypropylene, Vectran, Kevlar, etc. The scope in these traditional materials is broad enough to make potential hybrid-string setups between these materials an interesting endeavour. FYI, I'm a classic-stroke flat hitter using a non-polyester hybrid string-setup.
The ITF should also support wood-based tennis tournaments.
By "wood-based" I mean the frame is majority wood but may contain minor additions of strengthening/toughening materials like fibreglass/epoxy/graphite/boron/etc. and optional lead (Pb) for custom balance/weight scenarios.
With a "wood" scene, preservation of the "true" nature of tennis is more likely.
Frames have been getting stiffer/lighter and is not healthy for the body.
Inspector Jeff
The racquet is a cantilever with an eccentric force on the strings which is transferred to the hoop all-around. All of these forces end up at your hand in different magnitudes microseconds apart.
Extremely complex calcs.