
It's International Year of Astronomy and all eyes are on Galileo Galilei, whose astronomical observations 400 years ago revolutionised our understanding of the Universe. But few people know that Galileo wasn't the first to build a telescope and turn it on the stars. That honour falls to a little-known mathematician called Thomas Harriot, who might have become a household name, had he bothered to publish his results. This article is a tour of his work.

This portrait was once believed to be of Thomas Harriot, but modern evidence indicates that it is probably not him.
When the prestigious Royal Society was established in 1660, it quickly launched a seven year search for the lost papers of Thomas Harriot, a London mathematician and empirical scientist who had died decades before. The quest failed, but when the papers finally came to light a century later, they included a system of symbolic algebra, workable binary numbers, the sine law of refraction and detailed telescopic observations of the Moon and sunspots: all mathematical and scientific firsts. While Harriot's intellectual achievements had been gathering dust, much of his pioneering work was discovered by others, so his name never made it into the history books.
We know little of Thomas Harriot's background and early life and we can't even be sure of what he looked like — the authenticity of the portrait on the right is disputed. He was born around 1560, four years before Galileo and a lifetime ahead of Newton. Soon after graduating from Oxford University in 1580, he was living and working in the London home of Walter Raleigh, then one of Queen Elizabeth's favoured courtiers. Acting as Raleigh's accountant, Harriot tutored sea captains in navigation and gained early fame as an anthropologist and ethnographer.
Finding his way in the world
At Raleigh's instruction, the twenty-five year old Harriot spent a year in present-day North Carolina on the first, and ill-fated, expedition to set up a New World British colony, on Roanoke Island. Charged with mapping and surveying the new country, Harriot also documented the area's natural history and the customs of the local Algonquin peoples. He viewed the indigenous inhabitants as rightful owners of their land and was probably the first Englishman to communicate with them in their own language, even inventing an alphabet to represent their unique range of linguistic sounds. On his return home he published A briefe and true account of the new found land of Virginia with John White, the first English-language publication on the Americas and one that's still studied on early American literature courses. Harriot also promoted the new habit of smoking tobacco, recommending it as it "purgeth superfluous fleame and ... openeth all the pores and passages of the body".
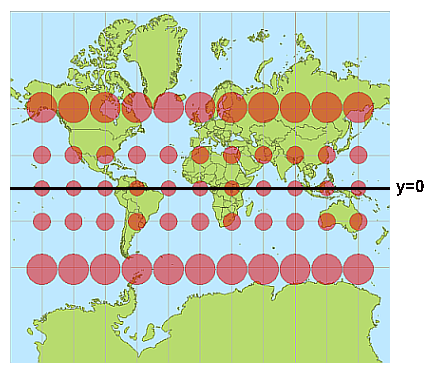
Mercator's projection enlarges areas close to the poles. The red circles indicate the level of distortion. The spacing between the lines of latitude increases as you move towards the poles. Image courtesy Stefan Kuhn.
During his time with Raleigh, Harriot focused his mathematical efforts on navigational matters. The map of the Flemish cartographer Gerardus Mercator was already revolutionising marine navigation, and even today is still the most familiar representation of our world. The Mercator projection was so valuable to marine navigation because it transforms lines of constant bearing on the surface of the Earth (the paths traced out when following a fixed direction as given by a compass) into straight line segments on the map. But like all projections of the spherical Earth onto a two-dimensional map, the Mercator projection comes with some distortion, in this case enlarging the areas near the poles. A measure of this distortion is the spacing between the lines of latitude, which increases towards the poles.
Unfortunately, Mercator had not explained how to calculate the spacing of the lines of latitude on his chart, leaving future map makers none the wiser about the exact distortion of dimensions. In the 1580s Harriot gave a mathematical explanation of the progressive increase of distances between them, and some years later he computed tables of these meridional parts. It was left to
Harriot's contemporary, English Cartographer Edward Wright, however, to publish the first table of latitudes in 1599. Some years later the Scottish mathematician John Napier invented the logarithm, enabling us to express the height
Getting light right
When Raleigh fell out of favour with the Queen for secretly marrying one of her ladies-in-waiting, he was imprisoned in the Tower and Harriot fell under the patronage of Sir Henry Percy, the ninth Earl of Northumberland. Known as the "wizard" earl for his free-thinking interest in science, Northumberland provided Harriot with a handsome pension of £80 a year, spacious rooms at his London home, Syon House (where Harriot lived for over twenty years) and the services of a talented technical assistant. Harriot was now free to pursue a range of scientific interests, but his focus remained on the mathematics relating to seafaring, navigation, surveying and astronomy.

The law of refraction relates the angle at which a ray of light meets the medium (θ1) to the angle of refraction (θ2).
Inspired by his own observations at sea, he developed techniques for correcting errors in navigational tools such as the cross staff, an instrument used to determine a ship's latitude by measuring the altitude of the Sun or pole star. His consideration of the effect of the refraction of light in the atmosphere on these observations may have been what drove him to embark on a systematic experimental and mathematical study of how light is bent by different media.
From the updated table he scrawled in a published book on optics, it's probable these experiments resulted in Harriot calculating the sine law of refraction, which relates the angle at which a ray of light hits the surface of a medium to the angle by which the ray is bent by the medium. Although the law, stated below, had already been mentioned in a tenth century manuscript by the Arab
mathematician Ibn Sahl, Harriot and his contemporaries were unaware of this. Harriot's notes do not state the law explicitly, but the table of angles he derived is too exact to be a result of experiment. His faith in the power of mathematics to describe physical laws must have encouraged him to interpret the results geometrically, and he must have been aware that the ratio of the sines of the two
angles remains constant. In fact, it is equal to the ratio of the speed
With applications for lens design, this relationship would have been of interest for Harriot's later work developing perspective trunckes or telescopes. The Dutch mathematician Willebrord Snellius shared his own discovery of the sine law with friends twenty years after Harriot's work but, though the law is now named after Snellius (also called Snell), it was actually René Descartes who formally published it another decade later.
Ballistics, cannonballs and fireworks
With seafaring comes warfare, and in the early 17th century Harriot correctly depicted the path of a projectile as a tilted parabola, where the descending segment is shorter and steeper than the ascending one, by taking into account gravity acting on the vertical component and air resistance on the horizontal. Galileo experimented with projectiles a few years later, but the parabolic theory was actually published by Bonaventura Cavalieri, one of Galileo's disciples.
On November 4th 1605, Harriot had the misfortune to dine at Syon with his patron, Northumberland, and a distant cousin of the Earl, Thomas Percy, who turned out to be a key conspirator in the gunpowder plot to blow up the Houses of Parliament. Three days later, Percy was dead and both Northumberland and Harriot were arrested. Harriot spent a month in the Gatehouse Prison in Westminster, accused by King James I, who distrusted mathematicians as astrologers and magicians, of secretly casting a horoscope on him. Harriot wrote to the Privy Council protesting his innocence, denying any meddling in matters of state and describing himself as "contented with a private life for the love of learning that I may study freely." He was released a few weeks later. Northumberland was sentenced to life imprisonment in the Tower, where Harriot regularly visited both him and Raleigh, now sentenced to death for high treason.

The face-centred cubic packing (left) consists of layers of spheres. Each layer is positioned so that the spheres rest on the 'holes' of the layer below. This arrangement is not unique, the hexagonal close packing (right) is constructed in a similar way and is equally efficient.
Harriot remained at Syon and soon entered into correspondence with the German mathematician Johannes Kepler, discussing, among other things, the problem of stacking spheres. Harriot had previously drawn up charts showing the ground space needed to store stacks of cannonballs on board Raleigh's ships, and had become intrigued by the question of how to stack the balls most efficiently. This was no doubt the prompter for Kepler's suggestion in 1611 that the most efficient arrangements of equally sized spheres in space are given by the so-called face-centred cubic packing and the hexagonal close packing, shown in the image on the left. This fact is familiar to greengrocers around the world, but as a mathematical statement that requires proof it turned out to be fiendishly difficult. It wasn't until nearly 400 years later, in 1998, that the mathematician Thomas Hales announced a proof which relied on a vast number of computer calculations. No human could possibly check all the calculations within a lifetime, so the validity of the proof has remained controversial to this day. (You can find out more about Kepler's conjecture and the controversy in the Plus article Welcome to the maths lab.)
The two mathematicians also discussed Harriot's work on rainbows and his investigation of refraction. Harriot thought that a light ray hitting the surface of a transparent medium was partially reflected and partially refracted because the medium contained solid parts, akin to atoms (which resist the ray), and empty spaces (which the rays penetrate). He even invited Kepler to imagine entering into an atom with him to see what wonders they would find in "Nature's House". To Harriot's amazement, Kepler declined.
Perhaps inspired by the idea of matter being comprised by either points or empty space, Harriot developed a binary number system almost a century before the German mathematician Gottfried Leibniz, who is usually credited with being the first to describe the modern binary number system, which underlies the workings of all our computers. Harriot manipulated the numbers by addition, subtraction and multiplication. His papers even show him experimenting with ternary, quaternary, quinternary and higher number systems.
Reaching for the stars and venturing into abstraction
By the summer of 1609, Harriot had acquired a magnifying "truncke" or telescope, which could magnify objects by a factor of six. His observations of the moon are the first ever recorded astronomical observations using a telescope. As ever, he didn't publish, and Galileo gained the credit when he made his own observations six months later. Harriot used telescopes which could magnify up to a factor of 32 to draw detailed lunar maps, follow Jupiter's satellites, track comets and catalogue nearly 500 sunspot observations.

The Hubble Space Telescope: Astronomy has come a long way since the time of Harriot. Image courtesy NASA.
Despite the impressive list of achievements above, Harriot is actually best-known for his work in algebra and, in his will, it was only his mathematical papers that he requested be collected and published. There is much debate about how important Harriot's algebraic work was, mostly due to the disorganised structure of his papers and the badly edited collection produced after his death. But his symbolic system of algebra, which dispensed with wordy descriptions and represented unknowns with letters and operations with symbols, could be manipulated independently from what it represented, according to its own rules and axioms. If this seems familiar to anyone who's studied algebra at school, it is. Like his earlier representation of the Algonquin language, Harriot's visual symbolism made mathematics accessible in a totally new way.
Harriot wasn't just responsible for developing new representations of mathematics, though. He tackled equations by carrying all the terms containing a power of the unknown to the left side of the equation, with the constant term on the right. He used algebraic zero and the concepts of greater than and less than, expressing them as symbols.He worked on cubic equations,
coming up with symbolic solutions and noting that a cubic
For the last eight years of his life, Harriot was plagued by a cancerous tumour on his nostril, almost certainly caused by smoking. He died aged 61 while visiting the City of London. His grave in the Chancel of St Christopher le Stocks was destroyed in the Great Fire of 1666 but a modern plaque stands on the site, now part of the Bank of England.
Publish or perish?
In accordance with his will, the Artis Analyticae Praxis, a collection of Harriot's work on the theory of equations and the solution of numerical polynomial equations, was published ten years after his death. His reputation for many years relied on this edited volume which included many mistakes and discrepancies from his original papers.
Why didn't he publish any of the 7000 manuscript pages in his lifetime? No one is quite sure. Perhaps the security of his annual pension meant he had no need to establish a reputation for himself. Perhaps he considered his discoveries the property of his patrons. Perhaps he just wasn't interested. His lifelong friend, Sir William Lower, wrote in 1610 that "too great reservedness hath rob'd you of those glories" and urged Harriot to let his "countrie and frinds injoye the comforts they would have in the true and greate honor you would purchase your selfe by publishing some of your choise works", but to no avail.
When Harriot's papers were finally unearthed in the late 18th century, among the stable accounts of Northumberland's country home, Petworth House in Sussex, they were disorganised and muddled, with few descriptions to help interpret them. Many were obviously written for Harriot's own personal entertainment, including acrostics, anagrams, hieroglyphics and puzzles. In among his major investigations were diversions to calculate the optimum size for the mast of a ship, the maximum supportable population of the world and the gaseous yield of a burning candle, as well as plans for the redesign of Syon House's drainage systems.
The German astronomer who discovered the papers, Frans Xavier Zach, was quick to promote Harriot's great work to the world, but there is still much debate about the level of his achievements. While descriptions of Harriot as an English Galileo or Kepler may be misplaced, there is no doubt that he committed his life to finding mathematical descriptions of observations of the natural world and applied himself to an amazingly broad range of practical problems. If he had published in his day, perhaps his contribution could have been greater, and his name would be much more familiar to us all.
Further reading
A more extensive biography, along with details of Harriot's work on binary numbers and the story of his lost papers can be found in the MacTutor History of Mathematics archive.
You can also read Harriot's only published works, A Briefe and True Report of the New Found Land of Virginia and Artis Analyticae Praxis.
About the author

Anna Faherty read physics at Cambridge before working in publishing for fifteen years. She commissioned the Modular Mathematics Series and recently worked on 50 Mathematical Ideas You Really Need to Know. Anna is now a freelance writer and consultant working with a diverse range of clients including the Science Museum, the National Maritime Museum and Time Out London Guides.
Comments
If Thomas Harriot had published in his day . . .
I have read that if Thomas Harriot had published in his day he would have been in danger of the inquisition.
According to the writer, he chose life over fame. Is that a fact as you understand his situation ?
Thanks,
Jim Overson
Jim.4iMR@gmail.com
Hariot and the Inquisition
No danger of the Inquisition in a Protestant country!