June 2000
Introduction
"Neither a borrower nor a lender be."
Today, nobody heeds the advice of Polonius to his student son Laertes. Everybody borrows and lends all the time.
- Children put their pocket money into a bank account to save up to buy a bicycle.
- Students take out loans to finance their studies.
- Credit cards are widely used for short-term borrowing.
- Young couples buy houses on mortgages of 20 to 30 years, and save for their retirement.
Understanding lending and borrowing means understanding compound interest. For a career in the world of finance, that understanding has to be broad and thorough.
In this article we will not attempt a comprehensive coverage of all the intricate details of the subject, but will try to show that compound interest
- is an essential component of numeracy,
- involves some interesting maths,
- throws up some surprising, even paradoxical results from time to time.
A slice of history

Figure 1: The real purpose of the ziggurat?
The application of mathematics to trade and financial affairs is as old as mathematics itself. History shows that as soon as a civilisation develops writing, including the writing of numbers, the new technology is used in financial transactions and the appropriate mathematical ideas develop rapidly.
Over 4000 years ago the Sumerian merchants of Babylon and Nineveh recorded bills, receipts and promissory notes in cuneiform script on clay tablets. They drew up multiplication tables and tables of reciprocals, squares, cubes and exponentials, and used them to calculate compound interest and mortgage repayments. A clay tablet now in the Louvre tackles the problem of finding how long it will
take a sum of money to double at an interest rate of 20%.
Hindu mathematicians of 500-1000 A.D. knew how to sum arithmetic and geometric series, and could solve problems involving discount and interest calculations.
There is a record of a loan agreement in London in 1183. The interest levied was 2d. in the pound per week, amounting to 43% per annum. An even higher rate was charged in 1235 by London merchants who lent money to a priory, demanding "every two months one mark for every ten marks as a recompense for losses" - an annual interest rate of 60%.
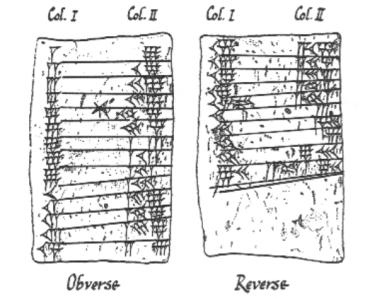
Figure 2: The 9 times table in Babylonian cuneiform
In 15th century Italy there was a tremendous increase in commercial activity. Double-entry book keeping was introduced, and textbooks in arithmetic were published: in Latin for the classical scholars in church schools, and in the vernacular for preparing students for commercial careers.
Logarithms (invented by John Napier in 1614) revolutionised financial mathematics. Only in the 20th century were they replaced by mechanical, and later electronic calculators.
Time is money

Figure 3: Time is money?
When you borrow money from a lender such as a bank, you pay the lender interest - a fee for the use of the money. Interest is charged as a percentage of the principle (the amount of the loan) for an agreed period, usually a year.
If the principle is and the interest rate is , then the interest is . At the end of the year the loan plus the interest must be repaid: a total of . \par If you make no repayment, your debt is now , which must be repaid at the end of the second year with interest of : a total of . \par After three years you will owe , and after years you will owe This is the basic formula for calculating compound interest. \par The same formula applies when you deposit money into a savings account. Now you are lending money to the bank, which pays you interest on your
deposit. If you deposit an amount at an interest rate , after years your investment will be worth . \par The value of money is thus seen to be time dependent. If denotes the {\it present value} of a sum of money, and denotes its {\it future value} in years at an interest rate of , then These simple formulae are the key to understanding complex investment and debt repayment schemes.
Frequent compounding
The basic compound interest formula assumes that interest is paid once a year. Sometimes interest is payable more frequently. A fixed deposit investment may pay interest quarterly, and car loans and mortgages are paid monthly.
If \pounds{1000} is invested at 8\% for a year, it will be worth, at the end of the year \par As the frequency of compounding increases, so does the effective rate, but successively more slowly. \par In general, if interest is compounded times a year at an interest rate of , after years an investment grows to What happens as ?
Continuous compounding
The core mathematical issue here is the behaviour of the sequence as . \par Every calculus textbook shows that this sequence increases, but not without bound (it is not too difficult to prove that for all ). In fact, the sequence converges to a limit whose value (to
7 decimal places) is 2.7182818. This number is denoted by . It plays a very important role in calculus. \par It can then be proved that, as , which is the value of an investment of after years at an interest rate of , compounded continuously. \par An alternative method of deriving the
formula for continuously compounded interest is to set up and solve a {\it differential equation}. \par Let be the value of an investment at time , which is earning interest at a rate . The initial investment (when ) is . Then over a short time interval from to , the interest earned is , and hence
\par Letting gives the differential equation This is a separable
differential equation, and is solved as follows: which is the formula for continuously compounding interest we obtained before.
Finance and physics
The differential equation describes exponential growth or decay. Similar equations arise in physics, notably in radioactive decay. This is not a chance occurrence, as there are a number of problems in financial mathematics where the underlying mathematics also models a physical process. Brownian motion describes molecular motion in a fluid, and also the
random movements of the stock market. The famous Black-Scholes equation used in options and derivatives pricing has close analogies in equations describing diffusion processes in physics.
How many days in a year?
In calculating interest over a part of a year, say 137 days, it would seem natural to use the fraction (or in a Leap Year). Some financial institutions, however, use an {"}interest year{"} of 360 days. This can make a significant difference. Interest on a million pounds at 8\% per annum over ths of a year is \pounds{30444.44}; over
ths of a year is \pounds{30027.40}: \pounds{417.04} less. \par A 360-day interest year is used in several European countries and the United States. Britain and Japan use a 365-day interest year, even in a Leap Year. Some Euro-Sterling bond issues accept that there are 366 days in a Leap Year. \par For a daily compounding of interest, however, it makes very little difference
whether the year is taken as 360, 365 or 366 days. For example, at 8\% On a million pounds over one year, the difference is at most 16 pence.
Double your money
How long will it take for your money to double when it is invested in a savings account at 5\%? And how long will it take to double at 7\%? A simple rule of thumb says that at \%, money will double in years. So at 5\%, a deposit doubles in 14 years, and at 7\% it doubles in 10 years. This is the "Rule of 70". \par How is the rule derived? If an amount is invested at an
interest rate of , the problem is to determine such that Some easy algebra gives The log function used could be to any base, but it turns out to be a good idea to use logs to base (natural logarithms, denoted by ). Then The reason for choosing natural logarithms is that there is a representation of the function as a {\it Maclaurin series} Since is small, the terms of the Maclaurin
expansion may be neglected, so that . So Replacing 0.6931 by the nicer rounder number 0.7000 gives when \%. The table below compares the doubling time given by the rule of 70 with the correct doubling time.
\par The Rule of 70 would be more accurate as a "Rule of 69.3". But 70 is a more manageable number.
In fact, 72 has more divisors than 70, and a "Rule of 72" serves almost as well as the Rule of 70. \par The rule of 70 was derived under the assumption of annual compounding. What happens with continuous compounding? Now the equation to solve is without having to use the
Maclaurin expansion.
Regular investment pays
At the beginning of each year an amount is invested in a savings account which pays interest at a rate . How much will be in the account at the end of years? The total investment will therefore be worth For example, if \pounds{1000} is invested at the beginning of each year into an account earning 10\% interest, at the end of 7 years the investment will be worth
Early investment pays
When Alice and Bob were both 19 years old they started new jobs. Alice started a savings programme, investing \pounds{1000} at the beginning of each year at 10\% per annum. At the end of 7 years her account shows a total of \pounds{10435.89} (see the calculation above). \par Bob, who has not saved a penny until now, is impressed by the size of Alice's nest egg, and decides to start
investing in the same way. Alice decides not to put any more into her account, just letting it grow at 10\% per annum. Bob is determined to build up his investment until it is bigger than Alice's. How long will it take for Bob's investment to overtake Alice's? \par The astonishing answer is that it will take 32 years! \par If you find this hard to believe, check the calculations below. \par After
31 years, Alice's investment will be worth Bob's investment will be worth and Alice is still ahead of Bob. \par After 32 years, Alice's investment will be worth Bob's investment will be worth and Bob has at last overtaken Alice.
Repayment of loans
When you buy a house or a car, you borrow the purchase price from a bank, and agree to pay the money back in regular equal instalments over a certain time. If the amount of the loan is , the interest rate is and the number of repayments is , what is the amount of the repayment ? \par The key is to look at the problem from the point of view of the bank: their loan must
be balanced by the present value of the repayments. \par The total present value of all the
repayments is therefore which is a geometric series of terms with first term and common ratio . The sum of the series is When this is equated to , we obtain \par For example, a loan of \pounds{100 000} is to be repaid over 25 years in equal monthly instalments. If the annual interest rate is 7\%,
what is the monthly repayment? \par Here and (since the repayments are monthly). So \par The repayments are made up of two components: interest on the outstanding balance, and
an amount of capital repayment. The table below shows the breakdown of the first three repayments. \par \par Initially, the monthly repayment is largely interest, with only 17.46\% of capital repayment. The interest component falls and the capital repayment rises as the payments are made. \par After 20 years, what proportion of the capital has been repaid? The answer could be found by continuing the above table for 240 lines, an easy exercise on a spreadsheet. A more elegant solution
is to realise that after 240 payments, the outstanding capital is precisely the present value of the remaining 60 payments: So more than a third of the capital is still outstanding, even after 80\% of the repayments have been made. \par Suppose that, for purely numerical neatness, it is decided to round the monthly repayment up from \pounds{706.78} to \pounds{710}. How does this affect the number of repayments? \par From the formula
relating and we obtain and thence, putting , and we obtain \par So a substantial
saving of more than 3 repayments is made with a very small "rounding up". Instead of 300 payments of \pounds{706.78}, totalling \pounds{212034}, 296.35 payments of \pounds{710} are made, totalling \pounds{210408.50}. The difference is \pounds{1625.50}! \par Rounding the repayments up to \pounds{720} per month saves a further 10 repayments at the end. Check the figures yourself!
Compound interest deception
As we have seen more than once, compound interest can produce surprises. The unexpected way in which compound interest works can be turned around by dishonest advertisers. Here is an example. \par A loan of \pounds{2000} is offered at "{only 5\%}", to be repaid over a year in monthly instalments. The catch lies in the incorrect, but plausible way in which the instalments are calculated.
\par "{At 5\% per annum, the interest on \pounds{2000} is \pounds{100}, making a total of \pounds{2100}. So the monthly repayments are .}" \par A closer look reveals that the true interest rate is a little over 9\%. To find the true interest rate, we use the formula with . The monthly rate, , is the unknown. Putting and multiplying up gives which simplifies to (An obvious solution is , which corresponds to , clearly not a solution of
the original problem. But then we recall that we multiplied through by , and that is how this solution came about.) So we must find a solution of other than . Some trial and error with a calculator shows that is a solution, corresponding to an interest rate of 0.75872\% per month, or a little over 9.1\% per
annum.
Simon Stevin's Problem

Figure 4: Simon Stevin's statue in Bruges
The mathematician Simon Stevin of Bruges (1548-1620) is famous for introducing decimal fractions into arithmetic. In a textbook of commercial arithmetic published in 1585 he set and solved the following problem:
\par His method of solution was to refer to interest tables to find the interest rate which best fitted the figures, which turned out to be 8\%. This was the only effective method of solving such
problems until the advent of calculators and computers. \par In mathematical terms, Stevin's problem is the same as the problem in the previous section, so we will end this introduction to compound interest with the words of many a maths textbook: "the details are best left to the reader".
About the author
John H. Webb was born in Cape Town and studied mathematics at the University of Cape Town. He won a scholarship to Cambridge where he obtained a Ph D. Back at the University of Cape Town, his career as a research mathematician was eventually overtaken by interests in mathematics
education, with particular emphasis on popularising mathematics and identifying and stimulating promising students.
He edits Mathematical Digest, a quarterly magazine for high schools, runs a maths competition for schools in the Cape Town area, and directs a nationwide Mathematical Talent Search, a problem-solving programme by correspondence which selects and trains South African teams for the International Mathematical Olympiad.
He is at present on sabbatical leave, and is spending six months working with the Millennium Mathematics Project in Cambridge. His visit is supported by the Institute of Actuaries.






Comments
Thank you!
Thank you, John - quite simply the clearest and best-written description of what initially seemed quite a complex mathematical problem. Am happy to be looking at mortgages (and those 'magical' online mortgage repayment calculators) with a whole lot more confidence!
Cheers,
Jonathan
Compound Interest
This is fantastic. Probably the most clear, concise and downright interesting Mathematical article I've read!
Compound Interest Deception
Dear John,
I'm struggling to understand the example for compound interest deception.
If the guy borrows 2000 sterling and has to pay back 2100 then annual interest rate is surely 5%.
Where is the 9% coming from? You use expression "true interest rate", am I missing the point?
I'm sorry if this is a bit blunt, I just like maths and to understand it, hence the challenge with deception for compound interest. Matt
If it was repaid at the *end*
If it was repaid at the *end* of the year in one lump sum, it is my understanding that would be 5%. If they give you 2000 at 5% and you pay them back at the end of the year 2100, that is 5%. You had use of the money the entire year. BUT because you are repaying monthly, you are paying them back both interest and principal. So in month one you've borrowed 2000 for the year. But in month two, you don't owe them 2000 any more, right? You already paid some of it back - which they can now lend to someone else. (they have use of the money). But if you've paid them some of the principle back, you don't "owe" interest on that part anymore because you aren't borrowing that portion of it anymore. But if you aren't borrowing that part anymore, why the heck are they charging you 5% on that part still? They have it back. However, they continue to charge you interest on money you've already put back in their pocket and turn around and do the same thing to someone else! Or something along those lines.
Regular saving and compounding
Thanks for your great work here. In my early forties and only getting around to studying compounding last year (2015). I should have studied it years ago :) but it's never too late I think. Very interesting. Seamus in Ireland.