Spheres within spheres: Simplicity and failure

As we explained in the first part of this article, mathematicians would ideally like to understand the homotopy groups 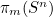$\pi _ m(S^ n)$ for all possible combinations of 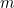$m$ and $n.$

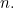
It turns out that this is an unachievable quest at present. Luckily, though, mathematical nature provides us with a wonderful simplification. As the mathematician Hans Freudenthal proved in 1937, the homotopy groups 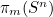$\pi _ m(S^ n)$ are the same (technically they are isomorphic) whenever the difference 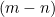$(m-n)$ between the dimensions involved is the same, as long as 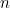$n$ is large enough. This means that there are whole chains of homotopy groups which are all the same.

For example,

$\pi _4(S^3) \cong \pi _5(S^4) \cong \pi _6(S^5) …$

Here the difference between dimensions is 1

$\pi _6(S^4) \cong \pi _7(S^5) \cong \pi _8(S^6)…$

Here the difference between dimensions is 2

$\pi _8(S^5) \cong \pi _9(S^6) \cong \pi _{10}(S^7) …$

Here the difference between dimensions is 3

The symbol $\cong$ means that the groups are essentially the same (i.e. isomorphic).

The homotopy groups that lie within these chains are called stable homotopy groups. There's one stable homotopy group for each integer: one homotopy group for the difference between the dimensions being 1, one homotopy group for the difference between dimensions being 2, and so on. "So we can try an easier problem first, which is only to study these stable homotopy groups, rather than all homotopy groups" says Schlank.

Dramatic failure

The mathematician Hans Freudenthal made important contributions to homotopy theory. This portrait was taken in 1957. Image: Hofland, L.H., Het Utrechts Archief, CC BY 4.0.

Easier it may be, but the problem of understanding the stable homotopy groups is still a huge challenge. Douglas Ravenel, who posed the telescope conjecture back in 1984 has said that he doesn't expect them all to be fully understood in the lifetime of his grandchildren. This is why mathematicians have stopped focussing on trying to understand more and more individual stable homotopy groups and instead try to understand their overall structure. Ravenel has compared this to being in a huge mansion and deciding that, instead of looking into each individual room, you want to understand the structure of the whole building.

The telescope conjecture provided a potential method of getting to grips with this overall structure. The conjecture has now been disproved by Burklund, Hahn, Levy and Schlank. This means that the method suggested by Ravenel doesn't work. "I think it's fair to say that, not only did we prove that this method doesn't work, but that the method fails drastically. The [mansion] is much more complicated than was predicted," says Hahn.

Not all is lost

This doesn't mean that we're back to square one, however. The things we do know about stable homotopy groups suggests that a certain pattern arises in their collection. You can break this pattern up into what you might think of as wavelengths (in analogy to light), each individual wavelength exhibiting periodic phenomena. "Those periods are there, but they appear in a more complex way than the most optimistic possible hope with the telescope conjecture," says Schlank. "But nevertheless, the way we disproved the telescope conjecture does give us positive information," says Schlank. "For example, it gives us a lower bound on how large those groups are."

Burklund, Hahn, Levy and Schlank announced their result at the Oxford conference to huge acclaim. "It was a special moment for all of us," says Hahn. "[Ravenel] was there, but also Mike Hopkins who had proved most of Ravenel's conjectures along with his collaborators, apart from the telescope conjecture. So in the room there was the whole history of people who had interacted with these conjectures. We really appreciated the conference which brought all these people together. It seemed like the perfect time to explain our work." Indeed, the conference was held in honour of Mike Hopkins. It was part of a two week programme organised by the Isaac Newton Institute for Mathematical Sciences (INI). The second part, called Homotopy: Fruit of the fertile Furrow, took place at the INI in Cambridge.

With all of Ravenel's conjectures now settled, nearly forty years after they were posed, the field of homotopy theory sets off in new directions in its quest to understand stable homotopy groups. The INI has played a part in this quest for some time: the recent conference followed up on a research programme held there in 2018. Perhaps it will also host the announcements of important results in the future. If we're still around, we'll be sure to report on them.

About this article

Jeremy Hahn is Assistant Professor at the Mathematics Department of the Massachusetts Institute of Technology.

Tomer Schlank is Visiting Associate Professor at the Mathematics Department of the Massachusetts Institute of Technology.

Jeremy Hahn

Tomer Schlank

Marianne Freiberger is Editor of Plus. Together with Dan Aspel from the INI, she interviewed Hahn and Schlank in July 2023. You might also want to listen to this episode of the INI's Living Proof podcast featuring the interview.

This article was produced as part of our collaboration with the Isaac Newton Institute for Mathematical Sciences (INI) – you can find all the content from the collaboration here.

The INI is an international research centre and our neighbour here on the University of Cambridge's maths campus. It attracts leading mathematical scientists from all over the world, and is open to all. Visit www.newton.ac.uk to find out more.