One morning early in 1913, he found, among the letters on his breakfast table, a large untidy envelope decorated with Indian stamps. When he opened it, he found sheets of paper by no means fresh, on which, in a non-English holograph, were line after line of symbols. Hardy glanced at them without enthusiasm. He was by this time, at the age of thirty-six, a world famous mathematician: and world famous mathematicians, he had already discovered, are unusually exposed to cranks. He was accustomed to receiving manuscripts from strangers, proving the prophetic wisdom of the Great Pyramid, the revelations of the Elders of Zion, or the cryptograms that Bacon has inserted in the plays of the so-called Shakespeare.
So Hardy felt, more than anything, bored. He glanced at the letter, written in halting English, signed by an unknown Indian, asking him to give an opinion of these mathematical discoveries. The script appeared to consist of theorems, most of them wild or fantastic looking, one or two already well-known, laid out as though they were original. There were no proofs of any kind. Hardy was not only bored, but irritated. It seemed like a curious kind of fraud. He put the manuscript aside, and went on with his day's routine...
That particular day, though, while the timetable wasn't altered, internally things were not going according to plan. At the back of his mind, getting in the way of his complete pleasure in his game, the Indian manuscript nagged away. Wild theorems. Theorems such as he had never seen before, nor imagined. A fraud of genius? A question was forming itself in his mind. As it was Hardy's mind, the question was forming itself with epigrammatic clarity: is a fraud of genius more probable than an unknown mathematician of genius? Clearly the answer was no. Back in his rooms in Trinity, he had another look at the script. He sent word to Littlewood that they must have a discussion after hall...
Apparently it did not take them long. Before midnight they knew, and knew for certain. The writer of these manuscripts was a man of genius.
"A Mathematician's Apology" by G. H. Hardy, from the Foreword by C. P. Snow

An image of Ramanujan projected over David Annen as Hardy in A disappearing number. Photo: Robbie Jack.
So C. P. Snow described, in his foreword to G. H. Hardy's A Mathematician's Apology, the day Hardy received his first letter from Srinivasa Ramanujan, the unknown Indian mathematician with whom he would go on to form one of the most remarkable collaborations in the history of mathematics. It was this scene, and the poetic descriptions of mathematics by Hardy in his book, that inspired Simon McBurney (Artistic Director of Complicite) to create a show based on Ramanujan and Hardy's story, and the mathematics they created. But rather than a straight portrayal of the lives and relationship of Hardy and Ramanujan, Complicite created a play (most recently performed at the Barbican in London) intertwining the past and the present, with mathematical ideas and concepts woven into the story.
At the heart of the play are two relationships: one set in the past, the mathematical collaboration between G.H. Hardy and Srinivasa Ramanujan, and the other set in modern day, a love story between a mathematician, Ruth, and her husband, Al. The depiction of Hardy and Ramanujan's extraordinary mathematical collaboration shows them as romantic heroes on a quest for truth, with all the passion, hardships and joy that such a quest should involve. In the scenes of the mathematicians at work, Complicite manage to convey the incredible passion and creativity of mathematical research. At one point, while Ramanujan and Hardy are shown working together at a blackboard, musical patterns created by Nitin Sawhney repeat over and over again, building to a climax that seems to evoke the frenzy of creativity and climax of discovery mathematicians experience in their research work.
Hardy and Ramanujan weren't just mathematical collaborators, they were also great friends. Indeed, in his book Ramanujan: Twelve Lectures on Subjects Suggested by His Life and Work, Hardy said, "my association with him was the one romantic incident in my life". And despite the reserved nature of Hardy's character, this personal relationship comes across strongly in the play. Unusually for portrayals of mathematicians (who often appear in popular culture as cold, insane or friendless), the play rejects the common stereotypes of mathematicians. Instead, all the characters as portrayed as human beings, experiencing the passions, desires, fears and frailties that we all do.
The second relationship also depicts a quest, but one that is more familiar: the personal quest for love, marriage and family. If the depiction of Hardy and Ramanujan is of two extraordinary heroes, the depiction of the mathematician Ruth and her husband Al is one we are much more likely to identify with. Ruth's character shows great passion for her mathematical work, but it is the very personal story of her relationship with Al, from meeting in a maths lecture, to marriage and trying for children, that forms the heart of the play.
Archimedes will be remembered when Aeschylus is forgotten, because languages die and mathematical ideas do not. "Immortality" may be a silly word, but probably a mathematician has the best chance of whatever it may mean.
G. H. Hardy, "A Mathematician's Apology" p81
The play opens with Ruth giving a maths lecture (the same lecture where she meets Al for the first time) explaining the maths contained in Ramanujan's very first letter to Hardy. Among many results in this letter was the following remarkable equation:
![\[ 1 + 2 + 3 + 4 + ... = -\frac{1}{12}. \]](/MI/5d5e9a4ea546cac526cee275362ed9e7/images/img-0001.png) |
The series 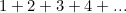 is a divergent series: the sum gets larger and larger the more terms you add. In fact you could say that
is a divergent series: the sum gets larger and larger the more terms you add. In fact you could say that
![\[ 1 + 2 + 3 + 4 + ... = \infty . \]](/MI/7ccb04e4716e02ffbf1a5384b3366fa6/images/img-0001.png) |
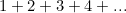 he had in fact substituted
he had in fact substituted  into Euler's zeta function
into Euler's zeta function ![\[ \zeta (s) = 1 + \frac{1}{2^ s} + \frac{1}{3^ s} + \frac{1}{4^ s} + ... = \sum _{k=1}^\infty \frac{1}{k^ s}. \]](/MI/7ccb04e4716e02ffbf1a5384b3366fa6/images/img-0004.png) |
 is greater than one, in which case the series converges to a
finite number.
is greater than one, in which case the series converges to a
finite number.
However, there is another function, known as the Riemann zeta function,which agrees with Euler's zeta function for all  , but has a defined finite value for all other
, but has a defined finite value for all other  , including
, including  . (Well, almost all: the Riemann zeta function is defined for all the complex numbers, apart from
. (Well, almost all: the Riemann zeta function is defined for all the complex numbers, apart from 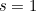 where it is infinite as it is the sum of the harmonic series.) The Riemann zeta function is an extension of Euler's zeta function, and is particularly important because it gives deep insight into the distribution of prime numbers.
where it is infinite as it is the sum of the harmonic series.) The Riemann zeta function is an extension of Euler's zeta function, and is particularly important because it gives deep insight into the distribution of prime numbers.
The formulas used to express the Riemann zeta function for all values of  are far more complicated than Euler's original series. However, to calculate the value of the Riemann zeta function for
are far more complicated than Euler's original series. However, to calculate the value of the Riemann zeta function for 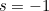 , we can use its functional equation, that is, an equation that describes the value of the Riemann zeta function,
, we can use its functional equation, that is, an equation that describes the value of the Riemann zeta function, 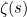 , at one point in terms of its value at another point. The functional equation
, at one point in terms of its value at another point. The functional equation
![\[ \zeta (s)=2^ s\pi ^{s-1}\sin \left(\frac{\pi s}{2}\right)\Gamma (1-s)\zeta (1-s) \]](/MI/1d8bede38b006d82647a304aefc3c25d/images/img-0004.png) |
 , in terms of its value at the point
, in terms of its value at the point 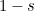 .
.
Most of the terms in the functional equation are familiar, perhaps apart from the Gamma function,  , which is similar to the factorial function but defined for all real and complex numbers. All we need to know about
, which is similar to the factorial function but defined for all real and complex numbers. All we need to know about  here is that for every positive integer
here is that for every positive integer  , it is defined as the factorial of
, it is defined as the factorial of 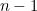 :
:
![\[ \Gamma (n) = (n-1) \times (n-2) \times ... \times 2 \times 1. \]](/MI/a0be6ad3786a570ebafbd7b06f4a8cfc/images/img-0005.png) |
Substituting 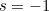 into the functional equation gives
into the functional equation gives
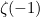 |
 |
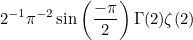 |
|||
 |
 |
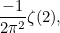 |
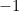 in terms of the value of the function at the point
in terms of the value of the function at the point 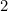 . And as
. And as 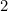 is a real number greater than one, the Riemann zeta function coincides with Euler's zeta function at this point:
is a real number greater than one, the Riemann zeta function coincides with Euler's zeta function at this point:  |
 |
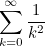 |
|||
 |
 |
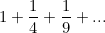 |
The problem of finding the value this series converges to is known as the Basel problem. Euler found the solution in 1735, showing that
![\[ \zeta (2)=\frac{\pi ^2}{6}. \]](/MI/c20836425f8072d2994c8d1ce3ed775b/images/img-0001.png) |
So we can now calculate the value of the Riemann zeta function at  :
:
![\[ \zeta (-1) = \frac{-1}{2\pi ^2}\zeta (2) = \frac{-1}{2\pi ^2}\times \frac{\pi ^2}{6} = -\frac{1}{12} \]](/MI/db2994996c598864cae778940e56737c/images/img-0002.png) |
This is exactly the answer Ramanujan gave in his letter to Hardy. This surprising result showed Hardy that Ramanujan had managed to derive the Riemann zeta function and its functional equation correctly himself. In fact Ramanujan, almost entirely self-taught, had reinvented many other areas of Western mathematics, on his own. He was a mathematical genius with an intuitive connection to mathematics. And Hardy displayed his own mathematical genius by seeing through Ramanujan's idiosyncratic notations to uncover the genius beneath.

Saskia Reeves as Ruth in A disappearing number. Photo: Tristram Kenton.
In the opening scene of A disappearing number the character of Ruth demonstrates this proof to the audience, immediately establishing a parallel between the mathematics lecture theatre and the theatre we were sitting in watching the play. Communicating maths is a performance, and in doing so lecturers weave for their students a mathematical story. And here we were, as an audience, suddenly finding ourselves in a sea of mathematics. The audience laughed nervously as the maths got more and more complex. You could feel the frisson of fear: were we really expected to understand what was going on? In a way it felt like the company was playing with the fear of maths that many people (sadly) feel. Comfortable in the safe surrounds of a theatre, they were suddenly thrown into the mathematical deep end. For a few minutes you could feel the audience's growing concern that they should be following the mathematical reasoning on the board. The tension was shortly broken when another character stepped on stage and the play moved on, but it was an exciting introduction. It's particularly exciting to see "real" mathematics literally on stage.
A mathematician is working with his own mathematical reality. 317 is prime, not because we think so, or because our minds are shaped in one way rather than another, but because it is so, because mathematical reality is built that way.
G. H. Hardy, "A Mathematician's Apology" pp 129-130
One of the most intriguing elements in the play is the use of mathematical concepts to express human emotions and the human condition. In the post-show talk, Simon McBurney explained that the play explores the connection between mathematics and real life. But rather than focussing on the direct connection we more usually talk about in Plus — how maths is used to describe the reality of our physical world — the play explores how mathematical concepts can also describe emotional experiences. One character uses infinity to describe the place they'll go when they die. Partitions of numbers are enacted on stage by partitioning people or objects, echoing the physical separations of characters in the play at that time. And the physical decomposition of dead bodies is compared to the decomposition of numbers into their prime factors, prime numbers being the bones of mathematics.
One particularly lovely example is the use of the convergent infinite series
![\[ \sum _{n=0}^{\infty } \frac{1}{2^ n} = 1+\frac{1}{2}+\frac{1}{4}+\ldots = 2 \]](/MI/ee8994ae6da9dd64bed4e81350d27592/images/img-0001.png) |
To see how this series converges we look at the partial sums, the sum of the series up to the  th term
th term
![\[ S_ n = 1 + \frac{1}{2} + \frac{1}{4} + ... + \frac{1}{2^ n} \]](/MI/d357264fda95670ebe35659fc87cfe54/images/img-0002.png) |
![\[ S_ n - \frac{S_ n}{2} = 1 + \frac{1}{2} + \frac{1}{4} + \ldots + \frac{1}{2^ n} - ( \frac{1}{2} + \frac{1}{4} + \frac{1}{8} \ldots + \frac{1}{2^{n+1}} ) \]](/MI/d357264fda95670ebe35659fc87cfe54/images/img-0003.png) |
![\[ S_ n=2 - \frac{1}{2^ n}. \]](/MI/d357264fda95670ebe35659fc87cfe54/images/img-0004.png) |
 , it is easier to see what happens as we take partial sums with more and more terms, and we can calculate its limit as
, it is easier to see what happens as we take partial sums with more and more terms, and we can calculate its limit as  goes to infinity. As
goes to infinity. As  gets very large,
gets very large, 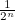 tends 0, so we calculate the limit as
tends 0, so we calculate the limit as ![\[ \lim _{n\to \infty } S_ n = \lim _{n\to \infty } 2 - \frac{1}{2^ n} = 2. \]](/MI/d357264fda95670ebe35659fc87cfe54/images/img-0007.png) |
A mathematician, like a painter or a poet, is a maker of patterns. If his patterns are more permanent than theirs, it is because they are made with ideas... The mathematician's patterns, like the painter's or the poet's, must be beautiful; the ideas, like the colours or the words, must fit together in a harmonious way... It may be very hard to define mathematical beauty, but that is just as true of beauty of any kind — we may not know quite what we mean by a beautiful poem, but that does not prevent us from recognizing one when we read it.
G. H. Hardy, "A Mathematician's Apology" pp 84-5
The content of the play was obviously mathematical, but even the structure of the play seemed to have a mathematical nature. Victoria Gould (who is the subject of this issue's career interview), a member of Complicite who is also a mathematician said: "We wanted to somehow embody the maths in the show, within the music and the rhythms and the space. We wanted it to be a mathematical experience as well as a theatrical one." The use of repetitions of the same scenes throughout the play, with each repetition giving more meaning, felt very much like the seeking of patterns that is an important element of mathematics. The play doesn't have a linear narrative. Instead it moves forwards and backwards through time and space, sometimes showing memories and past events superimposed on the present. Counting is used to evoke a feeling of moving forwards or backwards through time, and characters from different moments in history are positioned along a diagonal of the stage.

Shane Shambhu as Ramanujan and David Annan as Hardy in A disappearing number. Photo: Tristram Kenton.
It isn't surprising that it took a very unusual process to create such an unusual play. On and off over two years, the company played with the ideas and concepts to develop the show. They worked in a well equipped studio, the actors working with musicians, including Nitin Sawhney who provided the original music for the show, visual artists, sound designers, dancers, and mathematicians including Marcus du Sautoy. They explored the personal stories, as well as the mathematical concepts, and the images and emotions these all provoked.
The way that McBurney and Gould described this development process was very reminiscent of mathematical research. "What you get is an enormous amount of complete dross, but once in a while you know that something beautiful has happened," explained Gould. "When something is really right, and beautiful, you know. When Simon [McBurney] makes theatre, he is looking for that moment, when all the audience will come together." McBurney described mathematicians as being at the edge of what is knowable, and making the same kind of creative leaps of imagination as an artist. He recalled Hardy's own description of mathematical research: that mathematicians know when they are going in the right direction when what they are doing is beautiful.
The resulting play is a wonderful account of mathematical lives, real and imagined, and of creating mathematics. It puts some very sophisticated mathematical concepts onstage, bringing mathematics to an audience who otherwise might never have experienced it. But it also brings new perspectives to those with mathematical experience, including using mathematics as metaphor for the human condition.
For me the most inspiring and wonderful thing was seeing mathematics authentically portrayed in a dramatic setting. Mathematicians as real people, with real emotions. The mathematics was accurately conveyed, as was the mathematical passion and creativity that is vital to mathematical research. As someone who has always felt very passionate about maths, and that the creative moments of making mathematics are as exciting as any other creative discipline, it was fabulous to see this passion enacted on stage. Through the play, Complicite portrayed mathematicians that we could all identify with. If their vision of mathematicians can enter the popular imagination, maybe some day young people might be just as likely to daydream of becoming a mathematical hero, as any other.
Further reading
- You can find more about A disappearing number, including interviews and film clips on Complicite's web site
- Victoria Gould talks to Plus about developing the play in this issue's career interview
- And you can read more about Ramanujan and Hardy on the MacTutor History of Mathematics archive, or in Hardy's book A Mathematician's Apology (1940).
Rachel Thomas is co-editor of Plus.