
What is the area of the bird, shown below?
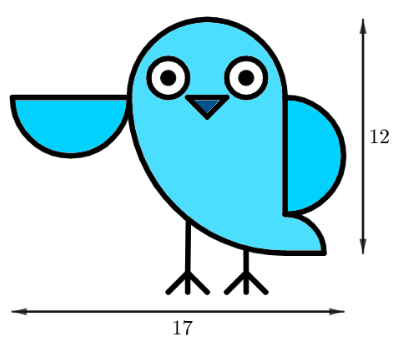
Hint: There are two equal semicircles representing the wings, one semicircle representing the head, one quarter of circle representing the body (with a radius equal to the diameter of the head) and one quarter of circle representing the tail.
Solution
Let
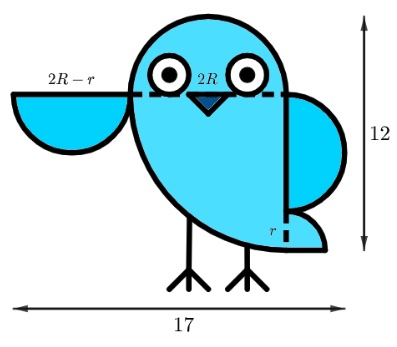
Then
Thanks to Paulo Ferro for this puzzle - you can find it in his new geometry puzzle book: Birds, Bees and Burgers. You can find out more about it at his new website EnigMaths.
Different answer
I love this! But I get a different answer (34pi). I get the radius of the wings to be 3 (you do too), hence area of 2 wings 9pi?
Thanks for pointing this out…
Thanks for pointing this out - we forgot a factor of 1/2! We have fixed it now.
Fun!
Fun!