
A day doesn't go by that we don't deal with knots. From tying a shoe in the morning or disentangling an extension cord to tying a ribbon around a present, knots are an integral part of our lives.
And they have been part of our lives for a very long time. At the beginning of civilization, knots would have been used to tie on rudimentary clothing, to secure weapons to the body, to create shelters. We have depended on them for thousands of years. But there is more to them than the physical applications that we take for granted.
Take a piece of string. Tie a knot in it, and then glue the two loose ends of the string together. You have now trapped the knot on the string. No matter how long you attempt to disentangle the string, you will never succeed without cutting the string open. The string models the mathematical concept of a knot.
![[IMAGE: tying a knot]](/issue15/features/knots/fig1.gif)
Fig 1: A mathematical knot
Given two such knotted loops of string, we will consider them equivalent if one can be rearranged to look like the other, again without allowing strands to pass through one another. The animation below shows an example.
Here are some of the most famous knots, all known to be inequivalent. In other words, none of these three can be rearranged to look like the others. However, proving this fact is difficult. This is where the mathematics comes in. The loop of string on the left is the unknot, also known as the trivial knot.
![[IMAGE: Three knots]](/issue15/features/knots/fig3.gif)
Fig 3: The trivial knot, the trefoil knot, and the figure of eight knot
The trefoil knot and the figure-eight knot are the two simplest nontrivial knots, the first having a picture with three crossings and the second with four. No other nontrivial knots can be drawn with so few crossings. Over the years, mathematicians have created tables of knots, all known to be distinct. Here is a random page from the knots of 10 crossings.
![[IMAGE: some 10-crossing knots]](/issue15/features/knots/fig4.gif)
Fig 4: Some of the distinct 10-crossing knots.
So far, over 1.7 million inequivalent knots with pictures of 16 or fewer crossings have been identified.
The mathematical theory of knots was born out of attempts to model the atom. Near the end of the nineteenth century, Lord Kelvin suggested that different atoms were actually different knots tied in the ether that was believed to permeate all of space. Physicists and mathematicians set to work making a table of distinct knots, believing they were making a table of the elements.
![[IMAGE: Knots and elements]](/issue15/features/knots/fig5.gif)
Fig 5: Do different knots correspond to different elements?
In 1887, the Michelson-Morley experiment demonstrated there was no such ether, and the physicists and chemists lost interest in knots for the next century. But for mathematicians, it was too late. They were hooked on the intrinsic beauty of the field and the mathematics that it engenders, and the theoretical aspects of knots continued to be developed.
In the 1980s, chemists and biochemists again became intrigued with the theory of knots. One of the important applications is to the molecule deoxyribonucleic acid (DNA). DNA consists of millions of atoms that together form a blueprint for life. Although it is in the shape of a double helix, we can think of it as a very long thin string packed into the nucleus of a cell, like 200 kilometers of fishing line tangled up inside a soccer ball. Yet it must be able to perform various functions such as recombination, transcription and replication. The tangling can prevent these operations from being able to occur. It turns out that there are enzymes in the nucleus that will perform knot theoretic operations on the DNA molecule that allow it to knot and unknot. Although we will not go further into this fascinating area of research here, biochemists and mathematicians are collaborating on attempts to figure out how various enzymes act.
Chemists are also very interested in creating knotted molecules. One can easily picture a so-called "cyclic" molecule. This is a molecule formed by a sequence of atoms bonded end-to-end. Then the last atom bonds with the first, forming a looped molecule as on the left in Figure 6. Benzene is a good example of such a molecule.
Now imagine constructing a molecule in exactly the same manner, with the same set of constituent atoms bonded in exactly the same order, only this time, before bonding the last atom to the first, a knot is tied in the molecular strand. After the ends are bonded together, we have a knotted molecule.
![[IMAGE: molecules]](/issue15/features/knots/fig6.gif)
Fig 6: A cyclic molecule and a knotted molecule with the same constituent atoms.
Whereas the unknotted version may behave like an oil, the knotted version may behave like a gel. It may have completely different properties, properties never before seen in a molecule. In fact, for each of the distinct knots in the tables of knots, we obtain a distinct substance from this same chain of atoms.
Since there are infinitely many possible distinct knots, it appears that a single sequence of atoms bonded in a certain order can generate an infinite number of different molecules, one for each of the different knots in the table of knots. Of course, that's not quite the case. If we have a sequence of 10 atoms, we will not be able to create a knot of 50 crossings. There will be some upper limit on the complexity of the knots constructible with this many atoms.
The first question one might ask is, "What is the smallest number of atoms needed to construct a nontrivially knotted molecule?"
Let's model each atom by a point in space, called a vertex, and each bond between atoms by a stick between the corresponding vertices. Then a cyclic molecule, knotted or not, is modelled by a sequence of sticks glued end-to-end so that the last one is also glued to the first.
![[IMAGE: stick knot]](/issue15/features/knots/fig7.gif)
Fig 7: A stick version of a knotted molecule.
We then ask the related question, "How many sticks does it take to construct a nontrivial knot?" Of course, we may not be able to make a knotted molecule with this number of atoms. There are additional constraints such as bond angles, and lengths of bonds. But the answer to this question should at least give us a minimum number of atoms we will need to construct a knotted molecule.
So let's figure out how many sticks are needed to make a nontrivial knot. One or two sticks aren't enough to make a loop, knotted or otherwise. Three sticks can form a triangle, but since a triangle lies in a plane, there will be no crossings and it will be a trivial knot.
![[IMAGE: 3-stick cycle]](/issue15/features/knots/fig8.gif)
Fig 8: Three sticks will not suffice to make a nontrivial knot.
What about four sticks? If we draw pictures with four sticks, we quickly see (and can prove) that there are only two possibilities. Either the sticks don't cross each other at all, in which case the knot is trivial, or the sticks can have one crossing, in which case, the knot can be rearranged to see that it is trivial.
![[IMAGE: 4-stick cycles]](/issue15/features/knots/fig9.gif)
Fig 9: Pictures of knots constructed from four sticks.
How about five sticks? Well, let's suppose that we could make a nontrivial knot out of five sticks glued end-to-end. Let's imagine drawing a picture of this supposed knot, but it will be a very particular picture. We will look straight down one of the sticks and draw the picture of the resulting knot. The stick that we look down will appear in the picture as just a single vertex, since we are looking straight down it. So in our picture, we will only see the four remaining sticks. Therefore our picture will look exactly like one of the ones in Figure 9 above, and again, our knot will be trivial. (How about that for a nice argument?) What about six sticks? Here is a picture of a trefoil knot constructed with six sticks:
![[IMAGE: 6-stick trefoil]](/issue15/features/knots/fig10.gif)
Fig 10: A trefoil knot made from six sticks.
The picture by itself is not enough to demonstrate that the knot could actually be constructed in space with straight sticks in this pattern. It might be that one of the sticks bends in the plane perpendicular to the picture, but we can't see that. However, the labels on the picture are there to convince us it can be constructed with straight sticks. The vertices labelled with P are to be thought of as lying in the plane of the screen. The vertices labelled B are in a plane behind the screen, and those labelled F are in front of the screen. One could actually choose coordinates in 3-dimensional space and then show that the edges are in the correct relationship with one another in order to prove that this model of the trefoil is realizable in 3-dimensional space.
So we can realize a nontrivial knot, in this case, the trefoil knot, with six sticks. How close to this theoretical limit have chemists come, when constructing molecular knots? So far, the simplest knotted molecule constructed appears in Figure 9, its first synthesis dating from the year 2000. So clearly we are nowhere near the theoretical limit yet.
![[IMAGE: knotted molecule]](/issue15/features/knots/fig11.gif)
Fig 11: The simplest knotted molecule so far.
Of course, synthesizing knotted molecules is a tricky business. It is simple enough to tie a knot in a piece of string. But imagine trying to replicate that process on the molecular level. That is no small feat.
One would like to answer the corresponding question for any particular knot K. How many sticks does it take to construct K? We call the least number of sticks for a knot K the stick number of K, denoted s(K). Translated back to chemistry, this is relevant to the question of how long a molecular chain is necessary to construct a knotted molecule in the shape of a particular knot.
Here is a picture of a torus knot, which wraps around the surface of a doughnut (whose mathematical shape is called a torus). We can count the number of times it wraps the long way, denoted by p, and the number of times it wraps around the short way, denoted q. We say such a knot is a (p,q)-torus knot.
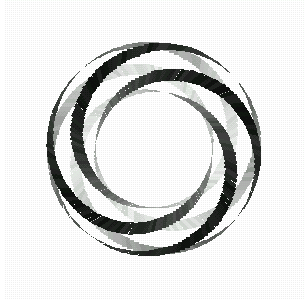
Fig 12: The (p,q) - torus knot.

Fig 13: The trefoil as a torus knot.
In 1994, three students and I proved that the stick number of the (q,q-1)-torus knot was exactly 2q. Since the trefoil knot is a (q,q-1)-torus knot for q =3, we obtain 6 for the number of sticks to construct it, as we expected. This formula extends the example of the trefoil to an infinite set of examples.
As sometimes happens in mathematics, this result was independently proved by Gyo Taek Jin, a Korean mathematican, who found the stick number of an even larger class of torus knots. The idea for showing that a (q,q-1)-torus knot can be constructed from 2q sticks was to model the torus by two truncated hyperboloids, one skinny and one wide.
![[IMAGE: truncated hyperboloids]](/issue15/features/knots/fig14.gif)
Fig 14: A torus can be modelled by two truncated hyperboloids.
Hyperboloids are ruled surfaces, meaning they can be covered entirely by disjoint lines. Choosing the right q line segments lying on each truncated hyperboloid allows us to construct the (q,q-1)-torus knot.
The second part of the argument is to show that the knots cannot be constructed with fewer sticks. Although too technical to get into here, we used the total curvature of knots to demonstrate this, while Gyo Taek Jin used something called the superbridge number of a knot.
Many questions about stick number remain open. Here are two particular ones.
1. How many sticks does it take to make a 2-braid knot (see Figure 15)?
![[IMAGE: 2-braid knot]](/issue15/features/knots/fig15.gif)
Fig 15: A 2-braid knot.
The conjecture is c+3, where c is the number of crossings. That certainly works for the trefoil. It's not too hard to show that any 2-braid of c crossings can be constructed with c+3 sticks. (Try it!) But one needs to show that none of them can be constructed with a smaller number of sticks. That's where the going gets tough.
2.Can the stick number of a knot change if you only allow equal length sticks?
An answer to either of these questions would be of interest to mathematicians and chemists alike.
As the mathematical theory of knots develops, their usefulness will continue to expand. Hidden within these simple objects is an amazing amount of mathematics, with essential implications for physics, chemistry, biology and the other sciences. It is not difficult to answer the question, "why knot?"
About the author

Colin Adams is the Francis Christopher Oakley Third Century Professor of Mathematics at Williams College, Massachusetts. He is particularly interested in the mathematical theory of knots and their applications. He is the author of the Knot Book, an elementary introduction to the mathematical theory of knots, co-author of the humorous supplements to calculus "How to Ace Calculus" and "How to Ace the Rest of Calculus" and the author of a regular mathematical humour column called "Mathematically Bent" in the Mathematical Intelligencer.
Comments
"demonstrated there was no such ether"
It's popular to bash the ether theory because nobody really remembers anymore why they came up with it. Actually it's brilliant, and the fact that people are going back to the idea that atoms are knotted flows is a good indication that we should re-interpret what the ether MIGHT be rather than simply throwing out the idea because the analogy doesn't hold in literally every way. All the MM experiment shows is that the ether is frame-relative and not fixed absolutely in space like a literal fluid. And why would it be? I don't know, but people seem quick to reject the model based on this completely arbitrary feature.