Add new comment

New designs from Africa

Mar 2002

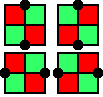
Inspiration from Angolan traditional designs

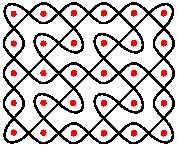
Since the middle of the 1980's I have been analysing mathematical properties of traditional ideo- and pictograms of the Tchokwe population in Angola. These drawings were traditionally done in sand, and were used to illustrate stories the men told at evening gatherings, around the communal fires in the village or at hunting camps. Young boys would learn the easier stories and accompanying designs, but only the storytellers, who were highly esteemed for their knowledge, and formed an elite, knew many more.

Sadly, colonialism and the slave trade caused most knowledge of the patterns to be lost. Some missionaries and ethnographers saved a few hundreds of them from oblivion, collecting designs and the accompanying stories to keep their knowledge alive.

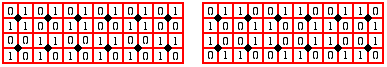
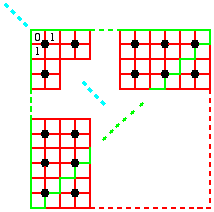
Above is an example of a typical Tchokwe pictogram. It belongs to a very particular class of curves that I call "mirror curves". I discovered that these curves share interesting mathematical properties, and decided to define the class of designs they generate as "Lunda-designs". The Tchokwe are descendants of the Lunda people, and live predominantly in a region today called Lunda, hence the name Lunda-design to honour my source of inspiration.

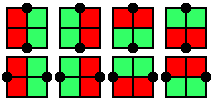
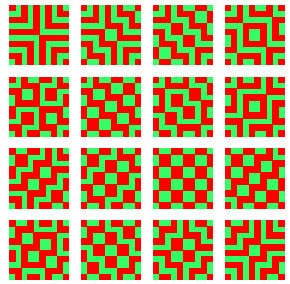
The picture below presents examples of Lunda-designs. Do you find them appealing? Why?

Do you note something particular about these two-colour designs? Do they have some common characteristic?

You are invited to return to these questions at the end of this article. Before trying to answer more general questions, mathematicians - and not only mathematicians - like to consider more specific questions in order to get some feeling for what is happening. In this article we will reflect about a special class of Lunda-designs.

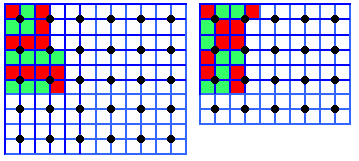
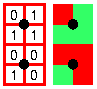
On the day before my daughter Likilisa's fourth birthday I started to analyse one particular class of Lunda-designs. As these designs turned out to have some interesting properties I gave them the name of Liki-designs. To the left are two examples.

How may we characterise a Liki-design? To get an idea try to copy one of the Liki-designs on squared paper in such a way that each unit square has only one colour. What can be said about the distribution of the unit squares of each colour?

Some examples of Liki-designs

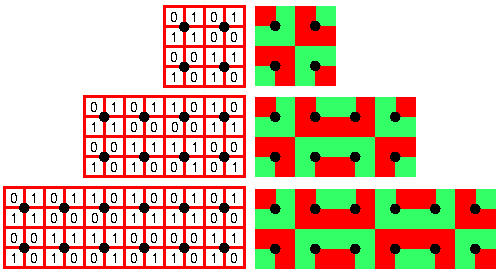
Liki-designs may be presented in alternative ways, each of which is shown in turn below:

as a two-coloured design, which, for convenience, may be drawn on squared paper with each unit square completely covered with one of the two colours;
as such a coloured rectangle to which grid points are added;
as a matrix obtained by substituting the red unit squares coloured by the number 0 and the green ones by the number 1.

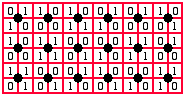The distance between any grid point and the rectangular border is one unit of measurement (equal to the length of the side of a unit square) and the distance between two horizontal or vertical neighbouring grid points is two units. In the example above there are 3 rows of 5 grid points each. We will call the related design a 3x5 Liki-design. The corresponding Liki-matrix has dimensions 6x10 (6 rows and 10 columns).

Observe the total number of 0's in each column of the 3x5 Liki-design in this example. Do you notice anything particular? What about the total number of 1's in each column? Can you say anything about the total number of 0's and 1's in each row?

Observe pairs of horizontal or vertical neighbouring grid points in this example. What can be said about the distribution of red and green unit squares in the middle between the two grid points?

What can be said about any two unit squares simultaneously adjacent to the border and to a grid point?

The defining characteristics of Liki-designs

Examining the example described above, you can see that in each column and row the 0's and 1's are in balance. This implies also that the total number of 0's in the matrix is equal to the total number of 1's. This is a general property of a Liki-design: the two colours are in balance. As well as this global symmetry, there are local symmetries of two types:

Each grid point along the border has a red (=0) square on one side and a green (=1) square on the other.
Of the four unit squares between any two neighbouring grid points, two neighbouring unit squares are always red (=0), while the other two are green (=1).

These two local symmetry rules are the defining characteristics of Liki-designs, and imply the equality of the total numbers of red and green squares.

Consider the designs below. If possible, try to complete them in such a way that they become Liki-designs. Are there many ways to complete them? Why?

How many Liki-designs are there?

How many different mxn Liki-designs can be built up?

In order to avoid counting two designs as the same when actually you can turn one into the other just by swapping the roles of the two colours, we'll take the first number in the first row of the corresponding matrix to be 0. Let L(m,n) denote the total number of mxn Liki-designs.

Try to build all possible 4x2 Liki-designs. Are you sure you found them all?
Construct all 3x3 Liki-designs.
Try to determine L(m,n). To do this, you could try to produce tables successively for L(1,n), L(2,n), L(3,n) and L(4,n), and conjecture thereafter a general formula for L(m,n).

We'll try to find L(2,n) as an example.

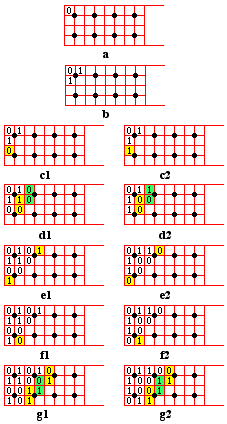
Consider the open grid rectangle in labelled (a), with a 0 in the top left corner. By the first symmetry rule, both the horizontal and vertical neighbour unit squares have to contain a 1, as shown in (b). There are too few numbers filled in for us to be able to apply either of the construction rules. So we are free to choose the first number in the third row to be either 0 or 1. These two possibilities are labelled (c).

By twice applying rule (2), we obtain the two possible situations labelled (d). At the left and top borders we can apply rule (1) and complete a triangular array of numbers (labelled (e)).

Having only two rows of grid points at our disposal, we have already arrived at the bottom border, and can apply the first symmetry rule once again (labelled (f)), and immediately apply the second rule three times (labelled (g)). Once again we can apply rule (1) at the top and bottom border and with rule (2) fill in a diagonal array of numbers, and so on.

Below are shown two possible matrix situations after several further iterations.

In order to get Liki-designs we now have to decide at what points it is possible to introduce a border on the right side.

In the first situation, this is not possible after one grid point, as it would lead to a matrix that does not satisfy rule (1) at the right border, as shown on the right.

The same happens after any odd number of grid points. However, it is possible to introduce a border after any even number of grid points, as the diagram below shows for n=2, 4, and 6.

In the second situation, the right border can be introduced after any number of grid points. Below are the cases n=1, 2, and 3.

So what can we say about L(2,n)? If n is even there are two Liki-designs (one of each type); if n is odd, there is only one Liki-design (of the second type). Table 1 presents this result in an alternative way.

n	1	2	3	4	5	6	7	8	9	10
L(2,n)	1	2	1	2	1	2	1	2	1	2

Analogously, the following relationships can be established:

n	1	2	3	4	5	6	7	8	9	10
L(3,n)	1	1	4	1	1	4	1	1	4	1

n	1	2	3	4	5	6	7	8	9	10
L(4,n)	1	2	1	8	1	2	1	8	1	2

We can present these results together in a single table:

			n
	L(m,n)	1	2	3	4	5	6	7	8	9	10
m	1	1	1	1	1	1	1	1	1	1	1
	2	1	2	1	2	1	2	1	2	1	2
	3	1	1	4	1	1	4	1	1	4	1
	4	1	2	1	8	1	2	1	8	1	2
	5
	6
	7
	8
	9
	10

How to fill in this table further? Obviously the table should be symmetrical and we may try to guess what values will appear on the principal diagonal: 1, 2, 4, 8, 16, 32, 64, and so on.

			n
	L(m,n)	1	2	3	4	5	6	7	8	9	10
m	1	1	1	1	1	1	1	1	1	1	1
	2	1	2	1	2	1	2	1	2	1	2
	3	1	1	4	1	1	4	1	1	4	1
	4	1	2	1	8	1	2	1	8	1	2
	5	1	1	1	1	16
	6	1	2	4	2		32
	7	1	1	1	1			64
	8	1	2	1	8				128
	9	1	1	4	1					256
	10	1	2	1	2						512

Observing this incomplete table, we may conjecture that L(m,n) is always equal to 1 if m and n do not have common divisors greater than 1. Examining the values in the table we might guess that the greatest common divisor of m and n plays an important role.

We may conjecture that

L(m,n) = 2^{gcd (m,n) -1},

where gcd(m,n) denotes the greatest common divisor of m and n.

The reader is invited to prove this conjecture. The method of mathematical induction may be very useful. We will also use induction in the following section.

Square Liki-designs

Since gcd(n,n) = n, the number of square Liki-designs L(n,n) is equal to 2 ^n-1.

Construct all 3x3 Liki-designs or, equivalently all 3x3 Liki-matrices. Don't forget that the matrices will have the dimensions 6x6.
Construct all 4x4 Liki-designs.
What properties do these square Liki-designs share?
If you know the concept of the determinant of a square matrix, you may calculate the determinants of the 3x3 and 4x4 Liki-matrices. Do you notice anything?

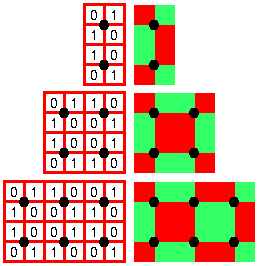
Here are the sixteen 5x5 Liki-designs. They all have two diagonal axes of symmetry. Can you see why this has to be so?

Let us try to prove more generally the theorem that nxn Liki-designs and Liki-matrices display diagonal axes of symmetry. We will use induction.

Theorem

nxn Liki-designs and Liki-matrices display diagonal axes of symmetry.

Proof:

In the case n=1, there is only one square Liki-design, shown on the left, and it has two diagonal axes of symmetry.

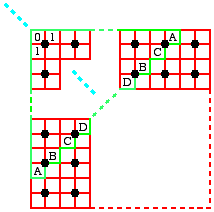
Let us suppose that for m less than n, all mxm Liki-designs have two diagonal axes of symmetry. Let us consider an nxn Liki-matrix. As the triangular array at its top left corner may appear in building up a (n-1)x(n-1) Liki-matrix, this array must be symmetrical, by the induction hypothesis.

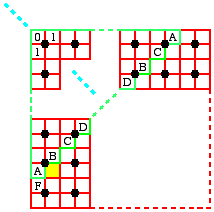
Now let us consider the first number F, either 0 or 1, in the last row but one of the nxn Liki-matrix. By construction rule (2), the first number to its upper right, immediately below the "toothed" side of the triangular array, has to be 1 if F=0, or 0 if F=1.

When we climb further up along the "toothed" side of the triangular array until we arrive at the top border, the numbers continue to alternate 0, 1, 0, 1, and so on. As the total number of unit squares situated between oblique rows of grid points is odd when going from the left border to the top border, the oblique row of 0's and 1's is either of the type 0,1,0,..,0,1,0 or of the type 1,0,1,..,1,0,1.

In both cases, the number sequence is symmetrical.

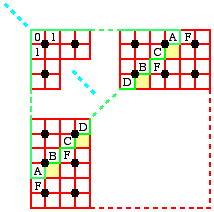
In other words, not only is the initial triangular array symmetric, but so is the expanded triangular array that includes this symmetric oblique row of 0's and 1's.

Now let us analyse what happens with the next oblique row, going from the bottom left corner to the top right corner of the nxn Liki-matrix. The first number of this oblique row is equal to the last number, as both are equal to 1-F by rule (1).

Now consider pairs of consecutive unit squares located between diagonally neighbouring grid points along this oblique row. Let us take any pair and let P and Q be the numbers inscribed in them. On the other side of the axis there exists a corresponding pair, shown to the right. Let S and T be the numbers inscribed in them. What can be said about their neighbouring numbers that belong to the expanded triangular array?

G and H of the initial triangular array correspond to H and G, as the initial array is symmetric in agreement with the induction hypothesis. The numbers of the expanded triangular array are in both cases F, 1-F, and F as the expanded array is symmetric as already shown. By construction rule (2), we have, on the one side

P = 2 -(F + G + (1-F)) = 1-G

and

Q = 2-(F+H+(1-F)) = 1-H.

On the other side

S = 2 -(F + G + (1-F)) = 1-G

and

T = 2-(F+H+(1-F)) = 1-H.

In other words, we find that P=S and Q=T. As this happens with all corresponding pairs, the central oblique row of numbers is symmetric. As a consequence, the triangular array obtained by expanding the initial triangular array successively with two oblique rows is symmetric.

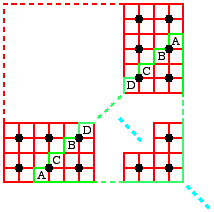
As for the triangular array at the left top of the nxn Liki-matrix, we can analyse the triangular array at its bottom right (as below), and conclude that the principal diagonal of the nxn Liki-matrix is a symmetry axis. Analogously the second diagonal is also a symmetry axis.

In this way we have completed our proof.

Some Liki-designs also have horizontal and vertical axes of symmetry. Liki-designs of this type can be used to build up interesting magic squares.

Liki Magic squares

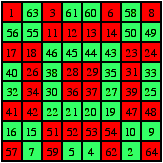
Let us construct a number square starting with the 4x4 Liki-design shown below, using the following construction rule:

Write the numbers 1 through 64 in the unit squares from the left to the right, from the top row to the bottom row.

The set of numbers written inside the green unit squares is rotated through 180^o about the centre of the Liki-design. The resulting number square is a magic square, as the sum of the numbers in any row or column, (or along the principal diagonal) is equal to 260.

Construct other 4x4 Liki magic squares.
Is it possible to use 5x5 Liki-designs in the same way to to construct magic squares?
Construct 6x6 Liki-designs with horizontal and vertical symmetry axes. Use them to build up magic squares.
Will it be possible to construct a 7x7 Liki-design with horizontal and vertical axes of symmetry?
Construct 8x8 Liki-designs with horizontal and vertical symmetry axes. Use them to build up magic squares.
Try to formulate a theorem about Liki-designs and the generation of magic squares. Try to prove the theorem.
If you know the concept of the determinant of a square matrix, you may calculate the determinants of some of the magic squares you constructed. Do you notice anything?

Lunda-designs

As observed in the introduction, Liki-designs constitute a particular class of Lunda-designs. In order to construct Lunda-designs, the first construction rule is the same as for Liki-designs:

(1) Along the border each grid point has always one red (=0) and one green (=1) unit square associated with it.

The second construction rule for Lunda-designs is a little more general than the respective rule for Liki-designs.

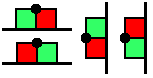
The second rule for Liki-designs was the following:

(2) Of the four unit squares between two arbitrary (vertical or horizontal) neighbouring grid points, two neighbouring unit squares are always red (=0), while the other two are green (=1),

for Lunda-designs it is:

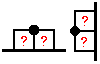
(2') Of the four unit squares between two arbitrary (vertical or horizontal) neighbouring grid points, two are red (=0), and two are green (=1) (this means that situations like the ones shown on the right are admitted too).

Try to construct some Lunda-designs.
Try to discover some properties of Lunda-designs.

Plus.Maths.org