Add new comment

101 uses of a quadratic equation

March 2004

It isn't often that a mathematical equation makes the national press, far less popular radio, or most astonishingly of all, is the subject of a debate in the UK parliament. However, in 2003 the good old quadratic equation, which we all learned about in school, was all of those things.

Where we begin

It all started at a meeting of the National Union of Teachers. The quadratic equation was held aloft to the nation as an example of the cruel torture inflicted by mathematicians on poor unsuspecting school children. Intrigued by this accusation, the quadratic equation accepted a starring role on prime time radio where it was questioned by a formidable interviewer more used to taking on the Prime Minister. The (London) Times took space in its leader column, more usually reserved for weighty discussions on the moral (or otherwise) health of the modern world, to proclaim that the quadratic equation was useless, maths was useless and that no one wanted to study maths anyway, so why bother. Concerned lest dangerous admissions by the quadratic equation remain unchallenged, the vital importance of the equation to the survival of the UK was debated (a positive view was taken, you may be glad to know) in the British House of Commons.

Where would it all end? Was the quadratic equation really dead? Did anyone care? Are mathematicians really evil monsters who only want to inflict quadratic equations on a younger generation as a means of corrupting their immortal souls?

Maybe so, but it's not really the quadratic equation's fault. In fact, the quadratic equation has played a pivotal part in not only the whole of human civilisation as we know it, but in the possible detection of other alien civilisations and even such vital modern activities as watching satellite television. What else, apart from the nature of divine revelation, could be considered to have had such an impact on life as we know it? Indeed, in a very real sense, quadratic equations can save your life.

The Babylonians

Babylonian cuneiform tablets recording the 9 times tables

It all started around 3000 BC with the Babylonians. They were one of the world's first civilisations, and came up with some great ideas like agriculture, irrigation and writing. They plotted the paths of the Sun, the Moon and the planets, and recorded them on clay tablets (which you can still see in the British Museum). To the Babylonians we owe the modern ideas of angle, including the way that the circle is divided up into 360 degrees (owing to a small miscalculation, one per day). We also owe the Babylonians for the rather less pleasant invention of the (dreaded) taxman. And this was one of the reasons that the Babylonians needed to solve quadratic equations.

Let's suppose that you are a Babylonian farmer. Somewhere on your farm you have a square field on which you grow some crop. What amount of your crop can you grow on the field? Double the length of each side of the field and you find that you can grow four times as much of the crop as before. The reason for this is that the amount of the crop that you can grow is proportional to the area of the field, which is in turn proportional to the square of the length of the side. In mathematical terms, if $x$ is the length of the side of the field, $m$ is the amount of crop you can grow on a square field of sidelength 1, and $c$ is the amount of crop that you can grow, then

$c = m x^2.$

This is our first quadratic equation, naked and blinking in the sunlight. Quadratic equations and areas are linked together like brothers and sisters in the same family. However, at the moment we don't have to solve anything - until the tax man arrives, that is! Cheerily he says to the farmer "I want you to give me $c$ crops to pay for the taxes on your farm." The farmer now has a dilemma: how big a field does he need to grow that amount of crop? We can answer this question easily, in fact

$x = \sqrt{ \frac{c}{m} }.$

Finding square roots by using a calculator is easy for us, but was more of a problem for the Babylonians. In fact they developed a method of successive approximation to the answer which is identical to the algorithm (called the Newton-Raphson method) used by modern computers to solve much harder problems than quadratic equations.

Now, not all fields are square. Let's now suppose that the farmer has a more oddly shaped field with two triangular sections as shown on the right.

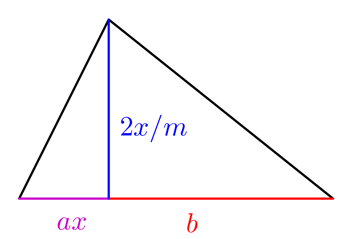
For appropriate values of $a$ and $b$ the amount of crop that the farmer can grow in this field is given by

$c = a x^2 + b x.$

This looks a lot more like the quadratic equation that we are used to, and even under the evil eye of the tax man, it’s a lot harder to solve. Yet the Babylonians came up with the answer again. First we divide by $a$ to give

$x^2 + \frac{b}{a} x = \frac{c}{a}.$

Now we complete the square by using the fact that

$\left( x + \frac{b}{2a} \right)^2 = x^2 + \frac{b}{a} x + \frac{b^2}{4a^2}.$

Combining this with the original equation we have

$\left( x + \frac{b}{2a} \right)^2 = \frac{c}{a} + \frac{b^2}{4a^2}.$

This is now an equation that we can solve by taking square roots. The result is the famous " $-b$ formula":

$x = -\frac{b}{2a} \pm \sqrt{\frac{c}{a} + \frac{b^2}{4a^2}},$

which can be rewritten as

$x = \frac{-b \pm \sqrt{b^2 + 4ac}}{2a}.$

(The formula usually has "-4ac" because the quadratic equation is more usually written in the form " $ax^2+bx+c=0$ ".)

The fact that taking a square root can give a positive or a negative answer leads to the remarkable result that a quadratic equation has two solutions. So much for mathematical puzzles only having one solution!

Now, this is where the teaching of quadratic equations often stops. We have reached that object beloved of all journalists when they interview mathematicians - a formula. Endless questions can be made up which involve putting values of $a,b$ and $c$ into the formula to give (two) answers. But this isn’t what mathematics is about at all. Finding a formula is only the first step on a long road. We have to ask, what does the formula mean; what does it tell us about the universe; does having a formula really matter? Let’s now see where this formula will take us.

A surprise for the Greeks, a bit of mathematical origami and a sense of proportion

We now fastforward 1000 years to the Ancient Greeks and see what they made of quadratic equations. The Greeks were superb mathematicians and discovered much of the mathematics we still use today. One of the equations they were interested in solving was the (simple) quadratic equation

$x^2 = 2.$

They knew that this equation had a solution. In fact it is the length of the hypotenuse of a right angled triangle which had sides of length one.

It follows from Pythagoras’ theorem that if a right-angled triangle has shorter sides $a$ and $b$ and hypotenuse $c$ then

$a^2 + b^2 = c^2.$

Putting $a=b=1$ and $x = c$ then $x^2 = 2$ . Thus $x = \sqrt{2}.$

So, what is $x$ in this case? Or, to ask the question that the Greeks asked, what sort of number is it? The reason that this mattered lay in the Greek’s sense of proportion. They believed that all numbers were in proportion with each other. To be precise, this meant that all numbers were fractions of the form $a/b$ where $a$ and $b$ are whole numbers. Numbers like 1/2, 3/4 and 355/113 are all examples of fractions. It was natural to expect that $\sqrt{2}$ was also a fraction. The huge surprise was that it isn’t. In fact

$\sqrt{2} = 1.4142135623730950488 \ldots ,$

where the dots $\ldots$ mean that the decimal expansion of $\sqrt{2}$ continues to infinity without any discernible pattern. (We will meet this situation again later when we learn about chaos.)

$\sqrt{2}$ was the first irrational number (that is, a number which is not a fraction, or rational), to be recognised as such. Other examples include $\sqrt{3}$ , $\pi$ , $e$ and in fact "most" numbers. It took until the 19th century before we had a good way of thinking about these numbers. The discovery that $\sqrt{2}$ was not a rational number caused both great excitement (100 oxen were sacrificed as a result) and great shock, with the discoverer having to commit suicide. (Let this be an awful warning to the mathematically keen!) At this point the Greeks gave up algebra and turned to geometry.

Far from being an obscure number, we meet $\sqrt{2}$ regularly: whenever we use a piece of A4 paper. In Europe, paper sizes are measured in A sizes, with A0 being the largest with an area of $1 m^2$ . The A sizes have a special relationship between them. If we now do a bit of origami, taking a sheet of A1 paper and then folding it in half (along its longest side), we get A2 paper. Folding it in half again gives A3, and again gives A4 etc. However, the paper is designed so that the proportions of each of the A sizes is the same - that is, each piece of paper has the same shape.

We can pose the question of what proportion this is. Start with a piece of paper with sides x and y with x the longest side. Now divide this in two to give another piece of paper with sides y and x/2 with now y being the longest side. This is illustrated to the right.

The proportions of the first piece of paper are $x/y$ and those of the second are $y/(x/2)$ or $2y/x$ . We want these two proportions to be equal. This means that

$\frac{x}{y} = \frac{2y}{x},$

$\left( \frac{x}{y} \right)^2 = 2.$

Another quadratic equation! Fortunately it's one we have already met. Solving it we find that

$\frac{x}{y} = \sqrt{2}.$

This result is easy for you to check. Just take a sheet of A4 (or A3 or A5) paper and measure the sides. We can also work out the size of each sheet. The area $A$ of a piece of A0 paper is given by

$A = xy = x \left( \frac{x}{\sqrt{2}} \right) = \frac{x^2}{\sqrt{2}}.$

But we know that $A=1 m^2$ so we have another quadratic equation for the longest side $x$ of A0, given by

$x^2 = \sqrt{2} m^2 \quad \mbox{or} \quad x = \sqrt{\sqrt{2}} m = 1.189207115 \ldots m.$

This means that the longest side of A $2$ is given by $x/2 = 59.46 cm$ (why?) and that of A $4$ by $x/4 = 29.7cm.$ . Check these on your own sheets of paper.

Paper used in the United States, called foolscap, has a different proportion. To see why, we return to the Greeks and another quadratic equation. Having caused such grief, the quadratic equation redeems itself in the search for the perfect proportions: a search that continues today in the design of film sets, and can be seen in many aspects of nature.

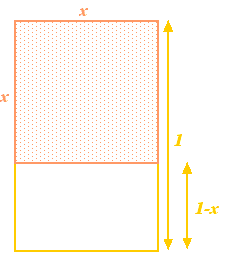
Let’s start with a rectangle, and then remove a square from it with the same side length as the shortest side of the rectangle. If the longest side of the rectangle has length 1 and the shortest side has length $x$ , then the square has sides of length $x$ . Removing it from the rectangle gives a smaller rectangle with longest side $x$ and smallest side $1-x$ . So far, so abstract. However, the Greeks believed that the rectangle which had the most aesthetic proportions (the so called Golden Rectangle) was that for which the large and the small rectangles constructed above have the same proportions. For this to be possible we must have

$\frac{x}{1} = \frac{1-x}{x} \quad \mbox{or} \quad x^2 + x = 1.$

This is yet another quadratic equation: a very important one that comes up in all sort of applications. It has the (positive) solution

$x = \frac{\sqrt{5}-1}{2} = 0.61803 \ldots .$

The number $x$ is called the golden ratio and is often denoted by the Greek letter $\phi$ .

The Golden Rectangle can be seen in the shape of windows, especially on Georgian houses. More recently, the Golden Ratio can also be found as the "perfect shape" for photographs and film images. The quadratic equation $x^2 + x = 1$ also arises in studies of the populations of rabbits and in the pattern in which the seeds of sunflowers and the leaves on the stems of plants are arranged. These are all linked with the Golden ratio through the Fibonacci sequence which is given by

$1,1,2,3,5,8,13,21,34,55,89,144,\ldots$

Sunflower seeds, arranged using Fibonacci numbers

The Parthenon, embodying the Golden Ratio

In this sequence each term is the sum of the previous two terms. Fibonacci discovered it in the 15th century in an attempt to predict the future population of rabbits. If you take the ratio of each term to the one after it, you get the sequence of numbers

$\frac{1}{1}, \frac{1}{2}, \frac{2}{3}, \frac{3}{5}, \frac{5}{8}, \frac{8}{13},\ldots \quad \mbox{or}\quad 1, 0.5, 0.66667, 0.6, 0.625, 0.61538, \ldots$

and these numbers get closer and closer to (you guessed it) the Golden Ratio $\phi$ .

By finding both of the roots of the above quadratic equation we can actually find a formula for the nth term in the Fibonacci sequence. If $F_ n$ is the $n$ th such number with $F_0 = 1$ and $F_1 = 1$ then $F_ n$ is given by the formula

$F_ n = \frac{(1/\phi )^ n + \phi ^2 (-\phi )^ n }{1 + \phi ^2}.$

Conics link quadratic equations to the stars

The Greeks were also very interested in the shape of cones. the picture on the left shows a typical cone.

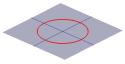
Half of the cone can be visualised as the spread of light coming from a torch. Now, if you shine a torch onto a flat surface such as a wall then you will see various shapes as you move the torch around. These shapes are called conic sections and are the curves that you obtain if you take a slice through a cone at various different angles. Precisely these curves were studied by the Greeks, and they recognised that there were basically four types of conic section. If you take a horizontal section through the cone then you get a circle. A section at a small angle to the horizontal gives you an ellipse. If you take a vertical section then you get a hyperbola and if you take a section parallel to one side of the cone then you get a parabola. These curves are illustrated below.



A cross-section of a cone can be a circle ...	... an ellipse ...	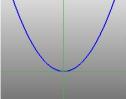... a parabola ...	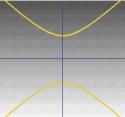... or a hyperbola.

Conic sections come into our story because each of them is described by a quadratic equation. In particular, if $(x,y)$ represents a point on each curve, then a quadratic equation links $x$ and $y$ . We have:

The circle: $x^2 + y^2 = 1$ ;

The ellipse: $a x^2 + b y^2 = 1$ ;

The hyperbola: $ax^2 - by^2 = 1$ ;

The parabola: $a x^2 = y.$

These curves were known and studied since the Greeks, but apart from the circle they did not seem to have any practical application. However, as we shall see in the next issue of Plus, a link between quadratic equations and conics, coupled with a mighty lucky fluke, led to an understanding of the way that the universe worked, and in the 16th century the time came for conics to change the world.

About the authors

Chris Budd is Professor of Applied Mathematics in the Department of Mathematical Sciences at the University of Bath, and the Chair of Mathematics at the Royal Institution in London.

Chris Sangwin is a member of staff in the School of Mathematics and Statistics at the University of Birmingham. He is a Research Fellow in the Learning and Teaching Support Network centre for Mathematics, Statistics, and Operational Research.

They have recently written the popular mathematics book Mathematics Galore!, published by Oxford University Press.

This article was inspired in part by a remarkable debate in the British House of Commons on the subject of quadratic equations. The record of this debate can be found in Hansard, United Kingdom House of Commons, 26 June 2003, Columns 1259-1269, 2003, which is available online at the House of Commons Hansard Debate website.