Outer space: Where to stand to look at statues
The Sphinx, at Giza (Image by Usuario:Barcex CC-BY-SA-3.0)
Many of the great cities of the world offer fine statues to be admired from a distance. Usually, they stand on pedestals or are affixed to facades far above our heads. Where should we stand to get the best possible view of them? If we stand very close we find ourselves looking almost vertically upwards and capture only a small cross-section of the front. So we need to be further away, but how much further? As we go away from the statue on its pedestal it appears smaller and smaller; closer and it looks steadily larger until we get too close and it starts to look smaller again. In between there must be an optimal viewing distance, in the sense that the statue appears at its biggest.
We can find this distance by setting up the viewing scenario as in the figure below. You, or rather your eyes, are located at 
; the height of the statue’s pedestal is 
above your eyes and the height of the statue on top of it is 
. These distances are fixed but we can change 
, the distance you stand from the pillar.
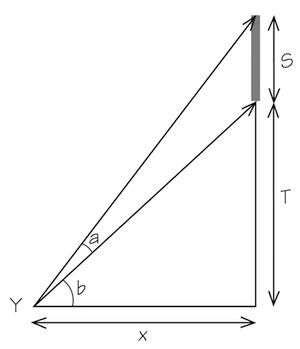
A little geometry helps. The tangents of the angles 
and 
between the ground and the top of the pillar and the ground and the top of the statue, respectively, are given by:
![\[ \tan (a+b) = \frac{(S+T)}{x} \mbox{ and } \tan (b) = \frac{T}{x} \]](/MI/d96d28d6166d6b0017b2cdc739763754/images/img-0003.png) |
![\[ \tan (a+b) = \frac{\tan (a) + \tan (b)}{1 - \tan (a)\tan (b)} \]](/MI/d96d28d6166d6b0017b2cdc739763754/images/img-0004.png) |
![\[ \tan (a) = \frac{Sx}{x^2 + T(S+T)} \]](/MI/d96d28d6166d6b0017b2cdc739763754/images/img-0005.png) |
for which the angle 
spanning the height of the statue is maximised. To do this we just work out the differential 
and set it equal to zero. (This differential is the rate of change of the angle with the distance from the statue . You can find out more about calculating these in Making the grade.) Differentiating, we have: ![\[ \sec ^2(a)\frac{da}{dx} = \frac{-S(x^2 - T(S+T))}{(x^2 + T(S+T))^2} \]](/MI/ca5bdaf9f14f3a696550053f227b882f/images/img-0001.png) |
is a square it is positive for a values in between 0 and 90 degrees; we see that 
and the statue looks biggest when the numerator on the right-hand side is zero – that is, when: ![\[ x^2 = T(S+T) \]](/MI/ca5bdaf9f14f3a696550053f227b882f/images/img-0004.png) |
Nelson on his column (Image by Eluveitie CC-BY-SA-3.0)
This is the answer to our question. The optimal viewing distance 
that makes the facade of the statue look the largest is given by the geometric mean of the altitude of the pedestal and the altitude of the top of the statue, or 
.
We can try this formula out for some well-known monuments (we'll assume that a person's eyeline is 1.5m above the base of the pedestal in these examples). Nelson’s Column in Trafalgar Square in London has 
m above eye level, roughly, with 
m so you should stand about 
m away to get the best view. The Sphinx, at Giza just south of the Pyramid of Khafre, has 
m and 
m so the optimal viewing distance is at 52.6m. Michelangelo’s statue of David in Florence has 
m and 
m so we need step back only to 
m for the best view. If you want to prepare for your visit to view one of the world’s great statues on a pedestal then you can find the dimensions you need for calculating the optimal viewing point on helpful websites.
About the author
John D. Barrow is Professor of Mathematical Sciences at the University of Cambridge, author of many popular science books and director of the Millennium Mathematics Project of which Plus is a part.
This article is an extract from Barrow's new book 100 essential things you didn't know you didn't know about maths and the arts. You can hear John talk about his new book, and help him celebrate its launch with a drink after, at 6pm on Thursday 13 November at Gresham College. See their website for more information.