Last week a team of 18 US and European mathematicians and computer scientists managed to tame one of the wildest beasts of mathematics: the exceptional Lie group E8. After four years of work, the team's supercomputer produced a description of E8 that, if it were written out on paper, would cover an area the size of Manhattan. It comprises 60 gigabytes, enough to store 45 days' worth of continuous music in MP3 format. For comparison, the human genome, which contains all the genetic information of a cell, is less than a gigabyte in size.

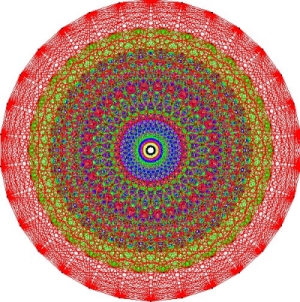
This intricate diagram describes some of the structure of E8. It is a two-dimensional representation of an 8-dimensional object called the Gosset polytope 4₂₁ and was generated on a computer by John Stembridge, based on a hand drawing by Peter McMullen. The image was taken from the website of the American Institute of Mathematics.

Lie groups encode one of the most fundamental phenomena we humans perceive in the world around us: symmetry. Symmetry not only occurs in visual objects like geometric shapes or patterns. Mathematical objects like equations, physical systems and even the laws of the universe can exhibit forms of symmetry and spotting these is essential in our understanding of them. "This is an exciting breakthrough," said Peter Sarnak of Princeton University and the American Institute of Mathematics, "Understanding ... Lie groups has been critical to understanding phenomena in many different areas of mathematics and science including algebra, geometry, number theory, physics and chemistry. This project will be invaluable for future mathematicians and scientists."

But what is a Lie group? If you look at a geometric shape like a square, you'll find that certain transformations of the plane leave it unchanged. Rotations around the central point of the square through 90, 180 or 270 degrees leave the square looking as it did to start with, as do reflections in the diagonals of the square and the axes connecting the midpoints of opposite sides. Transformations like these are called symmetries of the square and together they form neat algebraic systems. Follow one rotation by another, for example, and the result is also a rotation. The effect of one rotation can always be reversed by performing another rotation; for example, the effect of rotating the square clockwise through 90 degrees can be reversed by rotating anti-clockwise through 90 degree (or, equivalently, rotating clockwise through 270 degrees). Once you are in the land of rotational symmetries, you'll never get out again by rotations alone and you can reverse every step you take within it.

Collections of objects that behave like this (and satisfy a couple of additional rules) are what mathematicians call groups. Every geometric object comes with symmetries that combine together in groups. Some objects, though, are more symmetric than others. A circle, for example, can be rotated around its centre through any angle without changing its appearance. In this case, the symmetries involved are said to be continuous: if you labelled each rotational symmetry by its angle, then the labels would line up and merge into a continuous line. This isn't the case for a square; here the labels would give just four isolated points on the line: 90, 180, 270 and 360. Informally, a Lie group is a group of continuous symmetries. Lie groups are named after the 19th century Norwegian mathematician Sophus Lie.

Lie groups are also associated with higher-dimensional objects; in three dimensions the sphere obviously gives rise to continuous groups of symmetries, as do other round objects like the cylinder or the cone. There are infinitely many different Lie groups and most of them are well-understood, except for a collection of exceptional Lie groups. Of these, the group E8 is the most complicated. The geometric object it is associated with has 57 dimensions.

The new breakthrough concerns an annoying and devilishly difficult problem in group theory: that one group can appear in many different guises. Groups can consist of all sorts of objects, not just symmetries. Numbers, functions and other mathematical objects can also form groups. To express everything in a common language, mathematicians use objects called matrices. They assign a matrix to each group element so that the matrices interact in the same way as the corresponding group elements do.

As an example, consider again a circle and all its rotational symmetries. To a rotation r_θ around the centre of the circle and through angle θ you can associate the matrix $$ \left( \begin{array} {cc}cos(\theta) & sin(\theta) \\ -sin(\theta) & cos(\theta)\end{array} \right).$$ This is a very natural thing to do: if you know some linear algebra, you will know that a matrix of this form, when viewed as a transformation of the plane, describes exactly a rotation through angle θ around the point (0,0). If you now multiply the matrix associated to the rotation r_θ by that associated to the rotation r_φ, you will find that the result is precisely the matrix associated to the rotation you get when you follow r_θ by r_φ — the matrices interact in exactly the same way as the rotations do.

However, you could just as well have decided to associate to a rotation r_θ the matrix $$ \left( \begin{array}{cc} cos(2\theta) & sin(2\theta) \\ -sin(2\theta) & cos(2\theta)\end{array} \right).$$ Again, you would find that the matrices interact in the same way as the rotations do. This new rule gives just as valid a representation of the group in terms of matrices as the first one. And this is what representation theory is all about: pinning down all the different matrix representations of a group, so that you can recognise it when you come across it.

In this case spotting that the two matrices represent the same group is relatively easy, but the more complicated the group, the harder the problem becomes. In the case of E8 there are infinitely many representations, though using a trick mathematicians managed to reduce the number of those they needed to describe the group to 453,060. This is still a huge number and, on the face of it, a vast amount of equations would have to be solved to crack the group. The team had already resigned themselves to waiting for the next generation of powerful computers to arrive on the scene, when Noam Elkies of Harvard University came up with an ingenious trick to reduce the number of calculations needed. The team's supercomputer finally pinned down E8 in only 77 hours.

The E8 calculation is part of an ambitious project known as the Atlas of Lie groups and representations. These are some of its members. The image is taken from the website of the American Institute of Mathematics.

While pure mathematicians celebrate the final clinching of E8 for reasons of abstraction and beauty, theoretical physicists are just as excited. For decades they have been trying to come up with a unifying theory of everything that can describe every aspect of our universe. String theory is a prime candidate here and the group E8 plays a major role in the subject. "While mathematicians have known for a long time about the beauty and the uniqueness of E8, we physicists have come to appreciate its exceptional role only more recently," says Hermann Nicolai, Director of the Albert Einstein Institute in Potsdam, Germany, "This is an impressive achievement."

Notable also is the way in which the result was achieved: as an active and continuous collaboration between, as far as maths goes, a relatively large group of researchers. As Brian Conrey, Executive Director of the American Institute of Mathematics, put it, "People will look back on this project as a significant landmark and because of this breakthrough, mathematics is now a team sport."

Further reading

• You can find more detail on the E8 calculation on the American Institute of Mathematics website.
• For more on group theory, read Plus articles The power of groups and Through the looking glass.
• There is more on symmetry and the role it plays in our universe in Plus article Symmetry rules.
• For an introduction to string theory read Plus article Tying it all up.