
Heavens above
One of the great triumphs of nineteenth century physics was to enable us to go beyond the bounds of our ordinary experience. It was the culmination of Newton's realisation that the laws which govern what happens here on earth are the same as the laws that govern the way the planets move. Before Newton, people thought there were entirely different rules governing the heavens and the more mundane region "down here". The fact that the law of gravity that applied near us also applied "up there" gave us a means of extrapolating our experience down here to up there.
Conic sections
The law that Newton discovered was the inverse square law of gravitation - a simple law, but one with great mathematical beauty. The solutions to the equations describing the motions produced by this law are called conic sections - ellipses, hyperbolae and parabolae - which you get by intersecting a plane and a cone. One of the observations you can make about
physical laws of nature is that the ones which apply over wide ranges tend to have a deep mathematical structure. This is certainly true of conic sections - they occur in many different areas of mathematics, and are in that sense deep, as well as mathematically beautiful.
By the nineteenth century people could say a lot about what was happening "up there". They also started to be able to say something about what was happening at very small scales. The laws governing electricity and magnetism, which were put into a unified form with a coherent structure by James Clark Maxwell about the middle of the nineteenth century, were found to apply to things as small as atoms. One of the things that really surprised Paul Dirac when he started to learn about physics in some detail was that you could use the laws of electromagnetism, which were discovered by studying the objects around us, to say things about how atoms are structured.
Don't use your common sense

Where will it go?
freeimages.co.uk
Common sense tells you that if you throw a ball you can work out where it goes. In other words, if you know the position and the velocity of the ball, then, using the laws of mechanics, you can work out what will happen to it. We are used to a world in which you can use basic equations to calculate the trajectory of a ball you throw. You might think - and for a long time people did think - that the atom should be the same. When it was discovered that the atom consisted of a nucleus - a concentrated centre with a positive electrical charge - and tiny negative electrical charges - electrons - moving around it, people tried to think about atoms using the picture they had of everyday objects.
But this commonsense approach ran into problems. The picture of electrons moving around the atomic nucleus, obeying the same laws of attraction as the planets moving around the sun, didn't fit the facts. For example, if the ordinary laws of mechanics governed what happened inside atoms, we would expect atoms to be unstable, because when electrons move, they accelerate, and so they would radiate energy and spiral into the nucleus. Also, if that were the correct picture, then electrons could be in any orbit, just as the planets can be in any orbit around the sun. But then we wouldn't see the characteristic atomic spectra of the different elements - atoms would be able to produce light of any colour, which is not what we observe.
The answer to the conundrum is that it is not correct to assume that what happens at the scales at which we live, also happens at the atomic scale. We have to undergo the reverse process to the one Newton underwent, when he said that what happened in the heavens was the same as what happened on earth. Forces can be the same in very different domains - and this realisation was very productive in Newton's day - but it's not inevitable that our common sense should still be accurate when we think about what happens at very tiny scales.
Fantastic voyage
Imagine you could shrink yourself till you were tiny enough to fit inside an atom. Wouldn't the electrons look to you like little balls, travelling around the nucleus in the same way that planets orbit the sun? Would they have to behave the same way? This seems like a valid thought experiment - but actually it isn't. People are made of lots and lots of atoms, and atoms have a certain scale,
which makes it intrinsically impossible that a person should be inside an atom! So, although the way things behave inside the atom doesn't match what we imagine a little person living inside an atom would see, this doesn't violate common sense. Shrinking down to smaller than an atom is a fantastic voyage no person could take - not even in imagination.

Particular place, particular speed
freeimages.co.uk
By the time Dirac started working on quantum mechanics in 1923, people had already understood that the mechanics of the atom had to be very different from the mechanics of objects on the scale in which we live. Soon they realised you could not say that at some particular time an electron is in some particular place and moving at some particular speed. Of course we are accustomed to being able to make such statements about the objects we deal with every day - "there's a car outside the house going at 50 miles an hour down the road" - but totally different notions are needed to describe what happens at very tiny scales.
These descriptions were found by studying the mathematical relationships between properties inferred from the results of experiments. One of the things that was clear from experiments was that electron have spin. A first naive picture of an electron - this is not an accurate picture but it's a start - is as a tiny ball with electric charge - which is what flows when a current flows in a wire. If you spin a ball of electric charge, the electric charge goes around in a circle. You effectively have a tiny current going around, and when you have a current like that you have a magnetic field - the electron becomes a tiny magnet. The presence of that magnetic effect is pictured as the electric charge spinning around. If the electron was still, it wouldn't have this magnetic effect.
Spin was first observed in an experiment of Stern and Gerlach in 1921, where electrons were passed through a magnetic field and it was found that they deviated, some of them one way and some of them the other. This suggested that the electron had two ways of spinning, called "spin up" and "spin down". You might have expected that there would be a whole range of ways of spinning - that the electron's spin could be anything you liked. But then there would not just be two places the electron could go, but a whole range of places, and that wasn't what happened in the experiment. There was this particular pair of values for the spin - it was either one way or the other.

Paul Dirac. Portrait by R. Tollast, 1963; reproduced by permission of the Master and Fellows of St. John's College, Cambridge
That spin was manifested not only by the fact that the electrons acted as tiny magnets, but also by the fact that they had angular momentum. If you sit on a typist's chair and make yourself spin round with your arms straight out, and then pull your arms in to yourself, you make yourself go faster. The reason for this is that when your arms are further out, parts of you are moving faster. The further those bits are out from the centre the faster they're moving. When something moves in a circle like that we say it has angular momentum. If you bring your arms in, they're no longer moving so fast, but the amount of angular momentum stays the same, and so the chair goes round faster and faster.
Now the angular momentum of a system is conserved - if particles interact, by colliding, for example, their combined angular momentum stays the same. This fact was used to design experiments, in which it became clear that electrons have innate angular momentum.
So observationally, some of the properties of the electron were known, but, until Dirac's famous electron equation, there didn't seem to be any particular reason for them. He wanted to find a way to describe the quantum mechanics of the electron - mechanics where you didn't know in general where something was and how fast it was moving. This requirement led to a particular sort of equation, a wave equation.
Quantum Relativity
The other major development of twentieth century physics was Einstein's publication of his special theory of relativity in 1905. This describes how mechanics changes when things move very fast. Again, the intuition that we have from moving at everyday speeds leads us astray. Compared to the way things behave at the subatomic scale, things differ less drastically from what we intuitively expect. It's really very drastic not to be able to say both what position something is in and what velocity it's got! But it's much less difficult to think about the differences caused by special relativity.
One thing special relativity tells us is that we can't all agree on what the time is. The time in New York is five hours behind the time in London, and so on, but that's not the point. We could all agree to use Greenwich Mean Time wherever we were. It would mean that some of us would be getting up at midnight, but the time we get up is just a convention! But what Einstein told us was that if one person sits with a watch in Greenwich and someone else zooms off very fast and then comes back to Greenwich, then their watches won't agree. That's intrinsic - there's no way round it. But it isn't such a drastic idea, it's just something you get used to. If you've been zooming off on a spaceship and when you get back your clock is a few tiny fractions of a second different, it doesn't change the whole way you think about things. Special relativity is less anti-intuitive than quantum mechanics because a person can't get inside an atom, but can get inside a rocketship. Travelling very fast wouldn't change the whole nature of their existence, and therefore it contradicts common sense less.
The problem then was how to reconcile relativity with quantum mechanics, because although the particles inside the atom are not moving very close to the speed of light, they are moving sufficiently fast that you can measure differences from what would happen without relativistic effects. So physicists needed to know how to make quantum mechanics - for an electron, say - fully compatible with Einstein's theories. Dirac went through a process - for him a very logical process - of working out how you should describe an electron, and that led to his equation.
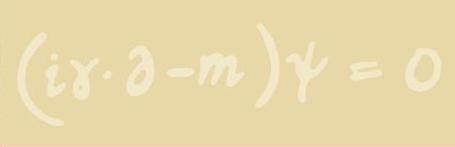
Dirac's electron wave equation
The point about this equation, when you understand what it means, is that it tells you that an electron has a spin and a magnetic moment and exactly what they should be. So it not only gives you a consistent description, but it does so in a way that inevitably points out that the electron must have a spin and a magnetic moment. It also predicted the existence of another particle, which was first thought to be an "electron with negative energy". When these anomalies were reinterpreted, physicists realised that there must be a completely new particle which had never before been seen. We now call that particle the positron, and it was the first example of antimatter. There's virtually no antimatter around, because as soon as it comes into contact with matter it is annihilated and produces energy, so you only get it under special circumstances, but now it is routinely created and used - for example in PET (Positron Emission Tomography).
In a spin - twice
Among the many counterintuitive properties of the electron is the fact that it has spin one-half. This is the mathematical way of saying that if you rotate an electron through 360 degrees, it doesn't look like it did before you started! There is no parallel for this in our everyday world - we are accustomed to being able to turn objects through 360 degrees and get them back to where they started. With an electron, you have to turn through 360 degrees a second time to get back to the original state.When you rotate an electron through 360 degrees, something more complicated happens than that things just look the exact same. There is a sort of degrees of freedom aspect associated with the spin, and they turn around as well. And what happens with spin one half is that they don't turn all the way back to where they started, they turn back to minus where they started. So that if you rotate through 360 degrees you're not back to where you started, you're back in the absolutely opposite space in terms of the degrees of freedom associated with the spin. You have to go through 360 degrees again to get back to your original state.
A quantum leap for computers

The way things are now
freeimages.co.uk
An ordinary computer is of course a piece of electronic equipment, which means it works by utilising electrons. But the bits of information you are manipulating via these electronics are ordinary numbers, represented in binary form. The information is contained in a sequence of 0's and 1's in base 2. This is classical information, something we are all used to dealing with.
In a quantum computer what you want to do is use quantum information. Quantum information is "what state is the electron in?". In the Stern-Gerlach experiment, as the electron moved through the magnetic field it was either spinning up or down and it went into one spot or the other, into one of two places. But that's an example of a quantum mechanical process, because when you put a given electron in, in general it won't be spinning up or down, it will be spinning in some other state - a quantum superposition of up and down. When the electron is subject to a measuring process, such as "we are going to look and see which of two places it ends up in" it somehow chooses to go one place or the other. But the initial state of the electron before it has made the choice is more complicated than just "up" or "down". Saying "up" or "down" is like saying "0" or "1".
Representing a series of 0's and 1's
freeimages.co.uk
If the electron had to be up or down to begin with and we had a number of electrons, they could be used to represent a series of 0's and 1's - in other words, they could be used to represent classical information, ordinary numbers. But, in fact, the electron is in this more subtle state which is neither up nor down, but which will choose to be one or the other with a certain probability when forced to do so. A sequence of electrons in these superposed states gives you much more subtle information - quantum information. If you can manipulate electrons but avoid forcing them to make this choice, you can manipulate more information than in a classical computer - and if you can do it at the same sort of speed, you can calculate far more.
One implication of this is that it may become possible to factorise very large numbers. If I give you an enormous number and I tell you it is the product of two prime numbers, it will be very difficult to find them using the techniques we know now. It will take you an impracticably long time however powerful your computer is. That principle is used to encode and decode information. But if you could factor large numbers then you could break these codes. A quantum computer - if you could build one in practice - would make a lot of things possible that aren't possible now. Keeping information secret would become highly challenging.
Quantum mechanics has turned out to be stranger than anyone could have predicted - and certainly stranger than nineteenth century physicists would have believed. Although the concepts involved seem to run counter to any reasonable understanding, quantum processes are essential to much modern technology, from medical imaging to electronic computing. Quantum computers would be another enormous
leap forward - with unpredictable consequences. Perhaps it would be easiest for us all if that particular can of worms was never opened, but we can't just say, "well I'd rather not know", because somebody else might find out first!
About the author
Peter Goddard is Master of St John's College and Professor of Theoretical Physics in the University of Cambridge. His contributions to research, which have centred on the development of String Theory, have been recognised by his election as a Fellow of the Royal Society (1989), the award of the Dirac Prize and Medal of the International Center for Theoretical Physics, Trieste, (1997) and appointment as CBE (2002).He has also been instrumental in the development of programmes aimed at widening access to the University of Cambridge, and encouraging ambition and attainment in school students in less advantaged areas of the country, including St John's College's successful EAGLE project in Lambeth. He currently chairs the University of Cambridge Joint Committee on Admissions and the group concerned with co-ordinating initiatives in the University aimed at widening access and raising aspirations.