
"Mechanics is the paradise of the mathematical sciences because by means of it one comes to the fruits of mathematics."
Leonardo da Vinci

William Leybourn, (1626-1719). Image courtesy National Oceanic & Atmospheric Administration, photographed by Sean Linehan.
The inspiration for this article comes from a slightly unusual source: The Proceedings of the Old Bailey from April 18th 1694. On this particular day 29 death sentences were passed at the court house, as well as numerous orders for brandings; there would have been 30 death sentences had not one lady's pregnancy saved her — she had successfully "pleaded her belly". The business part of the document ends with a list of the 29 unfortunates and continues to another list; this time of advertisements. Surprising though it may seem, advertisements for anything from quack remedies to religious texts formed an important part of the court proceedings between the 1680s and the 1750s. On April 18th 1694 the second item on the list was an advert for a book:
Pleasure with Profit: Consisting of Recreations of divers kinds, viz. Numerical, Geometrical, Mathematical, Astronomical, Arithmetical, Cryptographical, Magnetical, Authentical, Chymical, and Historical. Published to Recreate Ingenious Spirit, and to induce them to make further scrutiny how these (and the like) Sublime Sciences. And to divert them from following such Vices, to which Youth (in this Age) are so much inclin'd. By William Laybourn, Philomathes.
If the author of these lines is to be believed, then those who were tried in the docks that day may well have been spared their gruesome fate, had they only been given access to this work. We will touch on only a small part of it ourselves: to be precise, pages 12 and 13.
A detail of page 12 of Pleasure with Profit.
William Leybourn (1626-1719) (alias Oliver Wallingby) was in his time a distinguished land and quantity surveyor (although he began his working life as a printer). Such was his prestige, he was frequently employed to survey the estates of gentlemen, and he also helped to survey the remnants of London after the great fire of 1666. A prolific and eclectic author, his work The Compleat Surveyor, which was first published in 1653 and ran to five editions, is regarded as a classic of its kind and (in collaboration with one Vincent Wing) the 1649 volume Urania Practica, was the first book in English devoted to astronomy.
In 1694 he had published the recreational volume Pleasure with Profit. It contained a delightful mechanical puzzle, attributed to one "J.P.", which has become known as the Uphill Roller.
Pages 12 and 13 of the book detail the construction of a double cone and two inclined rails along which the cone can roll — uphill! His final paragraph explains the paradox: even though the cone does ascend the slope, its centre of gravity will actually move downwards, if the experiment is set up in the right way. Although one's senses might be confounded, the law of gravity is not.
This animation shows a virtual uphill roller in action. In the animation below you can see the downward movement of the centre of gravity of the double cone.
Before we examine his explanation, we will look at the matter through modern eyes, using elementary trigonometry. The experiment consists of two inclined rails and a double cone. The cone will roll "uphill" resting on the top surfaces of the two rails.
Figure 1 shows a vertical section of the set-up. The inclined line represents the top surfaces of the rails. The bottom end of the slope, shown at the left-hand side of figure 1, is at a distance a from the ground. The top end of the slope, on the right-hand side of figure 1, is at a distance b from the ground. The figure also shows the cross-section of the double cone after it has rolled some way up the slope. The point in the middle of this cross section is the double cone's centre of gravity, which we call G. The angle of inclination of the rails is
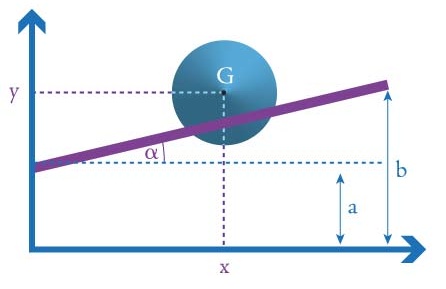
Figure 1
Figure 2 shows the view from the front, in other words from the point where the two rails meet. We see the cone as if it had been sliced vertically and lengthwise. Again we see the centre of gravity G, as well as the points P and Q where the top surfaces of the two rails meet the double cone. The points G1, P1 and Q1 are the projections of these three points to the ground. The angle
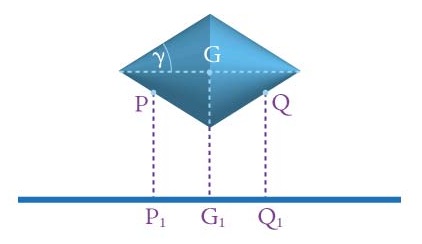
Figure 2
Figure 3 shows the projection of the whole set-up to the floor. The diamond shape is the shadow the double cone casts on the floor and the two lines emanating from the points P1 and Q1 are the shadows cast by the two rails. They meet at the point O and at an angle 2β. As before, the points G1, P1 and Q1 are the projections of G, P and Q.

Figure 3
With the coordinate system from figure 1 in place we can find the equation of the path of the centre of gravity of the double cone as it rolls up the slope. For convenience we write XY for the straight line segment running from a point X to a point Y.
First consider figure 3. The distance between O and G1 is precisely the x-coordinate of the point G. Using basic trigonometry we have that
Since P1Q1 = PQ we get
Now let's take the front view from figure 2. Write S for the point that lies on the line from G to G1 at the same height as P and write R for the apex of the cone which lies on the line from G to G1. See figure 4.

Figure 4
Then
Now the height y of G is SG1 + SG. For the second part we have SG = r-RS, where 2r is the thickness of the double cone at its centre (see figure 4). So
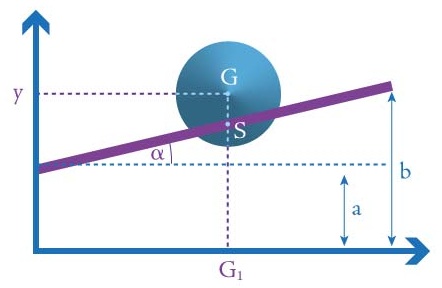
Figure 5
Therefore we have
The path of the centre of gravity of the cone is, then, the straight line
Of course, to properly appreciate the paradox a physical model is needed. The author's model (made by his long-term friend Brian Caswell) has:
An excerpt from Leybourn's recipe for an uphill roller
The things necessary for this Experiment are, First, A Roller of Wood [...] of two Cones (or Sugar Loaves). Let the thickness in the middle be about 5 or 6 Inches, the length [of the cone] about 3 times the thickness. [...] You must provide two straight smooth Rulers about a Yard in length, and strong enough to bear the weight of the Roller. Lastly, you must have three pieces of Wood to support the ends of the rulers; the first about two or three inches thick [to support the rulers at the bottom of the slope]; the other two [to support the rulers at the top of the slope] must be thicker than the first by somewhat less than half the diameter of the ruler.
Leybourn's recipe
Now that we have a clear criterion to use, we can look more closely at Leybourn's instructions for building an uphill roller (which we've reproduced on the right) and check that they really do give rise to angles that satisfy the required relationships. The instructions specify that each of the cones that make up the double cone should be between 5 and 6 inches in thickness, so taking the upper limit of 6 we get r=3. The length of each of the two cones is to be three times its thickness, so it is 9. The rails are to be one yard in length, and since one yard is 36 inches, the length of the slope is 36.
The blocks supporting the end of the slope should be thicker than those supporting the beginning of the slope by "somewhat less" than half the diameter of the cone, in other words by less than r (as marked in the left-hand diagram in figure 6 below). We can choose the thicknesses of the blocks so that their difference is less than, but very close to r. Then the difference in height between the bottom of the slope and the top of the slope, b - a, is roughly equal to r.
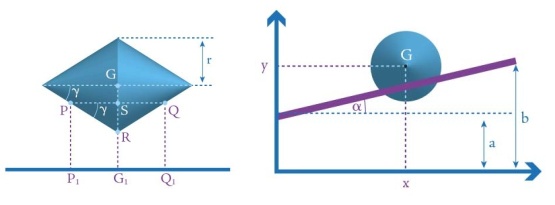
Figure 6
From this and the right-hand diagram in figure 6, we now deduce that

Figure 7
Now let's turn to the angle β. The right hand diagram in figure 6 shows that the sloped rail and the dotted horizontal line form a right angled triangle with an angle
At the end of the cone's journey, the point P1, shown in figure 7, will be at the left-hand apex of the double cone, and its distance to G will be the length of one cone, which is 9. This gives

Figure 8
From figure 8 we get that
In summary, Leybourn's instructions reduce to:
With the mystery of the uphill roller explained we will leave the reader with the following sentiment expressed in the book, with which we whole-heartedly agree:
But leaving those of the Body, I shall proceed to such Recreations as adorn the Mind; of which those of the Mathematicks are inferior to none.
About the author

Comments
Defying gravity: The uphill roller
There is a precise article in the European Physics Journal by S. Gandhi and C. Efthimiou about the uphill roller. The reference is: "The Ascending double cone: a closer look at a familiar demonstration", volume 26 in 2005, pages 681-694. The authors have looked at several details (some of which I do not understand since I am still in high school).
Thanks
Thanks to the author for such a simple explanation. The historical background has made it more enriched with the fact that human mind for very long has been seeking to explain all the actions with a proper scientific reasons. Such a mathematician (the author) definitely can bring the interested young minds to the fold of wonderful world of mathematics.