
In this article we present a set of unusual dice and a two-player game in which you will always have the advantage. You can even teach your opponent how the game works, yet still win again! Finally, we will describe a new game for three players in which you can potentially beat both opponents — at the same time!
Our two-player game involves two dice, but they're not the ordinary dice we're used to. Instead of displaying the values 1 to 6, each die has only two values, distributed as follows:
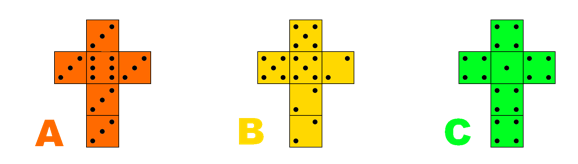
| Die A: | 3 | 3 | 3 | 3 | 3 | 6 | |
| Die B: | 2 | 2 | 2 | 5 | 5 | 5 | |
| Die C: | 1 | 4 | 4 | 4 | 4 | 4 |
This is how the game works: each player picks a die and the two players then roll their respective dice at the same time. Whoever gets the highest value wins. Seems fair enough — but is it?
It can be shown (see below) that in the long run die A beats die B (it'll win against B more often than it'll lose against B), and that die B beats die C. So it appears A is the strong die and C is the weak die. So you might expect that, in the long run, die A beats die C:
If this were the case, then we'd call the dice transitive, as the winning property transfers via die B in the middle.
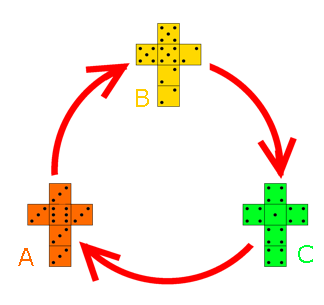
But it is not the case! In fact, the winning property goes round in a circle — like in a game of Rock, Paper, Scissors — with die A beating die B, die B beating die C, and die C beating die A (in the long run). There is no strong or weak die; the dice are what's called non-transitive. But how can this be?
Winning chances
Let's see why die A beats die B in the long run.
When you roll die A there are two possible outcomes; you either roll a 3 or a 6. The probability of rolling a 3 is 5/6, while the probability of rolling a 6 is 1/6. On the other hand, die B can either roll a 2 or a 5, each with a probability of 1/2. So in total, if we roll die A and die B together, we have four possible outcomes, as illustrated in the following tree diagram.

We find the probability of each possible outcome by multiplying the probabilities along the diagram. For example, the probability of rolling a 5 with die A and a 2 with die B is 5/6 x 1/2 = 5/12.
To find the probability that die A wins, add up the probabilities of all the outcomes where die A beats die B. So in this case, the probability die A beats die B (for which we write P(A>B)) is 5/12+1/12+1/12 = 7/12 — importantly, this is more than 1/2. So in a contest of several games die A is expected to win more often than die B.
In the same way it can be shown that in the long run die B beats die C, and then remarkably die C beats die A. (P(B>C)=7/12 and P(C>A)=25/36.) So, as long as your opponent picks first, you will always be able to pick a die with a better chance of winning. The average winning probability is (7/12 + 7/12 +25/36)/3 = 67/36, which is around 62%. It turns out that these three dice give a maximal set in the sense that they maximise the winning probabilities. But that's not the only surprising thing about them.
Double Whammy
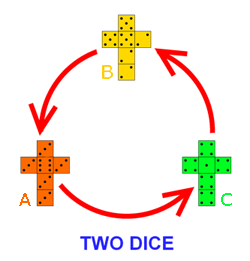
After a few defeats your opponent might become suspicious, so it's time to come clean and explain that you're dealing with non-transitive dice. You can give your opponent another chance, offering to pick your die first, so they should be able to pick a die with a better chance of winning. You can even offer a change in the game: each of you now rolls two of their chosen type of die. Surely, this means that your opponent has just doubled their chances of winning?
Not so! Amazingly, with two dice the order of the chain flips.
The chain reverses so the circle of victory now becomes a circle of defeat. Now, die A beats die C, die C beats die B, and die B beats die A, allowing you to win the game again!
The average winning probability with two dice is around 57%. The full probabilities are:
P(A > C) = 671/1296,
P(C > B) = 85/144,
P(B > A) = 85/144.
A word of warning though: although the probability that die A beats die C is greater than 1/2, it is a slim victory. In the short term, say less than 20 rolls, the effect is closer to 50-50, so you will still need some luck on your side.
Efron dice
The idea of non-transitive dice has been around since the early 1970s. However, the remarkable reversing property is not true for all sets of non-transitive dice, for you do need to pick your values carefully. For example, here is another famous set of non-transitive dice, invented by the American statistician Brad Efron:
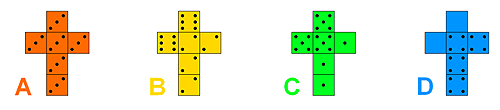
This time the dice use values 0 to 6. Each die has values:
| Die A: | 3 | 3 | 3 | 3 | 3 | 3 |
| Die B: | 2 | 2 | 2 | 2 | 6 | 6 |
| Die C: | 1 | 1 | 1 | 5 | 5 | 5 |
| Die D: | 0 | 0 | 4 | 4 | 4 | 4 |
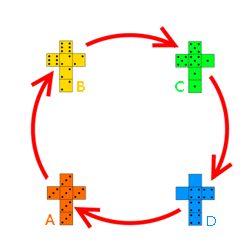
As before, the dice form a circle with die A beating die B, die B beating die C, die C beating die D, and die D beating die A, and they each do so with a probability of 2/3.
We also have two pairs of dice opposing each other on the circle. In fact, die B beats die D, but die A and die C each have a 50-50 chance of winning, with neither die dominating.
Unfortunately, the player picking die A will not have a very exciting game, with all the values being the number 3. Also, this chain does not display the remarkable property of flipping when you double the number of dice. The probabilities for two sets of Efron dice are:
P(A > D) = 5/9,
P(B > A) = 5/9,
P(B > C) = 5/9,
P(C > D) = 5/9,
P(B > D) = 11/27,
P(D > B) = 0,
P(B = D) = 16/27 (a draw),
P(A > C) = 1/4,
P(C > A) = 1/4,
P(A = C) = 1/2.
The American investor Warren Buffett is reported to be a fan of non-transitive dice. When he challenged his friend Bill Gates to a game, with a set of Efron dice, Bill became suspicious and insisted Warren choose first. Maybe if Warren had chosen a set with a reversing property he could have beaten Gates — he would just need to announce if they were playing a one die or two dice version of the game after they had both chosen.
Three-player games
I wanted to know if there is a three player game, a set of dice where two of your friends may pick a die each, yet you can pick a die that has a better chance of beating either opponents — at the same time.
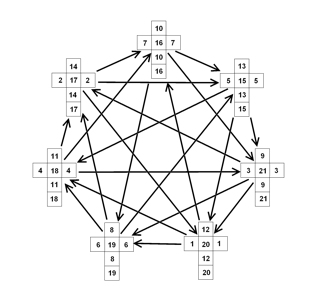
It turns out that there is. The Dutch puzzle inventor M. Oskar van Deventer came up with a set of seven non-transitive dice, with values from 1 to 21. Here two opponents may each choose a die from the set of seven, and there will be a third die with a better chance of winning than either of them. The probabilities are remarkably symmetric with each arrow on the diagram illustrating a probability of 5/9.
That's the probability of beating each individual opponent, but what about the chance of beating both at the same time? If the dice were regular fair dice, with two competing dice having a 50-50 chance of victory (ignoring draws), then the chance of beating two opponents at the same time would stand at just over 25%. It isn't exactly 25% because the event of beating one player isn't independent of the event of beating the other. This is because if you roll a high number, you're likely to beat both. In the case of van Deventer's dice, the chance of beating both opponents at the same time is around 39%. So even though you have the advantage against both opponents, beating both players is still a challenge.
Is it possible to construct a set of non-transitive dice with a higher chance of beating two players at the same time and possibly even involving less dice? Yes it is. I have devised such a set below.
Grime dice
Here is a set of five non-transitive dice:

These dice use values from 0 to 9, as follows:
| Die A: | 4 | 4 | 4 | 4 | 4 | 9 |
| Die B: | 3 | 3 | 3 | 3 | 8 | 8 |
| Die C: | 2 | 2 | 2 | 7 | 7 | 7 |
| Die D: | 1 | 1 | 6 | 6 | 6 | 6 |
| Die E: | 0 | 5 | 5 | 5 | 5 | 5 |
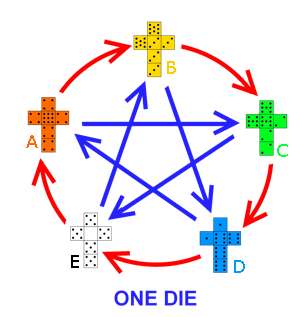
As with other sets of dice we have seen, we have a chain: A>B>C>D>E>A.
However, within that we also have a second chain: A>C>E>B>D>A.
The average winning probability for one die is 64.7%. The exact probabilities are
P(A > B) = 13/18,
P(B > C) = 2/3,
P(C > D) = 2/3,
P(D > E) = 13/18,
P(E > A) = 25/36,
P(A > C) = 7/12,
P(B > D) = 5/9,
P(C > E) = 7/12,
P(D > A) = 13/18,
P(E > B) = 5/9.
This means that given two dice, you cannot always find a third die that beats both. For example, this is the case when the two given dice are C and E.
If our original set of three non-transitive dice was like a game of Rock, Paper, Scissors, this diagram is closer to the related, but more extreme, non-transitive game Rock, Paper, Scissors, Lizard, Spock
With two dice the first chain stays the same. But the second chain now flips, so A>D>B>E>C>A.
The average winning probability for two dice is 59.2%. The exact probabilities are
P(A > B) = 7/12,
P(B > C) = 5/9,
P(C > D) = 5/9,
P(D > E) = 7/12,
P(E > A) = 625/1296 (a slim defeat, but in the short term the effect is 50-50),
P(A > D) = 56/81,
P(B > E) = 56/81,
P(C > A) = 85/144,
P(D > B) = 16/27,
P(E > C) = 85/144.
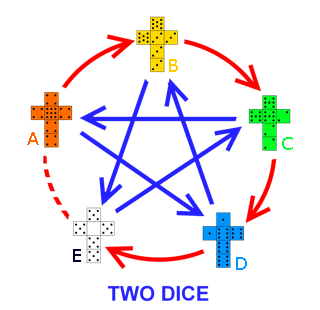
Overall, the average winning probability is 61.9%. This gives us a way of playing the game against two opponents: invite each player to pick one of the dice, but do not volunteer the rules of the game at this point. When the two other players have made their choice, you may now make your choice, including whether you and your opponents will be playing the one die or two dice version of the game. As before we play a game of say 10 rolls and, no matter which dice your opponents choose, there will always be a die with a better chance of beating each player.
For example, if one opponent chooses die B and the other opponent chooses die C, then you should pick die A and play the one die version of the game. Then, according to the first diagram above, you will have a better chance of winning than either individual opponent.
On the other hand, if one opponent chooses die C and the other opponent chooses die E, then you should pick die B and play the two dice version of the game. Then, according to the second diagram to the right, you can expect to beat each opponent again.
A gambling game
But, can we expect to beat the two other players at the same time? Well, we have certainly improved the odds, with the average probability of beating both opponents now standing at around 44%. That's a 5% improvement over van Deventer 's dice, and a 19% improvement over fair dice.
But if the odds of beating two players isn't over 50% how can we win? Consider the following gambling game:
Challenge two friends to a game in which you will play both of them at the same time. If you lose, you will give your opponent £1. If you win, your opponent gives you £1. So if you beat both players at the same time, you win £2; if you lose to both players, you lose £2; and if you beat one player but not the other then your net loss is zero. You and your friends decide to play a game of 100 rolls.
If the dice were fair, then each player would expect to win zero, since each player would be expected to win half the time and lose half the time.
With van Deventer 's dice, you should expect to beat both players 39% of the time, and lose to both players 28% of the time, which will give you a net profit of £22.
But with Grime dice, you should expect to beat both players 43.8% of the time, but only lose to both players 22.7% of the time, giving you an average net profit closer to £42! (And possibly the loss of two friends).
This set is the best set of five non-transitive dice with these properties that I have found.
I invite you to try out these games yourself. They are easy to make by either writing on blank dice, or modifying some old dice. Try them out on your friends and enjoy your successes and failures!
Here's a further challenge
For the very keen, here's a set to try that uses mathematical constants:
| Die A: | 1 | 1 | 1 | 1 | 1 | π |
| Die B: | Φ | Φ | Φ | e | e | e |
| Die C: | 0 | φ | φ | φ | φ | φ |
where e is Euler's number (2.718...), φ is the golden ratio (1.618...) and Φ is the conjugate of the golden ratio (0.618...). Do these dice form a non-transitive set? What about with two dice, does the order reverse as before?
Further reading
Find out more about non-transitive games in the Plus articles Winning odds and Let 'em roll.
About the author

James Grime is a lecturer and public speaker on mathematics, and can be mostly found touring the country on behalf of the Millennium Mathematics Project carting his trusty Enigma Machine. If you'd like James and the machine to visit your school, visit the Enigma website.