Over 2000 years ago the Greek mathematician Euclid of Alexandria established his five axioms of geometry: these were statements he thought were obviously true and needed no further justification. The first three are indeed pretty obvious (see here) postulating, for example, that through any two points there is a straight line. The fourth one, however, sounds a bit weird. It says:

All right angles are equal to each other.

This statement seems pretty vacuous: a right angle is a 90 degree angle, and obviously all 90 degree angles are equal. But we can understand Euclid's need for stating this fact when we consider that he didn't describe right angles in terms of degrees or any other angle measure (he didn't have one). Instead, he defined them in terms of a geometric picture: if two lines intersect, and the two angles formed by that intersection are equal, then that's your right angle.

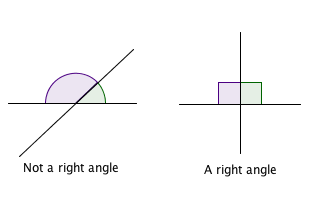
Now suppose you have two angles that adhere to that definition of being right drawn on two separate bits of paper (or papyrus if you're Euclid). You might have a nagging suspicion that perhaps those two angles are different from each other. The fourth axiom says that they're not. Those right angles are in fact equal in the sense that you could move one of them on top of the other so that they perfectly align. Having established that fact Euclid could then go on to measure other angles in terms of right angles, for example by saying that a given angle is equal to three right angles.

You can find out more about the fourth axiom in this very interesting article.

Return to the Plus advent calendar 2016.

This article comes from our Maths in a minute library.