
Kip Thorne
In brief: The laws of physics allow members of an exceedingly advanced civilisation to travel forward in time as fast as they might wish. Backward time travel is another matter; we do not know whether it is allowed by the laws of physics, and the answer is likely controlled by a set of physical laws that we do not yet understand at all well: the laws of quantum gravity. In order for humans to travel forward in time very rapidly, or backward (if allowed at all), we would need technology far far beyond anything we are capable of today.
Travelling forward in time rapidly
Albert Einstein's relativistic laws of physics tell us that time is "personal". If you and I move differently or are at different locations in a gravitational field, then the rate of flow of time that you experience (the rate that governs the ticking of any very good clock you carry with you and that governs the aging of your body) is different from the rate of time flow that I experience. (Einstein used the phrase "time is relative"; I prefer "time is personal".)
Example of Twins Paradox
Florence rides in a space ship that accelerates outward from Earth on a straight line with one Earth gravity of acceleration, $g=9.81 m/s^2$ for a time $T_F/4$ (as measured by her), then decelerates at $g$ for a time $T_F/4$, winding up at rest relative to Earth but very far away. Florence then accelerates back toward Earth at $g$ for a time $T_F/4$ and decelerates at $g$ for $T_F/4$, winding up at rest on Earth. When the trip is finished and Florence meets her Earth-bound twin Metheuselah, she has aged by $T_F$ while he has aged by a larger amount, $T_M$. A fairly simple calculation (see [7]) using the laws of special relativity gives the following expression for Methuselah's aging in terms of Florence's: $$T_M = (2 c/g) [exp(gT_F/4c)- exp(-gT_F/4c)].$$ Here $exp(x) = e^x$ is the exponential function and $c$ is the speed of light (about 299.8 million m/s). This relationship is plotted in the following figure:
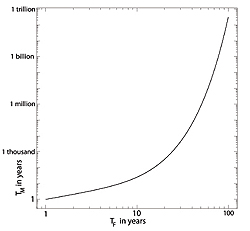
Click here for a larger version of the graph.
If Florence's clocks and aging report a round trip time of 10 years, Methuselah will have aged by 25 years. If Florence aged 30 years, Methuselah will have aged 4,500 years. If Florence aged 88 years, Methuselah will have aged 14 billion years, which is the current age of our Universe! Unfortunately, no known rocket fuel, not even thermonuclear fusion, is capable of producing the sustained multi-year-long acceleration required for such a trip.
This personal character of time allows one person to travel forward in time much faster than another, a phenomenon embodied in the so-called twins paradox. One twin (call him Methuselah) stays at home on Earth; the other (Florence) travels out into the Universe at high speed and then returns. When they meet at the end of the trip, Florence will have aged far less than Methuselah; for example, Florence may have aged 30 years and Methuselah 4,500 years. (The twin that ages least is the one who undergoes huge accelerations, to get up to high speed, slow down, reverse direction, then accelerate back and slow to a halt on Earth. The twin who leads the sedate life ages the most.)
A massive black hole is another vehicle for rapid forward time travel: If Methuselah remains in orbit high above the event horizon of a massive black hole (say, one whose gravitational pull is that of a billion suns) and Florence travels down to near the event horizon and hovers just above it for, say, 30 years and then returns, Methuselah can have aged thousands or millions of years. This is because time flows much more slowly near a black hole's event horizon (where the acceleration of gravity is huge) than far above it (where one can live sedately).
These time travel phenomena have been tested in the laboratory. Muons — short-lived elementary particles — travelling around and around in a storage ring at 0.9994 of the speed of light, at the Brookhaven National Laboratory on Long Island, New York, have been seen to age 29 times more slowly than muons at rest in the laboratory. And atomic clocks on the surface of the Earth have been seen to run more slowly than atomic clocks high above the Earth's surface — more slowly by about 4 parts in 10 billion.
Travelling backward in time: chronology protection
We physicists have been working hard since the late 1980s to understand whether the laws of physics allow backward time travel. We do not have a definitive answer yet, but the likely answer has been summarised by Stephen Hawking, in his Chronology Protection Conjecture (see [1]): The laws of physics always conspire to prevent anything from travelling backward in time, thereby keeping the Universe safe for historians.
We physicists have identified two mechanisms that might protect chronology: (1) The exotic material that is required in the manufacture of any time machine might be forbidden to exist, by the laws of physics — forbidden to exist in the large amounts that time machines always require. (2) Time machines might always self-destruct, explosively, when one tries to activate them.
These mechanisms (1) and (2) are descriptive translations of mathematical results that we physicists have derived using the laws of physics expressed in their own natural language: mathematics. The sentences (1) and (2) capture the essence of our calculations, but crucial details are lost in translation. For anyone who wishes to struggle to understand those details, good places to start are a recent beautiful but highly technical review article by John Friedman (see [2]), and a much less technical but older and slightly outdated article by Matt Visser (see [3]).
I shall illustrate these chronology-protection mechanisms by an example of a time machine that my students Mike Morris and Ulvi Yurtsever and I invented and explored mathematically in 1989: a time machine based on wormholes. (This is just one of many time-machine designs that have been studied. For others see Visser's review, [3])

Me crawling through a wormhole whose length is only a few centimetres but circumference is about that of my belly. (From my book Black Holes and Time Warps, [4], where you can find a more detailed description of this time machine.)
A wormhole-based time machine: A wormhole is a hypothetical tunnel through hyperspace that links one place in our Universe (e.g. my office at Caltech) to another place (e.g. the Caltech football field). Each end of the wormhole (each mouth) looks like a crystal ball. Staring into it, one sees a distorted image of objects at the other end. Looking into the mouth in my office, I see the football field, distorted; someone on the football field, looking into the mouth there, sees me and my office, distorted. The wormhole (tunnel) might be only 3 metres long, so if I enter the mouth in my office and then travel just 3 metres through the tunnel, I emerge from the other mouth, onto the football field 300 metres from my office.
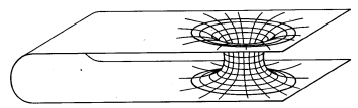
A wormhole as viewed from a higher-dimensional hyperspace. Our Universe is the two-dimensional sheet. The wormhole is a short cut through hyperspace from one location in our sheet (our Universe) to another.
Suppose, now, that a creature from an extremely advanced civilisation carries the football-field mouth out into the Universe on a "twins paradox" trip. When that mouth returns, it may have aged by only one second while the mouth in my office aged by one day. The wormhole has become a time machine: If I enter one mouth and travel through it for only a few seconds, I emerge from the other mouth one day in the future. Travelling through it in the other direction, I emerge one day in the past! (See [4].)
Exotic Matter and Vacuum Fluctuations: We do not know whether the laws of physics permit wormholes. We do know, however, that a wormhole will implode so quickly that nothing can traverse it, unless it is held open by gravitationally repulsive forces that can only be produced by exotic matter. By the phrase "exotic matter" I mean matter that has negative energy and therefore anti-gravitates, i.e. repels.
The quantum laws of physics do permit exotic matter to exist, and it has been created in the laboratory in very tiny amounts: in the so-called Casimir vacuum between two electrically conducting plates, and in the so-called squeezed vacuum that is generated by optical physicists using nonlinear crystals.
The key to this negative energy is the fact that empty space (the vacuum) is filled with tiny fluctuations of all kinds of matter and fields that exist in the Universe. It is impossible to make these fluctuations go away. They are a consequence of the quantum mechanical uncertainty principle: if, at one moment of time, there are no fluctuations at all of (for example) the electromagnetic field, then the rate of change of the fluctuations must be infinitely large and a moment later the fluctuations will be enormous. The product of the strength of the fluctuations and the magnitude of their rate of change is always bigger than a certain limit, given by the uncertainty principle. As a result, fluctuations are always present. We call them vacuum fluctuations because they are a property of the vacuum, i.e. of otherwise empty space.
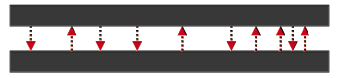 The Casimir Vacuum: When two electrically conducting plates are placed very close together, the vacuum fluctuational electric field parallel to the plates is strongly suppressed while that perpendicular to the plates is little affected. The suppression reduces the fluctuational energy between the plates below what it would be in plate-free empty space, so the vacuum between the plates (the Casimir vacuum) acquires negative gravitating energy. It has loaned some fluctuational energy to the electric fields inside the plates. The plates have catalysed this lending. |
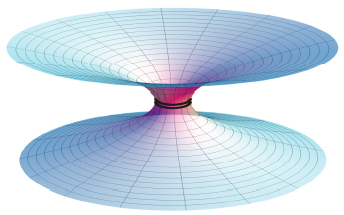 Wormhole held open by Casimir Vacuum: Two concentric spherical conducting plates are placed at the throat of a wormhole, with a tiny separation. (One dimension is suppressed here, so our Universe is a 2-dimensional sheet and the plates look like circles rather than spheres.) If the plates' energies (including their mass's energy E=mc2) are small enough, then the repulsive gravity due to the Casimir vacuum between the plates can hold the wormhole open. |
The laws of quantum physics say that vacuum fluctuations produce no gravity — or perhaps only an exceedingly tiny amount of gravity: the gravity that is accelerating the expansion of the Universe. In other words vacuum fluctuations may be responsible for the so-called cosmological dark energy. But that dark energy is so tiny (10-121 in dimensionless numbers) that it is irrelevant for my discussion of time machines; so I shall say that the quantum fluctuations produce no gravity at all.
Or, rather, they produce no gravity under normal circumstances. One can devise ways, in fact, to make one region of empty space lend some of its vacuum fluctuations to an adjacent region. (This is what experimental physicists do with the Casimir vacuum and with the squeezed vacuum.) When this happens, the lending region is left with a negative amount of gravitating energy, and the borrowing region gets positive gravitating energy. The quantum laws place tight constraints on the amount of fluctuational energy that can be loaned. The larger the size of the lending region, the less energy it can loan and therefore the less negative its energy can become. This is true in the Casimir vacuum, in the squeezed vacuum, and in all other variants of exotic matter. The laws of physics dictate it.
These constraints on the amount of negative gravitating energy might be severe enough to prevent one from ever accumulating enough of it to prevent a wormhole from imploding (see [5]). The reason is that regions of space which do the borrowing and the devices which catalyse the borrowing might always have so much positive energy of their own, that their attractive gravity counteracts the negative energy's repulsive gravity, and triggers all wormholes to implode. If that is the case, then wormhole-based time machines are forbidden: one can never travel through a wormhole before it implodes. (John Friedman and colleagues have called this topological censorship, see [2].)
My personal guess is that these constraints on exotic matter do not prevent wormholes from being held open and thus do not protect chronology, but I could well turn out to be wrong. To learn the truth, we physicists must develop a deeper understanding of quantum theory in warped spacetime than we now have — i.e. a deeper understanding of the combined laws of quantum theory and general relativity, the laws of quantum gravity.
Time machine self destruction: If it turns out that wormholes can be held open, then doing so is not enough to guarantee that an ultra-advanced civilisation can convert a wormhole into a time machine via a twins-paradox trip (carrying one mouth out into the Universe at high speed and then back). There is a second obstacle that must be surmounted — time machine self destruction:

Time machine self destruction: As the right wormhole flies back toward the left, at the end of its twins-paradox trip, vacuum fluctuations flow through the wormhole then out through the space between them, returning to their starting point at the moment they left. Their gravitating energy grows extremely large, and perhaps destroys the wormhole at the moment it becomes a time machine. (Figure adapted from my book Black Holes and Time Warps, [4].)
As the travelling mouth is returning to Earth, there comes a first moment when its wormhole can be used to travel backward in time. The first thing that can do so, and thereby meet itself before it left, is an entity that enters one mouth, exits from the other before it entered, and then flies through the Universe back to its starting point at the highest possible speed, the speed of light — arriving back at the first mouth at precisely the moment it started its trip. Even if no light or other light-speed radiation travels on this round-trip time-travel route, vacuum fluctuations will always do so. They cannot be stopped. Upon arriving back at their starting point at the very moment when they left, the vacuum fluctuations will pile up on top of their younger selves. The result is a duplicate of every fluctuation, and then, with another round trip, a quadrupling of every fluctuation, and so forth. The bottom line, according to a calculation that I did with my postdoc Sung-Won Kim in 1990, is an explosive flow of gravitating fluctuational energy through the wormhole at precisely the moment when time travel is first possible — at the moment of time machine activation [4].
Will this explosive fluctuational energy destroy the wormhole and thence the time machine? At first Kim and I thought the wormhole could survive. However Stephen Hawking gave strong arguments to the contrary, in his seminal 1991 research paper on chronology protection. The explosion is very likely to destroy the time machine when it is first activated, Hawking argued — and not just this time machine, but any time machine that even the most advanced civilisation might conceive and build. Over the next few years many other physicists weighed in, with analyses of other time machine designs, and it began to look like Hawking might be wrong: a sufficiently clever design might protect a time machine from self destruction. Then in 1996 Bernard Kay, Marek Radzikowski and Robert Wald developed a powerful mathematical proof that the version of the laws of quantum physics which we were all using to analyse time machine self destruction are incapable of revealing the explosion's outcome. The outcome is held tightly in the grip of the laws of quantum gravity, which we do not yet understand fully.

The fate of any time machine?
Hawking and I have a long history of bets with each other, about unsolved mysteries in physics. But we are not making a bet on this one, since for once we are on the same side. When we physicists have mastered the laws of quantum gravity (Hawking and I agree), we will very likely discover that chronology is protected: the explosion always does destroy any time machine, when it is first activated.
In June 2000, on the occasion of my 60th birthday, Hawking presented me with a tentative analysis of the explosion's outcome, using his own tentative version of the laws of quantum gravity. His conclusion: if I try to use a very advanced civilisation's wormhole to travel backward in time, the quantum mechanical probability that I will succeed is one part in 1060; see Hawking's article in my birthday party book, [6]. That's an awfully small probability of surviving the explosion. Given the opportunity to try, I would not take the risk.
Other time machines: It is amazing what we can learn from the laws of physics, when we understand them well. One famous example is the laws' absolutely firm insistence that it is impossible to construct a perpetual motion machine, even if one has all the tools of an exceedingly advanced civilisation. Another example is a proof by Hawking that to make a time machine, no matter how one goes about it, one must use exotic matter — matter with negative energy — as an integral part of the device; wormholes illustrate this, but it is true in general. And a third example is the proof by Kay, Radzikovsky and Wald that the laws of physics as we now know them will break down whenever a time machine is activated, no matter how one designs the machine. Again wormholes are just one example. Hawking's theorem, and that of Kay, Radzikovsky and Wald, tell us that the fates of all time machines are held tightly in the grip of the laws of quantum gravity.
Progress in the quest to understand quantum gravity has been substantial over the past two decades. Complete success will come, I am convinced, within the next two decades or so — and it will bring not only a clear understanding of whether backward time travel is possible, but also an understanding of many other mysteries, including how our Universe was born (see the Plus article What happened before the Big Bang?).
References
[1] Stephen W. Hawking, The Chronology Protection Conjecture, Physical Review D, 46, 603 (1992).[2] John Friedman and Atsushi Higuchi, Topological Censorship and Chronology Protection, Annalen Phys. 15:109-128,2006; also available on the web.
[3] Matt Visser, The Quantum Physics of Chronology Protection, in The Future of Theoretical Physics and Cosmology: Celebrating Stephen Hawking's 60th Birthday, edited by G.W. Gibbons, S.J. Rankin and E.P.S. Shellard (Cambridge University Press, Cambridge, England, 2003); also available on the web.
[4] Kip Thorne, Black Holes and Time Warps: Einstein's Outrageous Legacy, Norton, 1994: Chapter 14.
[5] Lawrence H. Ford and Thomas A. Roman, Negative Energy, Wormholes and Warp Drive, Scientific American, January 2000. For the past twenty years, Ford and Roman have been the leading researchers on deducing what the laws of physics say about how much negative energy one can accumulate by lending.
[6] S.W. Hawking, Chronology Protection: Making the World Safe for Historians, in The Future of Spacetime, volume authored by S.W. Hawking, K.S. Thorne, I. Novikov, T. Ferris, A. Lightman and R. Price (W.W. Norton, New York, 2002); see also Chap. 5 of S.W. Hawking, The Universe in a Nutshell (Bantam, New York, 2001).
[7] Section 1.8 and Exercise 1.16 of a textbook that Roger Blandford and I expect to publish next year; an advance copy is available on the web.