Add new comment

Imaging maths - Inside the Klein bottle

September 2003

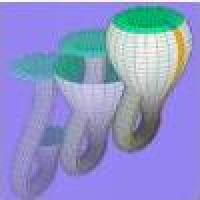
You can read a briefer introduction to the Klein bottle here.

My first practical experience with a Klein bottle was a failure. When I was still at school I came across a picture of a Klein bottle in a weekly journal. I shared my fascination with a friend who was a glassmaker specializing in physics equipment for schools. A few days later I was a proud owner of a physical Klein bottle, made of glass.

What to do with such a bottle? Why not use it for something? Filling it was not easy, because the water flowing in blocked the air inside from coming out. So I decided to keep the remaining air in the bottle and see how effective the half-filled bottle was as a thermometer. I added a few crystals of potassium permanganate to colour the water and make it easier to mark the water levels on the glass.

View the <a href="./kleinBottle_anim.html">animated version</a> (997K) or explore the <a href="./applets/appletKleinWithBand.html">java applet</a>

View the animated version (997K)

To my delight, this primitive but interesting thermometer did indeed show signs of life during the day. Unfortunately, the next morning was a disaster, with lots of red water poured over my windowsill. It was winter, and the low temperature during the night had compressed the remaining air inside the bottle too much, bringing the water level in the handle below the lower curve, so that more air got sucked in. When the air warmed up again in the morning, the increased volume of air in the body pushed the water level too far up the handle. In those days, I never managed to find stable proportions of water and air, but the picture at the beginning of this article shows a workable configuration.

The Klein bottle was discovered in 1882 by Felix Klein [1] and since then has joined the gallery of popular mathematical shapes known to the general public outside the "ivory tower". The bottle is a one-sided surface - like the wellknown Möbius band - but is even more fascinating, since it is closed and has no border and neither an enclosed interior nor exterior. Following Klein we use visual models to study this surface.


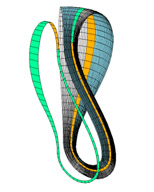The two Möbius bands of a Klein bottle are connected by an ordinary two-sided band whose back and front sides are colored white and blue respectively - see the animated version (364K)

From the Möbius band to the projective plane

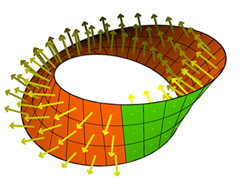
The Möbius band is one-sided - view the animated version (1.3M)

The Möbius band is the simplest one-sided surface, and is easy to make out of a strip of paper. Mark the two sides of the paper - by drawing some red dots on the front and some green dots on the back, for example. Now take two ends of the strip and stick them together after making a single twist in the strip, so that the red-dotted side joins the green-dotted side. This is a Möbius band, and moving along the surface will bring us to each of the red and green dots without us ever crossing a boundary.

The band was discovered in 1858 by the German astronomer and mathematician August Ferdinand Möbius. Adding 0 twists, 2 twists, or more generally, an even number of twists, will always produce a two-sided surface. Similarly, uneven numbers of twists produce various one-sided surfaces. Interestingly, the boundary of a Möbius band is a single closed curve.

Topology is the mathematical discipline which investigates those properties of shapes which do not change under continuous bending and stretching. For example, if a Möbius band is made out of a rubber sheet and we stretch it slightly without breaking it, it will still be a one-sided surface. In contrast, if we had glued the two ends of the strip without first twisting, the resulting cylindrical shape would have been the topologically different two-sided cylinder.

Despite its simplicity the Möbius band was a genuine mathematical discovery. Reasoning about orientability of surfaces is one of the keys to understanding and classifying surfaces and manifolds in topology.

A follow-up task in topology is to get rid of the remaining boundary of the Möbius band to produce a closed surface. The simplest solution would be to use the rubber Möbius band and pull all boundary points continuously together, just as we can pull the points of a circle to a cone. Whether or not we round off the tip of the cone, we obtain a closed surface without boundary, and it is one-sided since from everywhere we can always go to the Möbius band and then switch sides as before. This surface is called the projective plane, and topologically it is the simplest closed one-sided surface. Unfortunately, the geometric realizations of the projective plane are quite sophisticated. For example, it wasn't until 1903 that Werner Boy [17], at the suggestion of David Hilbert (his PhD supervisor at the time), found a geometric realization of the projective plane which has no sharp corners or edges. Fortunately, a different closing of the Möbius band is much simpler to obtain.

The Klein bottle is not a doughnut

One construction of a torus - a doughnut-like shape - starts with a sheet of paper, rolls it up to form a cylinder, and then bends both ends around to close the shape. The inside of the cylinder on one side is connected with the inside on the other side, and the same with the outside. Therefore the torus is a two-sided surface.

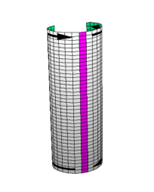
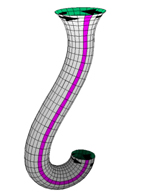
But we could also use the cylinder to make a Klein bottle. Instead of adding a twist, as we did when making a Möbius band from a strip of paper, we loop one end back through the cylinder and glue it to the other end, with the two boundary circles given opposite orientations. To accomplish this with a pleasing shape we adjust the thickness of the cylinder. This allows us to glue inside to outside, and obtain a one-sided surface. In the diagram below we have used white and green to distinguish the two sides of the original cylinder. When the Klein bottle is finished, the colors still show where the cylinder was glued together, but gluing at any other parallel circle would have been just as good.

In his original work [1], Klein introduced the bottle as a "certain unbounded double surface" which "can be visualized by inverting a piece of a rubber tube and by letting it pass through itself so that outside and inside meet".


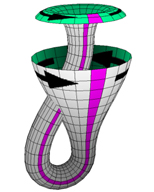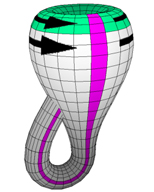A Klein bottle is formed by joining two sides of a sheet to form a cylinder, then looping the ends of a cylinder back through itself in such a way that the inside (green) and outside (white) of the cylinder are joined - view the animated version (632K)

Unfortunately, the Klein bottle does not bound a volume - in other words, it has no interior. This means you could put twice as much sugar on a "Klein bottle" doughnut as on a torus doughnut, but it would have no dough inside!

Orientability and one-sidedness

Half of a Klein bottle with MÃ¶bius strip

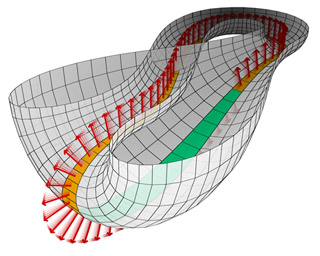
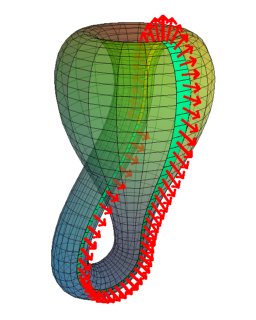
Half of a Klein bottle with Möbius strip - view the animated version (1.3M)

When studying the Möbius band or the Klein bottle, orientability and one-sidedness are important. A surface is one-sided if, standing upright, you can walk along the surface and reach both sides of each point of the surface. Most surfaces in nature are two-sided. For example, the round sphere is two-sided, which ensures that we always walk on top of the earth and never below. Similarly, a wheel-shaped torus, a pretzel, and, more generally, all surfaces that enclose a solid volume, are two-sided.

In nature we do not usually see one-sided surfaces, and remember that the first one-sided surface found by Möbius was an abstract mathematical construction. By drawing perpendicular arrows (normal vectors) along the Möbius band in this illustration of the Klein bottle we emphasize its one-sidedness: by continuous movement we can transport the arrows to both surface sides of a point, meaning that we cannot distinguish between its front and back. Unfortunately, the very concept of one-sidedness depends on the ambient (surrounding) space - for example, closed curves (loops) in 3-dimensional space do not have sides, although in 2-dimensional space they do.

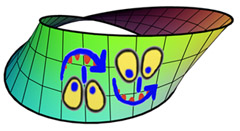
Mirror image and orientability - see the animated version (73K)

A surface is said to be orientable if a shape drawn on it cannot be transformed into its mirror image by simply moving the shape along a path on the surface. Consider the right-way-up face in the illustration. If you move this face around the Möbius band, it returns as its mirror image (and upside-down). This means that the Möbius band is non-orientable. The notion of orientability extends to higher-dimensional spaces, for example, in a non-orientable 3-dimensional universe there would be a way of throwing a right glove so that it returned to you as a left glove!

In contrast to one-sidedness, orientability is an intrinsic property and does not require that a surface is embedded in an ambient space. Since topologists have worked out ways to think about shapes without an ambient space, the notion of orientability is in general much more applicable than that of one-sidedness. Nevertheless, for surfaces in our 3-dimensional world one-sidedness is a very natural concept.

Mathematical Models

Felix Klein

When Klein became a Professor in Leipzig in 1880, he immediately started to acquire mathematical models and establish a model collection. Klein was a geometer and used these plaster models in his university lectures. Model collections became very popular in mathematics departments world-wide. When he then moved to Göttingen, Klein, together with his colleague Hermann Amandus Schwarz, expanded his new department's collection of mathematical models and instruments so much that at peak times up to 500 models were on permanent display. When you think that a model could cost about £150, this was a major investment in education.

Following the success of mathematical models, in 1893 the Prussian government decided to participate in the World's Columbian Exhibition in Chicago with a university exhibition. Klein and his former student Dyck organized an Exhibition of Mathematics, including about 100 mathematical models and instruments. Production of mathematical models by the publisher Martin Schilling and others stopped in the beginning of the 20th century but many of the plaster shapes are still around in university mathematics departments [14]. Photographs of many plaster models are also available in the internet. Nowadays, repositories like the Electronic Geometry Models journal [15], hosted in Berlin, provide peer-refereed digital models as a source for mathematical experiments.

Make your own...Klein bottles

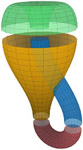
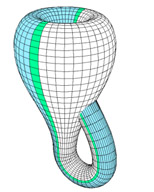
The standard Klein bottle

The standard (Euclidean) Klein bottle can be assembled from four elementary building blocks as shown to the right. Three of the blocks are parts of surfaces of rotation and the vertical blue tube is a slightly deformed cylinder. Stewart Dickson's page [13] shows the assembly in detail.

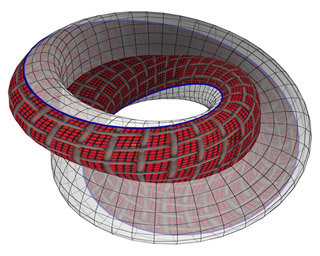
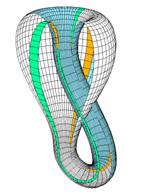
The Figure-8 Klein bottle

The Figure 8-Klein bottle

The Figure-8 surface shown on the left has an even simpler parameterization than the standard Klein bottle. It starts with a planar curve in the xz-plane in the form of an 8. While rotating this curve around the z-axis we add a twist so that the inside is joined with the outside after the loop. Note that the two Möbius bands of this surface are swept out by the crossing of the 8. A Figure-8 curve is given by c(u)=(2cos u, 0, sin 2u), where u runs between 0 and 180^o. Applying the rotation and twist we derive the Figure-8 surface:

$F(u,v)= \left( \begin{array}{c} \sin v (4 +2\cos u \cos tv - \sin 2u \sin tv) \\ \cos v (4 +2\cos u \cos tv - \sin 2u \sin tv) \\ 2\cos u \sin tv + \sin 2u \cos tv \end{array} \right),$

where u and v are both in between 0 and 360^o. The parameter t=0.5 determines the twist. The figure above shows how constraining u to the interval [0,180^o] or [180^o,360^o] produces a Möbius strip, shown in red and white respectively.

The Lawson-Klein bottle

H.B. Lawson came across an elegant realization of a Klein bottle in the 3-sphere S³ among a family of helicoidal - staircase-like - surfaces [12]. The figure on the right shows a projection of the surface into ordinary 3-dimensional space, R³, where the top part of the surface is clipped away to enable an inside view. Along the blue central circle you can see a red and a green Möbius band intersecting, just as in the Figure-8 Klein bottle seen above. By the way, the Lawson-Klein bottle is also a minimal surface in the 3-sphere.

The 3-sphere is the 3-dimensional space consisting of all points at distance 1 from the origin (0,0,0,0) in 4-dimensional Euclidean space. It is a higher-dimensional analogue of the familiar sphere in 3-Euclidean space. And, just as great circles (circles with maximum possible radius) are the shortest routes between two points on the 3-sphere, they also give the shortest routes on the 4-sphere. (Such shortest routes are known as geodesics, and you can find out more about geodesics in Time and motion from Issue 7 of Plus.)

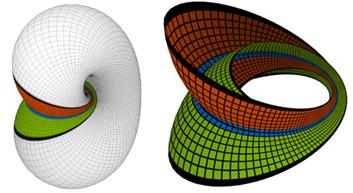
Two Möbius bands on the Lawson-Klein bottle in S³ intersect orthogonally along their base circle

All the grid lines shown on the figure of the Lawson-Klein bottle are great circles, which intersect the blue central circle and which twist when moving along that circle. This spiraling allows a simple formulation of the Lawson-Klein bottle as a helicoidal surface, and other interesting shapes arise when the twist rate is changed. We will devote a future issue of "Imaging Maths" to un-twisting the properties of twisted "Staircases in Space Forms", with an explicit formula for the Lawson-Klein bottle as a spin-off.

Conclusion

Visualization effect of JavaView

The Möbius band and the Klein bottle were discovered in the 19th century during the search for a classification of surfaces and shapes. Often mathematical shapes are first imagined as a technical tool in an abstract investigation, while some of their beauty remains in the unexplored darkness. From time to time we discover among them a new jewel, a shape in its own right, and then slowly recognize it as a member of an eternal visual repository.

Notes

Genuine glass and other real Klein bottles are available from Acme Klein bottle or at the science shop of Spektrum der Wissenschaft, among others.
There are also some strange versions in glass by Alan Bennett.
Orientability is a binary property of manifolds in any dimension. For example, instead of gluing opposite sides of a square one can pairwise identify opposite faces of a cube. This gives a 3-torus, or, when adding a twist, a 3-Klein bottle. Interested in life in a non-orientable universe? Then watch the video "The Shape of Space" [10].
Since the Möbius band is bounded by a single closed curve you might ask if the curve spans a non-orientable soap film when dipped into soapy water. This was answered in the affirmative by a more general construction of W. Meeks, who provided the explicit formula of a minimal Möbius band as an immersion of C-{0} into R³ [16].
Lopez constructed a minimal immersion of a 1-punctured Klein bottle into R³ [9]. Since minimal surfaces must extend to infinity, Lopez's immersion doesn't have the nice-looking closed form of the familiar topological immersions. An interesting but open question is whether there exists a compact Klein bottle with constant mean curvature larger than zero.
Using a different projection from R⁴ to R³, G. Francis et al. obtained a shape featured as "Etruscan Venus" [11].
The Klein bottle is topologically equivalent to the connected sum of two crosscaps. Therefore the bottle is different from gluing the boundary of a Möbius band with a small hole on a torus. This surface is equivalent to the connected sum of three crosscaps.
As part of the virtual exhibition "Surfaces Beyond the Third Dimension", Banchoff [4] includes images of the Klein bottle.
Background information on mathematical terms related to the Klein bottle and this column can be found at Weisstein's MathWorld [5].

About this article

Konrad Polthier is full professor of mathematics at Freie Universität Berlin and the DFG research center MATHEON, and chair of the Berlin Mathematical School. Konrad Polthier received his PhD from the University of Bonn in 1994, and headed research groups at Technische Universität Berlin and Zuse-Institute Berlin. His current research focuses on discrete differential geometry and mathematical problems in geometry processing applications. Dr. Polthier co-edited several books on mathematical visualisation, and co-produced mathematical video films. His recent video MESH (www.mesh-film.de, joint with Beau Janzen, Los Angeles) has received international awards including "Best Animation" at the New York International Independent Film Festival.

Images, animations and applets on this page were produced with the JavaView software (www.javaview.de).

Plus.Maths.org