
B.S. Sathyaprakash
This article was part of a project we ran to celebrate the International Year of Astronomy 2009. The project asked you to nominate the questions about the Universe you'd most like to have answered, and this is one of them. We took it to Professor Bangalore Sathyaprakash of the School of Physics and Astronomy at Cardiff University, and here is his answer. This interview is also available as a podcast.
Gravity according to Newton
"According to Newton's relatively simply picture, gravity is a force that works between two objects," says Sathyaprakash. "So if you have the Earth and the Sun, for example, then the Earth feels a force that is exerted by the Sun, and in turn the Sun feels the same force, exerted by the Earth." The magnitude $F$ of this force is given by the familar inverse square law $$F=G\frac{m_1 m_2}{r^2},$$ where $G$ is the \emph{gravitational constant}, $r$ is the distance between the centres of the Earth and the Sun, and $m_1$ and $m_2$ are their respective masses.

The gravitational interaction of two spherical bodies according to Newton. Image: Dennis Nilsson.
The forces experienced by Earth and Sun may be equal in magnitude, but the resulting motion is not the same for the two bodies. According to Newton's second law of motion, the magnitude of the acceleration a body experiences when it is subjected to a force is equal to the magnitude of the force divided by the body's mass. Since the Sun's mass is large, the acceleration it experiences due to the Earth's gravitational pull is negligible compared to that experienced by the much less massive Earth. That's why the Sun remains more or less stationary, while the Earth is forced on an orbit around it.
Newton's theory of gravity, published in 1687, is remarkably accurate when it comes to most practical purposes, and went unchallenged for over 300 years. Problems arose, however, when Einstein developed his special theory of relativity in 1905. "According to Newton's theory, gravitational interaction is instantaneous. Suppose the Sun were to vanish from the horizon today. We would not notice its disappearance immediately just by looking at the Sun, because light takes some time to travel. But according to Newton's gravity, the effect of the Sun's vanishing would be felt immediately, as the Earth would fly away in a tangential direction to its original path." Einstein's special theory of relativity, however, states that nothing, not even information, can travel faster than the speed of light. "It's possible to use the vanishing Sun analogy to construct [theoretical] gravitational telegraphs which would transmit information instantaneously — and that, according to Einstein, is impossible. That's the reason why Einstein had to reformulate the theory of gravity." Einstein published his reformulation in 1916, under the name of general relativity.
Gravity according to Einstein
"Einstein's theory of general relativity doesn't really look at gravity as a force anymore, rather it replaces the concept of force by that of geometry," says Sathyaprakash. According to general relativity, massive objects curve the geometry of space, and the paths that moving objects take through space are a result of this curvature. An analogy often used is that of a bowling ball placed on a trampoline: the ball will create a dip in the trampoline, curving its surface, so a marble placed nearby will roll into the dip towards the ball. The marble's motion isn't a result of some attractive force exerted by the ball, but a result of the curvature of the surface it's moving on. The analogy is slightly wonky, since it is the Earth's gravity, and not that of the bowling ball, which creates the dip in the trampoline as the bowling ball is drawn to the floor. "We should really move out of this analogy and try and picture the full three-dimensional space, as well as time, as being curved," says Sathyaprakash. "It's because of this curvature that planets move along curved orbits, rather than straight lines."
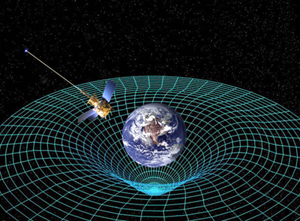
According to Einstein's general theory of relativity, massive objects curve spacetime. Image courtesy NASA.
In our own solar system the curvature of space, or rather of spacetime, as the two are inextricably linked, is mainly due to the Sun and to a small extent to Jupiter, which is next in line in terms of mass. And since these two bodies themselves move around, albeit only very slightly in the case of the Sun, the result is a dynamic system in which curvature varies continually as massive objects move around spacetime. "There is a famous phrase coined by John Archibald Wheeler: mass tells spacetime how to curve and spacetime tells mass how to move. The dynamics of Einstein's equations, the way these equations work, are encapsulated in this famous phrase."
Black holes and spacetime ripples
So far, Einstein's theory of gravity has proved amazingly accurate, and it's passed every test it's been submitted to. But two of its most important predictions still have not been completely verified. The first is the existence of the infamous black holes. These, so the theory goes, are formed when a lot of mass is concentrated in a tiny region of space. Densely packed mass exerts an immense gravitational pull, as we can easily see by momentarily reverting to Newton's inverse square law. Suppose that you are sitting on the surface of an extremely dense spherical body with a very large mass $M$ and a very small radius $r$. The gravitational force holding you to the body's surface, according to Newton, has magnitude $$F= G\frac{M m}{r^2},$$ where $m$ is your own mass. Now since $M$ is very large and $r$ is very small, $F$ in turn is very large. The body exterts a huge gravitational pull, and to escape its clutches you'd need to accelerate away from it very hard indeed. Now if the body is so dense that escape becomes impossible for everything including light, then the resulting object is called a black hole. (To be precise, it's the compactness of a body, the ratio between its gravitational radius and its physical radius, which determines whether it is a black hole.)

An artist's concept of a black hole gobbeling up a star. The star approaches (yellow blob on the left), then stretches apart (middle yellow blob), and eventually breaks into stellar crumbs, some of which swirl into the black hole (cloudy ring at right). Image courtesy NASA Jet Propulsion Laboratory.
The idea of black holes has been around since long before Einstein, but they also find a natural incarnation in his geometrical description of gravity. In Einstein's theory, a black hole is a region of space which, due to the presence of some immensely dense mass, has been curved so violently that ordinary physics breaks down in its vicinity. According to the mathematical equations describing the geometry of spacetime, the curvature at the centre of a black hole is infinite. No-one has ever observed a black hole directly, but there is much indirect evidence that these monsters do exist, and physicists believe that one resides at the centre of most galaxies.
The idea of dense objects like black holes shunting their mass around a constantly warping and bending spacetime leads to the second major prediction of Einstein's theory: gravitational waves. "Near black holes the curvature of spacetime is extremely high," explains Sathyaprakash. "Now imagine two black holes moving around each other: the curvature is large but also changing. It's a bit like taking a stick and moving it around in a pond. That's going to generate ripples in the water. Only in the case of black holes, we're talking about ripples in the very fabric of spacetime." Einstein's theory predicts that these ripples, the gravitational waves, should arise whenever massive bodies move about, just as a trampoline experiences tremours when you move a heavy object on its surface. As with black holes, there is indirect evidence that gravitational waves exist, but no-one has ever detected one directly.
Why catch waves?
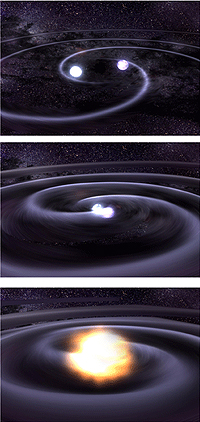
An artist's impression of gravitational waves caused by a pair of massive stars spiralling towards each other and finally colliding. Click here to see an animation of this process on the NASA website. Image: NASA/Dana Berry, Sky Works Digital.
Few things would give a more pleasing confirmation of Einstein's theory of gravity than the direct observation of black holes or gravitational waves, because both epitomise the idea of a flexing and bending spacetime. Sathyaprakash specialises in gravitational waves and believes that they in fact hold the key to both puzzles. "Black holes are black in an electromagnetic sense: they emit no light, no radio waves, and so on. But they could be emitting gravitational waves, the best example being the black hole binary system described above. By observing gravitational waves we might be able to measure the structure of spacetime geometry around black holes in a way that will never be possible in any other experiment. Most galaxies are believed to contain a massive black hole at its centre, and these black holes are not just sitting there idly. They are eating up other material and other black holes in their vicinity all the time. Now when a smaller black hole falls into a bigger one, it takes a very complicated orbit. As it goes around, the small black hole samples the geometry of spacetime in the region, and the gravitational wave signals that are emitted encode that geometry."
What's more, Einstein's equations predict that when two black holes collide, the resulting gravitational waves come with an immense amount of energy in a very short duration. Translated into brightness, this amount of energy would far exceed the light from all the stars in all the galaxies of the Universe. "So even though black holes are black electromagnetically, they could be outshining the entire Universe [in terms of gravitational waves], though only for a very short while. And this is where the interest in gravitational waves comes from."
Detecting gravitational waves
Existing evidence for gravitational waves is indirect. It comes from an astronomical system discovered in 1974 by Joseph Taylor and Russell Hulse, which contains two extremely dense neutron stars. "These stars are only about 10km in radius, but they have the same mass as the Sun" says Sathyaprakash. "Even a spoonful of the neutron star material weighs several billion tons." As with black holes, the motion of these dense stars should cause gravitational waves. Now it turns out that one of these two stars happens to be what's called a pulsar: it emits radio signals at regular intervals, which can be detected on Earth. Using these signals, Hulse and Taylor were able to establish that the two stars were spiralling towards each other. According to Einstein's theory, this can only happen if the system is losing energy in the form of gravitational waves — and the rate at which the two stars drew nearer to each other did indeed match the theory's predictions exactly.
But how can we detect gravitational waves directly? How do they manifest themselves? "Gravitational waves interact with any particle, indeed with any mass," says Sathyaprakash. "In fact, that's the trouble, they interact with everything so democratically that it's very hard to detect them, so we have to construct very sensitive instruments. Basically the tiny distortions in spacetime caused by gravitational waves move masses around. The instruments that currently exist to detect these movements are called laser interferometer gravitational wave detectors." These detectors contain mirrors, and the goal is to detect the tiny movements of these mirrors due to gravitational waves using laser interferometry (see the Plus article Catching waves with Kip Thorne for more information on this technique).
"Currently there are five gravitational wave detectors that are operating," says Sathyaprakash. "There are three instruments in the USA, called Ligo, and two in Europe, called Virgo and GEO 600. One more is being planned in Japan. These detectors are expected to make the first observations around 2014. The goal is to construct a global array of detectors, which can see sources from almost anywhere in the Universe."

An artist's impression of LISA. Click here to see an animation of LISA in orbit. Image: NASA/Dana Berry, Sky Works Digital.
Two ambitious projects are the laser inteferometer space antenna (LISA), a space-based detector scheduled to take flight some time around 2020, and the Einstein telescope, which could be built around 2025 or 2030. Both will be able to detect gravitational waves from black hole collisions far away in the Universe. Binary black holes and neutron stars will also provide astronomers with an improved way of overcoming the notoriously difficult problem of measuring cosmic distances. Comparing the observed intensity of a gravitational wave from a black hole merger with its intrinsic intensity (which can be calculated theoretically), it is possible to calculate how far the source of the wave is away from us. Thus, observations of waves from many black hole mergers will provide a cosmic distance ladder, distance markers in space and time against which other distances can be measured. This helps to determine some crucial features of the Universe, for example how fast it is expanding. Currently astronomers use other phenomena, for example supernovae, to construct the cosmic distance ladder, but Sathyaprakash says that gravitational waves will greatly increase accuracy (see the Plus article Hubble's top five achievements to find out more about how distances are currently determined).
One exciting possibility is the detection of waves that emanate all the way from the Big Bang. "A tiny fraction of a second after the Big Bang gravitational waves might have been produced. These are travelling to us unscathed without any corruption. By detecting them, we'll be able to deduce the conditions in the very early Universe. In a paper in Nature last month we have already set limits on how strong these gravitational waves could be."
But what if none of the sophisticated detectors that will come into action over the next twenty years will detect any waves? Will that throw doubt on their existence, or even Einstein's theory of gravity as a whole? "We know that gravitational waves exist — I don't think that anybody in their right mind would doubt [Hulse and Taylor's neutron star evidence]," says Sathyaprakash, "In fact, Hulse and Taylor were awarded the 1993 Nobel Prize in Physics for their discovery. What might very well happen, though, is that the sources of gravitational waves turn out not to be very common. The next generation of detectors will be sensitive to a distance of 600 million light years, and the question is whether that's good enough. But current predictions based on the Hulse-Taylor binary pulsar say that we will see events within about 600 or 700 million light years, and that's the distance that advanced detectors are aiming to achieve."
And if we do detect gravitational waves, will we be able to put them to some use here on Earth? "It's not impossible, but it's currently implausible. But when Hertz conducted his first experiments with radio waves he was of the same opinion — so who knows?"
To find out more about black holes and gravitational waves, play this online game developed by Sathyaprakash and his team, have a look at this online tutorial, or the Einstein online website. And don't forget that this interview is also available as a podcast.