The Abel Prize 2011 goes to John Willard Milnor of Stony Brook University, New York for "pioneering discoveries in topology, geometry and algebra". The Abel Prize is one of the most important international prizes in mathematics. It's awarded annually by the Norwegian Academy of Science and Letters and carries a prize money of around £650,000.

John Milnor
Announcing this year's prize, the Abel Committee noted, "Milnor is a wonderfully gifted expositor of sophisticated mathematics. He has often tackled difficult, cutting-edge subjects, where no account in book form existed. Adding novel insights, he produced a stream of timely yet lasting works of masterly lucidity. Like an inspired musical composer who is also a charismatic performer, John Milnor is both a discoverer and an expositor".
Former Fields medallist Tim Gowers, who gave the prize speech, praised Milnor's work not just for its impact and deep insights, but also or its clarity and "supreme beauty".
Milnor's work spans several mathematical fields, but he was particularly interested in the study of shapes. In 1962 Milnor was awarded the Fields Medal, another high honour in mathematics, for opening up a new area called differential topology. While geometry looks at objects and shapes in terms of properties like length, area, angle and curvature, topology allows the values of these properties to vary: to a topologist, a deflated football and a sphere are the same, as one can be transformed into the other without cutting or tearing.
Differential topology is slightly more restrictive and only considers shapes to be the same if they can be transformed into each other in a "smooth" way, not allowing jerks or kinks in the transformation process. Initially, the two ways of looking at shapes seemed the same: after all, if you can morph one object into another, then surely it must be possible to smooth out any jerks and kinks in the morphing process. But one of Milnor's earliest and most important results in this area showed that this is not necessarily true. In fact, Milnor showed that if you venture into 8-dimensional space you can find 7-dimensional spheres that are topologically the same, but cannot be transformed into each other in a smooth way. This is a surprising result and Milnor referred to these new kind of spheres as "exotic spheres". (You can find out more about higher-dimensional spheres and differential topology in this Plus article.)
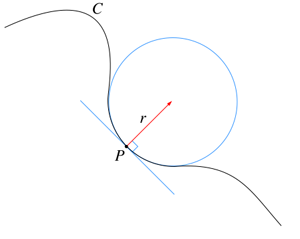
The curvature at a point is 1/r, where r is the radius of the tangent circle at the point.
Another interesting result of Milnor's concerns another class of objects beloved by topologists: knots. When you tie a piece of string into a knot you need to bend it round, in other words, the string needs to be curved. If you now consider any point on the knot, you can define the \emph{curvature} of the knot at that point: find the biggest circle that touches the knot at your point, but does not cross the knot anywhere near your point. The curvature of the knot at your point is defined to be $1/r$, where $r$ is the radius of the circle. This makes intuitive sense: if the knot is very curved at your point, then you will only be able to squeeze in a small circle, so $r$ is small and the curvature $1/r$ is large. You can define the overall curvature of the knot by averaging the curvature over all points and multiplying by the length of the string. If your knot is not really a knot, but just a circle, then this gives an overall curvature of $2\pi$: the curvature at every point is $1/r$, giving an average of $1/r$, and the length of the circle is $2\pi r.$ This reflects the fact that to make a circle, you need to bend the string round once.
If you now look at a genuine knot, which is not just a circle, you immediately see that you need to bend the string round at least twice, and then a little more, to ensure that you get something genuinely knotted. This is the result that Milnor proved at the tender age at 19: that the curvature of a knot is at least $4\pi$.
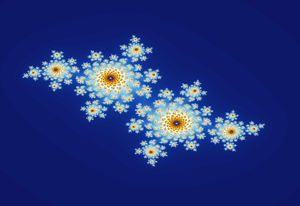
This is a Julia set, associated to the dynamics of a quadratic polynomial.
These may seem like two isolated results, but Milnor's work actually laid the foundation for whole fields of mathematics. Ever since the 19th century, geometry and algebra have been closely linked: we can describe a shape like a circle or sphere using symbols and coordinate systems. Similarly, there is a field called algebraic topology which uses algebra to gain insights into topology and vice versa. Milnor made significant contributions to algebraic topology, in particular a sub-field called K-theory, which benefited both topologists and algebraists and led to new notions that have developed into areas of their own.
Apart from topology and algebra, Milnor also made significant contributions to the field of dynamical systems. Given a mathematical function, for example the quadratic polynomial $$f(x)=x^2-1$$ you can create a dynamical system by taking an initial point $x_0$, then computing the value $f(x_0)$, then the next value along, $f(f(x_0))$, then $f(f(f(x_0)))$ and so on. You can ask where this sequence ends up: does it land on a fixed point, or bounce back and forth between two points, or escape to infinity? Questions of this type have led to the first examples of mathematical chaos and to fractal images like the \emph{Mandelbrot set} and \emph{Julia sets}, which have achieved fame outside mathematics. Together with another Fields medallist, William Thurston, Milnor developed a seminal theory for understanding the dynamics of a particular class of such functions.
Milnor joined the prize announcement ceremony, which took place in Norway today, via video link. Asked by Gowers whether he considered himself a problem solver or a theory builder, Milnor replied, "A problem solver. I never looked for the big theory, but tried to make small steps and ask intriguing questions. But you never know what can come from this."
Apart from his contributions to mathematics, which go far beyond what we have mentioned here, Milnor was also noted for his talent for communicating his ideas to others, which has inspired more than a generation of mathematicians. As Gowers put it, "[Milnor] has had a profound influence on mathematics over the past six decades and is one of the giants of the subject."
Further reading
- You can find lots of interesting information about Milnor and his work, including a paper by Tim Gowers on the Abel Prize website.
- You can read about topology and knot theory on Plus.
- And you can find out about the dynamical systems from quadratic polynomials in the Plus article Unveiling the Mandelbrot set.