Mathematical mysteries: How unilluminating!
In the 1950's, Ernst Straus asked a seemingly simple problem. Imagine a dark room with lots of turns and side-passages, where all the walls are covered in mirrors - just like the Hall of Mirrors in an old-fashioned fun-fair. Is it true that if someone lights a match somewhere in the room, then wherever you stand in the rest of the room (even down a side-passage) you can see a reflection of the match?
For example, consider an L-shaped room, with the person holding the match standing near one corner. If I stand round the corner, I can see a reflection of the match because a light ray can bounce off the two opposite walls. This is true wherever I stand in the room, so the whole room is illuminated by just one match!
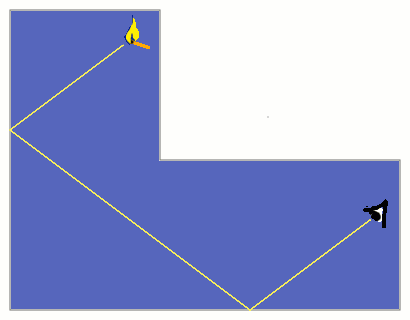
An illuminable room
The question Ernst Straus asked was whether there is any room which is so complicated that there's somewhere you can hold the match which leaves part of the room shrouded in darkness because the reflections can't reach it. Nobody knew the answer until 1995, when George Tokarsky of the University of Alberta in Canada showed that the answer was yes - there is such a room! He published this floor plan of a room with 26 sides - the smallest such room currently known. If the match is held in just the right place, then at least one other point in the room is dark.
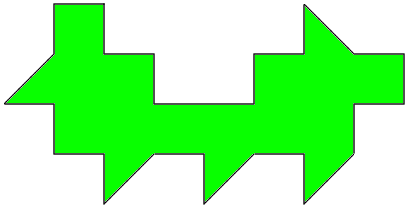
A 26-sided unilluminable room
But a mystery still remains, lurking in a dim corner of our imaginations. With the room Tokarsky found, there is certainly one particular place where the match can be held which leaves part of the room dark; but if you move the match slightly, the whole room is lit up again. Is there a room which is so fiendishly complicated that wherever you hold the match there are some dark spots in the room? Nobody knows the answer...
This problem of course, is a bit like a similar problem to do with snooker. Light bounces off the reflective walls of the room in exactly the same way that a snooker ball bounces off a cushion. (Well, except that the snooker ball eventually comes to a stop because of friction.) So you could imagine this mystery to be about whether you can design a snooker table which is such a strange shape that there are some places on the table from which it's impossible to pot the cue ball, if you're aiming for a pocket which is round enough corners!
There's one more difference between the match problem and the snooker table problem: what happens at sharp corners? A light ray which exactly strikes a corner of the room is assumed not to reflect at all - it just gets absorbed at the join between the mirrors on the adjacent walls. But a ball on a snooker table would always bounce off in some direction.
References
Scientific American, August 96, Unilluminable Rooms