Intriguing integrals: Part II

Share this page
March 2010

Euler's formula for ex

We want to prove the identity

  \begin{equation} \label{eq:euler_ e} e^ x = \lim _{n\rightarrow \infty } \left( 1+\frac{x}{n}\right)^ n. \end{equation}   (1)
If we consider the Taylor series for $e^ x$, on the left hand side we have
  \[ e^ x = 1+x+\frac{x^2}{2!}+\frac{x^3}{3!}+\frac{x^4}{4!}+\cdots \]    
On the right, we can expand out the product using the Binomial Theorem
  \[ \left( 1+\frac{x}{n}\right)^ n = \sum _{i=0}^ n \frac{n(n-1)\cdots (n-i+1)}{i!}\left(\frac{x}{n}\right)^ i = \sum _{i=0}^ n \frac{x^ i}{i!} \frac{n(n-1)\cdots (n-i+1)}{n^ i}. \]    
The coefficient of $x^ i$ equals
  \[ \frac{1}{i!} \frac{n(n-1)\cdots (n-i+1)}{n^ i} = \frac{1}{i!} \frac{n}{n}\frac{n-1}{n} \cdots \frac{n-i+1}{n}. \]    
As $n\rightarrow \infty $ each term here containing $n$ converges to $1$ giving
  \[ \lim _{n\rightarrow \infty } \left( 1+\frac{x}{n}\right)^ n = \sum _{i=0}^\infty \frac{x^ i}{i!}, \]    
or exactly the Taylor series for $e^ x$.

You might think this argument has made somewhat cavalier use of limits. The following argument, adapted from [1, pg 272], is close to Euler's original. He was even more bold in his use of limits!

"Euler unhesitatingly accepts the existence of both infinitely small and infinitely large numbers, and uses them to such effect that the modern reader's own hesitation must be tinged with envy." [1, pg 272]

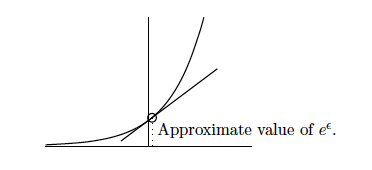
Thinking about $e^ x$ near $x=0$, and its gradient, we see that, for infinitely small $\epsilon $

  \[ e^\epsilon = 1 + \epsilon \]    
This is illustrated above. Let $x$ be any given number, then $N=x/\epsilon $ is infinitely large. So

Reference

[1] C.H. Edwards, The Historical Development of the Calculus, Springer-Verlag, 1979.

Return to main article