
The philosophy of applied mathematics
I told a guest at a recent party that I use mathematics to try to understand migraines. She thought that I ask migraine sufferers to do mental arithmetic to alleviate their symptoms. Of course, what I really do is use mathematics to understand the biological causes of migraines.
My work is possible because of a stunning fact we often overlook: the world can be understood mathematically. The party goer's misconception reminds us that this fact is not obvious. In this article I want to discuss a big question: "why can maths be used to describe the world?", or to extend it more provocatively, "why is applied maths even possible?" To do so we need to review the long history of the general philosophy of mathematics — what I will loosely call metamaths.
What is applied maths?

A stunning fact: the world can be understood mathematically.
Before we go any further, we should be clear on what we mean by applied mathematics. I will borrow a definition given by an important applied mathematician of the 20th and 21st centuries, Tim Pedley, the GI Taylor Professor of Fluid Mechanics at the University of Cambridge. In his Presidential Address to the Institute of Mathematics and its Applications in 2004, he said "Applying mathematics means using a mathematical technique to derive an answer to a question posed from outside mathematics." This definition is deliberately broad — including everything from counting change to climate change — and the very possibility of such a broad definition is part of the mystery we are discussing.
The question of why mathematics is so applicable is arguably more important than any other question you might ask about the nature of mathematics. Firstly, because applied mathematics is mathematics, it raises all the same issues as those traditionally arising in metamaths. Secondly, being applied, it raises some of the issues addressed in the philosophy of science. I suspect that the case could be made for our big question being in fact the big question in the philosophy of science and mathematics. However, let us now turn to the history of metamaths: what has been said about mathematics, its nature and its applicability?
Metamathematics
The long history of mathematics generally lacks a distinction between pure and applied maths. Yet in the modern era of mathematics over, say, the last two centuries, there has been an almost exclusive focus on a philosophy of pure mathematics. In particular, emphasis has been given to the so-called foundations of mathematics — what is it that gives mathematical statements truth? Metamathematicians interested in foundations are commonly grouped into four camps.
Formalists, such as David Hilbert, view mathematics as being founded on a combination of set theory and logic (see Searching for the missing truth), and to some extent view the process of doing mathematics as an essentially meaningless shuffling of symbols according to certain prescribed rules.
Logicists see mathematics as being an extension of logic. The arch-logicists Bertrand Russell and Alfred North Whitehead famously took hundreds of pages to prove (logically) that one plus one equals two.
Intuitionists are exemplified by LEJ Brouwer, a man about whom it has been said that "he wouldn't believe that it was raining or not until he looked out of the window" (according to Donald Knuth ). This quote satirises one of the central intuitionist ideas, the rejection of the law of the excluded middle. This commonly accepted law says that a statement (such as "it is raining") is either true or false, even if we don't yet know which one it is. By contrast, intuitionists believe that unless you have either conclusively proved the statement or constructed a counter example, it has no objective truth value. (For an introduction to intuitionism read Constructive mathematics.)

Plato and Aristotle as depicted in Raphael's fresco The school of Athens.
Moreover, intuitionists put a strict limit on the notions of infinity they accept. They believe that mathematics is entirely a product of the human mind, which they postulate to be only capable of grasping infinity as an extension of an algorithmic one-two-three kind of process. As a result, they only admit enumerable operations into their proofs, that is, operations that can be described using the natural numbers.
Finally, Platonists, members of the oldest of the four camps, believe in an external reality or existence of numbers and the other objects of mathematics. For a platonist such as Kurt Gödel, mathematics exists without the human mind, possibly without the physical universe, but there is a mysterious link between the mental world of humans and the platonic realm of mathematics.
It is disputed which of these four alternatives — if any — serves as the foundation of mathematics. It might seem like such rarefied discussions have nothing to do with the question of applicability, but it has been argued that this uncertainty over foundations has influenced the very practice of applying mathematics. In The loss of certainty, Morris Kline wrote in 1980 that "The crises and conflicts over what sound mathematics is have also discouraged the application of mathematical methodology to many areas of our culture such as philosophy, political science, ethics, and aesthetics [...] The Age of Reason is gone." Thankfully, mathematics is now beginning to be applied to these areas, but we have learned an important historical lesson: there is to the choice of applications of mathematics a sociological dimension sensitive to metamathematical problems.
What does applicability say about the foundations of maths?
The logical next step for the metamathematician who bothers to think about the applicability of mathematics would be to ask what each of the four foundational views has to say about our big question. Discussions along this line have been written by a number of mathematicians and scientists, such as Roger Penrose in the book The road to reality, or Paul Davies in his book The mind of god.
I would like to take a different path here by reversing the "logical" next step: I want to ask "what does the applicability of mathematics have to say about the foundations of mathematics?" In asking this question I take for granted that there is no serious disagreement about whether mathematics is applicable: the entire edifice of modern science and technology, depending heavily as it does on the mathematisation of nature, bears witness to this fact.
So what can a formalist say to explain the applicability of mathematics? If mathematics really is nothing other than the shuffling of mathematical symbols in the world's longest running and most multiplayer game, then why should it describe the world? What privileges the game of maths to describe the world rather than any other game? Remember, the formalist must answer from within the formalist worldview, so no Plato-like appeals to a deeper meaning of maths or hidden connection to the physical world is allowed. For similar reasons, the logicists are left floundering, for if they say "well, perhaps the universe is an embodiment of logic", then they are tacitly assuming the existence of a Platonic realm of logic which can be embodied. This turns logicism into a mere branch of platonism, which, as we will shall see below, comes with its own grave problems. Thus for both formalists and non-platonist logicists the very existence of applicable mathematics poses a problem apparently fatal to their position.
Neither logicism nor formalism is widely believed any more, despite the cliché that mathematicians are platonists during the week and formalists at the weekend. Both perspectives fell out of favour for reasons other than the potentially fatal one about the applicability of mathematics, reasons largely connected with the work of Gödel, Thoralf Skolem, and others. (See Gödel and the limits of logic.)

Is the world inherently mathematical or is maths a construct of the human mind?
The third proposed foundation, intuitionism, never really garnered much support in the first place. To this day, it is muttered about in dark tones by most working mathematicians, if it is considered at all. What is seen as a highly restricted toolkit for proofs and a bizarre notion of limbo, in which a statement is neither true nor false until a proof has been constructed one way or the other, make this viewpoint unattractive to many mathematicians.
However, the central idea of the enumerable nature of processes in the universe appears to be deduced from reality. The physical world, at least as we humans perceive it, seems to consist of countable things and any infinity we might encounter is a result of extending a counting process. In this way, perhaps intuitionism is derived from reality, from the apparently at-most-countably infinite physical world. It appears that intuitionism offers a neat answer to the question of the applicability of mathematics: it is applicable because it is derived from the world.
However, this answer may fall apart on closer inspection. For a start, there is much in modern mathematical physics, including for example quantum theory, which requires notions of infinity beyond the enumerable. These aspect may therefore lie forever beyond the explicatory power of intuitionistic mathematics.
There is one modern idea which could benefit from the finitist logic of the intuitionists: so-called digital physics. It holds that the Universe is akin to a giant computer. The fundamental particles, for example, are described by the quantum state they happen to be in at a given moment, just as the bit from computer science is defined by its value of 0 or 1. Just like a computer, the Universe is based on information about states and its evolution could in theory be simulated by a giant computer. Hence the digital physics motto, "It from bit".
But this world view too fails to be truly intuitionistic and seems to sneak in some platonic ideas. The bit of information theory seemingly posits a platonic existence of information from which the physical world is derived.
But more fundamentally, intuitionism has no answer to the question of why non-intuitionistic mathematics is applicable. It may well be that a non-intuitionistic mathematical theorem is only applicable to the natural world when an intuitionistic proof of the same theorem also exists, but this has not been established. Moreover, although intuitionistic maths may seem as if it is derived from the real world, it is not clear that the objects of the human mind need faithfully represent the objects of the physical Universe. Mental representations have been selected for over evolutionary time, not for their fidelity, but for the advantage they gave our forebears in their struggles to survive and to mate.
Created in the image of mathematics
Formalism and logicism have failed to answer our big question. The jury is out on whether inuitionism might do so, but huge conceptual difficulties remain. What, then, of Platonism?

Galileo Galilei, who believed that the world was written in the language of maths, facing the Roman Inquisition for proclaiming that the Earth moved around the Sun. Painting by Cristiano Banti.
Platonists believe that the physical world is an imperfect shadow of a realm of mathematical objects (and possibly of notions like truth and beauty as well). The physical world emerges, somehow, from this platonic realm, is rooted in it, and therefore objects and relationships between objects in the world shadow those in the platonic realm. The fact that the world is described by mathematics then ceases to be a mystery as it has become an axiom: the world is rooted in a mathematical realm.
But even greater problems then arise: why should the physical realm emerge from and be rooted in the platonic realm? Why should the mental realm emerge from the physical? Why should the mental realm have any direct connection with the platonic? And in what way do any of these questions differ from those surrounding ancient myths of the emergence of the world from the slain bodies of gods or titans, the Buddha-nature of all natural objects, or the Abrahamic notion that we are "created in the image of God"?
Indeed, the belief that we live in a divine Universe and partake in a study of the divine mind by studying mathematics and science has arguably been the longest-running motivation for rational thought, from Pythagoras, through Newton, to many scientists today. "God", in this sense, seems to be neither an object in the space-time world, nor the sum total of objects in that physical world, nor yet an element in the platonic world. Rather, god is something closer to the entirety of the platonic realm. In this way, many of the difficulties outlined above which a platonist faces are identical with those faced by theologians of the Judeo-Christian world — and possibly of other religious or quasi-religious systems.
The secular icon Galileo believed that the "book of the universe" was written in the "language" of mathematics — a platonic statement begging an answer (if not the question) if ever there was one. Even non-religious mathematical scientists today regularly report feelings of awe and wonder at their explorations of what feels like a platonic realm — they don't invent their mathematics, they discover it. Paul Davies goes further in The Mind of God, and highlights the two-way nature of this motivation. Not only may a mathematician be driven to understand mathematics in a bid to glimpse the mind of God (a non-personal God like that of Spinoza or Einstein), but our very ability to access this "key to the universe" suggests some purpose or meaning to our existence.
In fact, the hypothesis that the mathematical structure and physical nature of the universe and our mental access to study both is somehow a part of the mind, being, and body of a "god" is a considerably tidier answer to the questions of the foundation of mathematics and its applicability than those described above. Such a hypothesis, though rarely called such, has been found in a wide variety of religious, cultural, and scientific systems of the past several millenia. It is not natural, however, for a philosopher or scientist to wholeheartedly embrace such a view (even if they may wish to) since it tends to encourage the preservation of mystery rather than the drawing back of the obscuring veil.
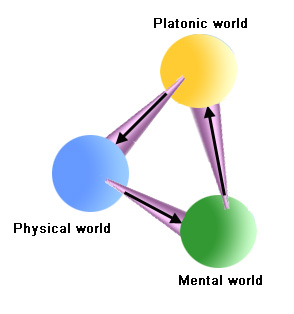
Penrose's three-worlds diagram.
Roger Penrose has most lucidly illustrated some of this mystery with a three-worlds diagram. The platonic, physical, and mental worlds are the three in question, and he sketches them as spheres arranged in a triangle. A cone then connects the platonic world with the physical: in its most general form, the diagram shows the narrow end of the cone penetrating the platonic world and the wider part penetrating some of the physical world. This is to show that (at least some of) the physical world is embedded in at least some of the platonic world. A similar cone connects the physical to the mental world: (at least some of) the mental world is embedded in the physical world. Finally, and most mysteriously, the triangle is completed by a cone from the mental to the platonic world: (at least some of) the platonic world is embedded in the mental world. Each cone, each world, remains a mystery.
We seem to have reached the rather depressing impasse in which none of the four proposed foundations of mathematics can cope unambiguously with the question of the applicability of mathematics. But I want you to finish this essay instead with the feeling that this is incredibly good news! The teasing out of the nuances of the big question — why does applied mathematics exist? — is a future project which could yet yield deep insight into the nature of mathematics, the physical universe, and our place within both systems as embodied, meaning-making, pattern-finding systems.
About the author

Phil Wilson is a senior lecturer in mathematics at the University of Canterbury, New Zealand. He applies mathematics to the biological, medical, industrial, and natural worlds.
Comments
Anonymous
Cute quotation from Knuth - however Knuth was wrong. Brouwer would have no problem with the statement 'either it is raining outside or it is not raining outside' because he knew you could go and look. He did not insist on your actually looking. He had a problem with things like König's lemma - not quite the same. I have a problem with things like König's lemma too, but in the great scheme of things it is not very important.
PhilWilson
Well, of course Knuth's comment was made in jest, and if it does slightly miss the mark the in terms of its accuracy it is bang on target in terms of capturing the discomfort many mathematicians feel about Brouwer's perspective.
Anonymous
Would you really need three realms (mental, physical, platonic) to answer the question?
I am no mathematician but wouldn’t it be a plausible model to assume that the mind (the mental realm) reflects those properties of the physical realm that were important to our survival at some evolutionary time point? This would obviously include rules like cause and effect and the natural numbers. If these inbuilt rules were correct, they could by inference (likewise a mental rule reflecting properties of the physical world) be used to extrapolate more and more of the rule framework of the physical realm. This would also explain why applied mathematics (in the chosen definition) can describe counter-intuitive physics, i.e. it works beyond the limits where the mind correctly reflects the physical world. This model obviously is still platonic but I do not see that it needs three realms.
Daniel
PhilWilson
Daniel, you make some good points which strike at the heart of part of what this article is about. But let me try to unpick a little of what you say to see if I understand you correctly.
You are worried about needing three realms. The two realms you seem to affirm are the mental and the physical. Even this is a leap, actually, since a pure materialist would say that the mental realm can be reduced to the physical, that our language for describing the mental realm is a kind of shorthand for what is "really" happening on a physical level. I disagree with this perspective, and you can find coherent book-length arguments against it in pop science books by Robert Laughlin (A Different Universe) and Stuart Kauffman (Reinventing the Sacred), and elsewhere. So you and I agree that there are at least two realms, the mental and the physical. While neither is reducible to the other, they are not independent either.
So the real question which I think you are asking is, "Do we need to posit the existence of a platonic realm." Well, to some extent, my article is all about this very question! But while I think that there is a lot of currency in your evolutionary argument, it doesn't seem to me to satisfactorily address all of the issues. As I said in the article, the evolutionary pressure would have been on (a) survival, and (b) mating. While understanding cause and effect, and being able to count distinct objects, and so on, would presumably have helped, it doesn't seem obvious to me that the leap you make to an evolutionary explanation for advanced mathematical ability is a necessary one, or one well-founded in the hypothesis of mathematics being somehow an encoding of the physical world in the mental. However, I think that John Barrow is a fan of this idea - I remember him discussing the work of Rosen in this context in his book "Impossibility". I'm afriad I can't get the reference for you now because I am in quake-shattered Christchurch, and I cannot get in to my office building!
As far as I can tell, an evolutionary approach to the question of the existence of applied mathematics does not address its ability to work in counter-intuitive realms, its apparent timelessness, its felt sense of independence, its coherence, its ability to explain the physical universe, or even our ability to find the universe comprehensible at all!
Anonymous
Thank you for answer Phil. If you don’t mind, I would like to defend my evolutionary explanation a bit (neglecting the other parts of your answer for the moment).
In a way, there is lot of applied mathematics in biology – a spectacular example would be echolocation in bats or dolphins but there are many others. Admittedly, those are mostly specialized systems and not all-purpose processors like the human mind, but I still think they illustrate that advanced mathematical abilities can be acquired by evolution.
The human ability to apply mathematical rules and – probably more important for real mathematics - to combine them freely might be a chance product of their coexistence in the same mental apparatus. The chance element is of course a weak point here, but then again, chance is an essential part of evolution and there are quite a few examples where unsuspecting inventions have been coopted by evolution for some unforeseen purpose (e.g. feathers for flight).
I would also like to outline a possible explanation for the ability of mathematics to work in counter-intuitive realms which is in line with an evolutionary approach: The rules used to interpret the physical world (or to manipulate it) would be more useful the more general (and accurate) they were. I would further posit that intuition is based on what we can directly experience through our senses or actions but those experiences are constrained by the implementation of our physical body. However, mental rules that are able to interpret data accurately in the working range of our body do not necessarily fail where the body fails, in the same way that the validity of a temperature scale does not stop where a particular thermometer reaches the end of its dynamic range.
regards,
Daniel
PhilWilson
Hi Daniel.
The great thing about a conversation like this one is that since no-one knows the answers we can have a lot of fun defending different viewpoints! I must admit, however, that I don't really have a strong opinion on this matter one way or the other, so it is wonderful for me to find myself attracted to what you are saying - and then to try to play devil's advocate with your ideas.
You say that there is a lot of applied math in biology. With my devil's advocate (tail?) on, I would say that really you are only allowed to say that that which we call (applied) math can be found in biology. I mean to say, that if you are saying that echolocation and the like actually are mathematics, then you already have a platonic view, to some extent. You're saying that there is an embodiment of an abstract
principle, and that the universe (or the bit of it identified with the bat) is somehow solving equations to work out the source of an echo. This may be true, or it may be true that our mathematics is (as I think you want to say) a language, honed by evolution, for describing patterns, some of which can be found in nature. So I think that there may be a contradiction in your advocacy of "finding math in nature" and "math being an evolutionary product". If it is not a contradiction, then it may instead be a circular argument, in that you say (a) math evolved, because (b) math is in nature, because (a) math evolved. The problem with this is the same as when I pointed out that to say that the bat is "doing" math (unconsciously, presumably) is to assume that that which we call math is identical to how the universe works. And this is unknown.
You also point to what are known as Darwinian preadaptations: incidental features of an organism which were not directly selected, but which later turned out to be advantageous in another, unforeseen way. Your example of feathers, which are hypothesised to have evolved for warmth and possibly plumage display, but which later enabled controlled flight, is a good one. You then seem to want to argue that math was selected
for its utility in the (energy? length? speed? mass?) range of our bodies, and then had a Darwinian preadaptation utility in more advanced forms of math. First, I'm not convinced that being able to do math consciously has a utility of any form. We may certainly need it subconsciously, like the bat, or like a recent study of a certain Brazilian tribe which showed that they have excellent spherical geometry intuition but not even natural numbers, arithmetic, or sense of time or object persistence. Secondly, even if the first point were true, we still come back to the question of why we should be able to extend math beyond the range of our bodies and still find it has utility. Just because Darwinian preadaptations exist doesn't help to explain our Big Question - in fact, it tells us almost nothing about it. Why should math extend this way? Why should the universe be comprehensible at all?
As a footnote of sorts, Stuart Kauffman has written a lot about the mathematical structure of evolution, and John Barrow has been one of the brave few to raise the question of the mystery of the universe's comprehensibility - and potential limits on our own understanding of it.
Anonymous
Very interesting post. My 2 cents:
Why maths can be applied? Whatever one's approach to understanding our universe is, we have to agree that, as it exists, there needs to be some notion of "inner coherence" (call it physical laws or whatever). On the other hand there is maths which in my understanding is no more (no less) than a grammar whose associated language is all that is coherent (I should extend here but will not). To me, physics study this particular "coherent reality" and maths study any possible "coherent reality". N.B. a topological variety is a potential reality to me as long as it is coherent and can be described "grammatically", so to speak.
Regards,
Pedro Pablo
PhilWilson
Hi, Pedro, and thanks for your comments.
Your suggestion that maths studies structure, or pattern, or "coherent realities" of all forms, and that physics is one of these, and that the universe in which we live is another, is certainly popular among mathematicians and mathematical scientists. But it does seem to displace the central question in favour of even harder questions! These include the following. Why should physics be coherent - why not be too difficult for us to understand, or not coherent at all? Must the universe be coherent for life of any kind to arise? Is there a hidden anthropic principle in this line of argument, requiring a vast array of unknowable parallel universes?
You might be able to sense that while I find your "answer" appealing, I also think that it raises harder and more troubling questions!
Phil
Anonymous
I'd like to start this comment with a question:
When you talk about a "Platonic world" you mean a distinct, clearly defined world, completely different from the physical world, or is it a short-hand term used to describe the underlying order and internal coherence of physical phenomena?
If the former, I don't think a discussion is possible, because: where do you draw the line? As you very well put it in your article, a hypothesis such as that would be, for all practical purposes, virtually indistinguishable from a religious one, because it entails assuming all order and coherence are derived from a world to which we cannot have access empirically, and is thus impossible to prove scientifically.
As Albert Szent-Györgyi once put it "Thus Aristotle laid it down that a heavy object falls faster than a light one does. The important thing about this idea is not that he was wrong, but that it never occurred to Aristotle to check it."
My point being that the empirical aspect of scientific inquiry is just as important as the logical/mathematical one, as Galileo himself so aptly proved.
If the latter, then I don't see why the formal justification of mathematics would be wrong, provided you assume that the symbols (and their possible relations) are adjusted to coincide with physical phenomena, and, to a great extent, are able to predict them.
According to this definition, mathematics would ultimately be a scientific enterprise concerned with the coherence and consistency of sets of symbols and relations we invent as we come across new phenomena, and, since these relations can be studied without empirical analysis, mathematics can go further than other, more heavily empirical sciences, which allow it to predict the behavior of phenomena we have yet to come across (assuming these phenomena follow the same underlying principles as those encountered before).
I hope the above made sense, and that I did not misunderstand any of the concepts you used.
Regards,
Rodrigo
PhilWilson
Hi Rodrigo.
Your comment was very coherent, and absolutely right in identifying a need for clarity concerning what is meant by a "platonic realm". Perhaps I wasn't clear enough in the article. Anyway, of the two options you mention it is the former: a completely distinct, transcendent, non-physical realm.
This definition raises the problem that you and I have mentioned: apparent difficulties with empirical investigation. But I would like to ask you what you mean by "prove scientifically". Are you working in a Popperian scheme? The trouble with doing so is that it itself is more of an over-arching classification (science/not-science), or perhaps a working definition, rather than an actual established, provable fact, or even something open to empirical investigation. In fact, to the extent that it is open to empirical study, it is plainly falsified in the working lives of almost every scientist. Alternatives, such as Quine's holism, are perhaps closer to the everyday experience of most scientists.
Either way, both schemes may allow for an empirical test of the platonic hypothesis, if I may call it that. Because after all, although the platonic realm is distinct and independent of the physical realm, the one influences the other (I'm talking from within the hypothesis, you understand). So it is possible that someone may be able to come up with a (falsifiable?) statement about the existence of the platonic realm which can be investigated with empirical tools of the physical realm.
As for the second option you mention, that the platonic realm is a kind of shorthand for a quasi-empirical science of mathematics (a science of pattern, essentially), this is not what I meant. It is a perspective which has its advocates, but which few mathematicians or mathematical scientists find appealing (this doesn't make it wrong, of course). There are serious implications of such a viewpoint for the entire scientific program.
Anonymous
It was precisely with Popper's scheme in mind (and, to a lesser extent, Hume's simpler "How do you know?") that I wrote my previous comment, although, as you said, very few scientists work with said scheme in mind, given its obvious impracticality and lack of precisely defined methods.
I didn't even know Quine's holism existed, thank you for providing me with the link.
As for the rather liberal use of the words 'empiric' and 'scientific' in my previous post, it was because I wanted to stress the importance of the 'applied' part of this article's title.
When it comes to applicability, the old engineering adage says it all:
"In engineering, you're not done when there's nothing more to add, but when there's nothing more to take away".
When developing a philosophy aimed at giving some sort of justification for the applicability of abstract mathematical principles to concrete, physical reality, shouldn't the prospective scientist/philosopher/logician guide his reasoning by that same adage?
I can't think of a more gross violation of the most basic principles that guide applied mathematics than literally inventing a whole world to justify itself.
I apologize if this all sounds rather vague, but I lack the preparation or background to give a more rigorous and detailed defense of my case, assuming I have one at all.
P.S. I'm currently trying to study this subject on my own using Mendelson's "Introduction to mathematical theory", Pierce's "Introduction to information theory" and Devlin's "Information and logic".
Are there any other good books I could use to study this, preferably mathematical in format?
Regards,
Rodrigo
PhilWilson
Hi Rodrigo,
First, my apologies for my slow reply. I am currently attending a workshop at the American Institute of Mathematics in California and have been working very hard!
I like the idea of stopping when there is nothing more to take away. It reminds me of the adage about how Michaelangelo went about his sculpting. I note also, however, that this is logically irreducible to an algorithm: if you are only "subtracting", then how do you define the initial condition?
Let me ask you, however, why it is that the philosophy of a branch of science should follow the same rules as the science itself?
Don't worry about what you feel may be vagueness: no-one knows the answers to these deep questions we are discussing, so we all of us can have as much fun as we like in discussing them! Your preparation for doing so may in fact be much better than my own, which has largely grown organically through reading popular science and being fortunate enough to have been able to bend the ear of talented and indulgent philosophers.
Have fun!
P
Anonymous
I take the view that mathematics is simply an extension of language, made more precise by use of abbreviated symbolism. This doesn't seem to be the same as either "formalism" or "logicism", though it has aspects of both. on this basis mathematics is language developed to describe "structures" from simple things like sets up to complex scientific theories. This approach makes no presumptions about the nature of what is being described, i.e. the world, other than that it can be described. Mathematics is indeed inadequate to describe many aspects of the world. It is effective in areas where elements can be clearly defined. On this view "pure" mathematics is an outgrowth from this practical process of developing methods of mathematical description, abstracting it to describe formalisms not yet having any application in the world other than to mathematical ideas themselves.
Regards
George Jelliss
PhilWilson
Hi George, and thanks for your comment.
If mathematics is a language, why should it have the precision it appears to do? Why should the universe be describable and comprehensible at all? If it is abstracted from the world why should we be able to develop it in non-world realms ("mathematical ideas themselves" as you put it)? Why are we able to make abstractions, and in a precise way?
I realise that in asking such questions I am in a way moving beyond your points and in some way beyond the point of the article. But I am trying to emphasise that in trying to "solve" this question, we employ assumptions that raise larger, wider questions.
This shouldn't be taken as an excuse not to raise such questions! Indeed, I was trying to inculcate a sense that there is still great mystery in the world.
Cheers,
P
Anonymous
It seems that different languages have different levels of precision.
English, with its notorious ambiguity, jargon, idiom, &c seems to be very imprecise. German, with its very specific words for very specific things and a high level of succinctness, seems much more precise.
Could it be that mathematics as a language is just the language that is the most precise that we know of currently?
PhilWilson
It is possible that math is "just" the most-precise currently-known language. But this doesn't answer any of the questions we have been raising, although it does possibly reframe the overall question to be something more like "how accurately are we able to describe patterns and is math the optimal language for so doing?".
I also note that precision is something, but not everything. In human languages, it is possible to convey things by ambiguity and choice of words that it is impossible to encode formally in precise language. Understanding what "Feeling under a cloud" means takes a whole lifetime of experience. Languages are embedded in culture and personal experience, such that semantics require that context even if syntactics do not.
Vaguer languages such as Japanese, can convey much subtler meanings than English or German. There is possibly a way to maximise a weighted sum of the creativity which ambiguity allows and the accuracy which precision allows.
Anonymous
Reading your article a radical thought came to my mind.
Being a mathematician or a physicist (like myself) we are used to treating mathematics as the ultimate tool in understanding nature. We develop mathematical formalisms that describe nature and then marvel at how well mathematics is suited to describe our subject of interest. But is mathematics really so universal as we make it out to be, or do we just see it in that light because we are so used to it?
The foundations of mathematics evolved in our ancestors for the need to count things in order to have to survive. Mathematics was used for centuries by the Babylonians, Egyptians, Greeks and other highly developed cultures. But it took the genius of a Newton or Leibniz to develop differential calculus and to be able to properly describe and object's motion when a variable force acts upon it. This formalism uses infinities and infinitely small intervals and is quite a long way from the simple act of counting.
So my answer is: The world can be understood mathematically, only because we develop the mathematical tools that allow us to understand it. Constructions, like differential calculus, do not follow inevitably from the basic principles of mathematics, but only from our need to describe nature. But this reverses the dependency. The universe is not inherently mathematical, but mathematics is constructed to be universal.
Holger
PhilWilson
Hi Holger.
Yes, this seems to me to be very good and valid point. We identify a subset of real-world experiences all of which are well-described by mathematics and then step back and marvel at the "universality" of mathematics. I'm reminded of this.
Nevertheless, there remains the question of exactly why we have access to a "language" (to take George's point below) which we can (perhaps) abstract from (a subset of) the world, play with in our minds, and then accurately re-apply to (a subset of) the world. We take this for granted, but, as I am trying to establish, this fact is stunning and (perhaps) non-obvious.
P
Anonymous
A mathematics fan myself (and a self proclaimed philosopher :), though everyone is a philosopher in their own rights), I do believe in the universality of mathematics but feel that mathematics is just an aspect of reality and not an all pervading foundation. Mathematics can be seen as a language of describing certain kinds of correlations between objects, events etc. So whether mathematics is all pervading depends on whether
i) Everything in this world is correlated
ii) Can mathematics describe all correlations in this world.
Irrespective of whether i) is true or not, I think its not too difficult to construct examples to show ii) is not true. As many would already have thought of, feelings like love, etc cannot be explained by mathematics. (Reminds me of this valentine's day quote by H.L. Mencken: Love is the triumph of imagination over intelligence).
On a different note, some of you may enjoy this tangentially related blog post of mine: http://janakspen.blogspot.in/2011/05/infinite-soul-and-bit-of-discrete-…
I can say this to extend Holger's comment: Most of the things or phenomena around us can be measured (if not objectively, then perhaps subjectively), and measurement is very much mathematical. So one may feel that everything is mathematical. But in reality, measurement or correlation between entities is just one aspect of their existence. So its wrong to say mathematics can describe or is the basis of everything.
I did enjoy aspects of this article .. this has become one of my favorite sites on the internet :)
Anonymous
A nice point made by Anonymous and PhilWilson. It is true that relations of the heart are not the same as relations of numbers. People not believing this no mathematical equation can predict what gift gift your valentine will want on this Valentine's Day:) Having said that I think there is no end to the argument whether emotions like love are matters of the heart or produced mathematically controlled events in the brain. And now on a lighter note, for all those who believe that mathematics can describe everything including love, two proofs:
Firstly, some gems of quotes from this page: mathematical love quotes for valentines day:
* I'm a fraction of what I should be without you... you complete me.
* You and I are living proof one plus one equals three, happy valentines day to a dad to be
Now this is one great use of applied mathematics, right? Caution: Use the above quotes only if your valentine is a geek :)
Second proof: these beautiful love equations from http://www.walkingrandomly.com/?p=2326
Also do read this interesting article on valentine programming apparently people are researching how mathematics can explain lost love and what not. Applied mathematics is a whole ocean out there.
Anonymous
I appreciate the appeal of your question. I was a philosopher before I began seriously studying mathematical physics, and even though I had already considered the basic questions, I found myself struck anew by the regularity with which nature has been found to conform to the most abstract imaginative leaps of rare minds.
Nevertheless I believe that the question is more to be dissolved than solved. It is akin to the question of how language can "latch onto reality" (the answer to which lies in understanding our own behavior).
Some of the questions you ask (e.g. "why should the universe be comprehensible at all?") may be illuminated by evolutionary considerations. An amoeba responding to being poked is already "comprehending" (a tiny piece of) the world in a sense, and higher animals display a finely-tuned set of useful, reality-conforming behaviors requiring discrimination and goal-pursuit. Their mental constructs "conform to reality" because (and to the extent that) they worked in the past (and others didn't). (And of course we know that past results are no guarantee…)
At the same time, it helps to be aware that we have no idea what it would mean for the universe NOT to be AT ALL comprehensible. We can contrast understanding here with ignorance there, or complete understanding with partial understanding; but we have no concept of a world without any understanding at all. So this is why I say that your question is at bottom a pseudo-question (which may nevertheless be very educational and fruitful along the way to being dissolved).
I don't think your 4 positions are exhaustive. I'd say you're missing a position of Realism, which says that mathematical structures inhere in nature, without requiring any "independent realm" to give them "reality". It is nature that gives them reality. For we cannot conceive of a nature without mathematical structure--without unity and multiplicity, spatial orientations, boundaries, sets…
And by the way I'd like to add this open-ended list of mathematical categories to your account of the natural genesis of mathematics out of counting. Not just counting, but geometrical intuition and discrimination of natural sets seem to me to be equally grounded in an evolutionary account.
You say that a theistic picture is tidier than the others, but are afraid of embracing religious baggage. I do think that a Spinozan patheism is a viable solution, as long as you realize that it is really nothing more than a counterweight to the kind of materialism that denies any structure or intelligibility to the world independently of humans. To call the world "divine" in this sense is just to acknowledge that before intelligent beings, the world already had intelligible structure, and contained the potentiality for the causal generation of intelligence.
PhilWilson
Hi, Anonymous, and thanks for a great comment.
I am currently attending a workshop at the American Institute of Mathematics and am very busy indeed, so I am afraid that your answer will not get the full and in-depth response which it merits. My apologies.
I think that your raise some excellent points. While I am very receptive to the evolutionary approach - and while I think that it can be extended to provide a definition of mathematical beauty in terms of maximum fecundity - it leaves me thinking "and yet, and yet . . .". It simply does not, to my mind, address the thorny issue of why completely abstracted mathematical ideas should turn out to be so central to our understanding of the physical universe.
You are correct to point out that we have no notion of what an incomprehensible universe would be like. This does not answer the question of why our universe is comprehensible and, as far as I can see, does not even "dissolve" the question or render it a "pseudo-question. (I like the notion of "dissolve" vs solve, by the way!)
I don't understand what you mean by nature "giving reality" to mathematical notions. This seems to avoid the question.
I am only afraid of embracing theological baggage to the extent of claiming that it answers beyond doubt the question before us. I am quite happy to load that baggage onto my life-train for other reasons, reasons which tend to subjectively exclude a Spinozan pantheism. I do agree with you that the potentiality for intelligence evidenced by our existence is an awe-inspiring aspect of the universe.
Anonymous
First, I think it's great that you're taking the time to answer comments. It's rare that authors take full advantage of the conversational potential the internet allows, as opposed to more traditional forms of publishing.
I consider myself a philosopher with only an elementary understanding of mathematical foundations, but I've always found Godel's use of the liar's paradox to undermine Bertrand and Whitehead's Principia especially brilliant. I tend to be drawn to subversive intellectual accomplishments more than straightforward constructive projects. Though I also greatly respect the work of those like Gell-Mann and Feynman who have worked on the nuts and bolts of the Standard Model, which is probably humanity's greatest mathematical project.
I'm not a huge fan of mereology (http://plato.stanford.edu/entries/mereology/), but for a while I've felt that it's central occupation, the relation of parts to wholes, is the same problem underlying metamathematical concerns. Things like, what constitutes a unit? Does the property of divisibility only inhere within some objects, or all of them? In reference to math, we could ask these questions more like, how do we bound objects in order to pick them out for counting? How do we maintain the identity of an object after it is unbound into parts or fractions? And how do those fractions become independent units themselves?
Seems like the question of how math is applicable to the world has to grapple with these types of questions at some point. If only because I place our denoting of physical objects as units prior to our employment of those units for counting. I'm only basing that on evolutionary assumptions, but I don't think it's a stretch as far as assumptions go.
My usual initial response to these questions is that we've adopted a method of differentiating units based on what we discern to be separate objects using visual perception. As it seems the dominant human sense, and especially tuned to delineating the edges of macroscopic objects. I'm certainly not the first to suggest that if we used a different faculty than vision for getting around in the world, then our math, as well as a lot of other things, might be quite different. Thus, we're left with a kind of chicken-and-egg situation. Does our visual field separate objects because objects really are separable (and not necessarily just at our normal scale of perception), or do we think of objects as separable because we've had such success with a visual system that stresses the distinction between objects?
Just one more thing. I take the problems of units to be the inverse of the problems of infinity. Instead of just asking how can there be an endless succession of things, we might also ask how a thing can be separate from everything else?
PhilWilson
Hi Anonymous.
I was bowled over by your comment, and not just because of the praise at the beginning. (Answering questions here has been a delight because I do so enjoy discussing such topics. I'm also fascinated that a lot of the questioners appear to be adults, on this site aimed at high school kids.)
Your comment was so deep that I barely know where to begin. I'm afraid that I cannot answer it in the way it deserves as I am currently in Palo Alto, attending a workshop at the American Institute of Mathematics and am very busy indeed.
I think that you have identified a very deep and important aspect of the scientifico-mathematical enterprise which is certainly a part of what I was discussing here, although not something I had emphasised in the article. How do we define our basic unit? This seems to me to be (a) partly arbitrary, (b) non-algorithmic, and (c) always wrong, although hopefully useful.
It is almost certainly impossible to define the "edge" of a human on a quantum scale, or even an atomic scale, yet this "unitisation" of human-scale objects lies at the heart of what so many consider to be "natural" about the natural numbers. So I certainly agree that there is something about our visual perceptions which define units, and hence the natural numbers, and hence counting - we discretise the world and call it natural. This only seems to deepen the mystery of why a system of thought which develops from such crude beginnings should be able to uncover the depth of quantum mechanics and more besides.
I would be keen on discussing these matters in a more formal way, so do please feel free to contact me at dr dot phil dot wilson at gmail dot com
.mau.
Hi Phil!
I may be dead wrong, but I thought that Intuitionists do not accept at all actual infinite in proving theorems: the only kind of infinite they would accept is the potential one (like in Euclid axioms: the line is not infinite, it may be prolonged at wish). Is it true, or constructivism is only a subsect of intuitionism?
PhilWilson
Yes, I think that's basically correct, although there are different kinds of intuitionism and constructivism, so I suppose the answer will depend upon to whom you are speaking. For example, you have the option to study constructive objects with classical logic, classical objects with intuitionistic logic, and other combinations. There is also the freedom in which axioms you have in your bedrock intuitionistic logic.
I'm not particularly clear on all of these distinctions myself. I wrote another article on Plus about this sort of thing, and my distinguished intuitionistic colleague Douglas Bridges thought it was OK so you might want to take a look. But he also said that some points were not properly developed so take it only as a starting point!
.mau.
It's something of a surprise that Brouwer, after proving this theorem, decided to start constructivism. Or maybe it was just a logical reaction :-)
Thanks!
PhilWilson
Hi .mau.
I think that Brouwer was already beginning to reject non-constructive methods before he developed his fixed-point theorems, and indeed some of these ideas were swirling around the mathematical zeitgeist at the time. But who knows what it was that triggered his formulation of a mini-revolution? These things are a product of a creative free will, I would say, and are thus somewhat unpredictable.
Anonymous
why?
Mathematics studies structures and is the language in which we can talk about these beings in a rather precise way (define them, express their properties, behaviour,...).
If we take a simplistic view of the universe, we could say that it is made of lego bits. These bits happen to fit with each other or can create new bits when something happens to them. These can be put together to form structures (or maybe they are structures already but where do we start?), and we have an increase of complexity that after some time gave rise to things like us.
There may be universes where these bits can not be put together, or the structures they form are more unstable than in ours. Since structures can be talked about with Mathematics, thus it appears as Mathematics can explain our universe. I say appears because it is a byproduct of the original structures and because OUR Mathematics does not really study all possible structures.
The fact is that we do a very human type of Mathematics. If you want me to put a number on it, I would say that with today's Mathematics we study less than 1% of what is out there. We are mostly interested in "nice" structures (that appeal to our sense of beauty). For example, a Ring in which lets say multiplication is associative for all its elements (otherwise it would not be called a Ring) is nicer than a "Ring" in which multiplication with all elements but one (or two, three...) is associative.
Why is it nicer you ask? Well, for starters it is easier to define, easier to find examples to work with and behaviour is more predictable. Just try to find an example of the other "Ring" or try to prove that such structure does not exist (there you have a life work).
So far we have been lucky that our "nice" Mathematics can explain so much of the universe. But if we find things we can not explain, then it becomes very interesting: Is it because we have not developed Mathematics enough? Or is it because there are structures that can not be explained with Mathematics at all? In the later case, are there universes out there that work on such structures? Is ours one of them and we have not realized it?
There is surely a limit to what our "nice" Mathematics can explain. What do we do when we get there? Well, we can try to evolve faster and become something better at math :)
Anonymous
Hi all. I've just recently written about this topic in my blog, and I'd be really interested in hearing what you think. http://bzaharatos.wordpress.com/2012/05/17/what-is-applied-mathematics-…
Chris G
Why should I trouble to point a finger at my sheep as they pass through a gate going one, two, three ... and end up at two hundred and seventy-nine? Because while a flock that size looks pretty much the same from week to week, counting 279 one week, 274 the next and 268 the week after tells me something's going on that requires my attention. The flock may look the same, but the counts 279 274 and 268 don't, their difference is immediately apparent.
Smart eh? Who thought that one up? Not Russell and Whitehead or Plato, or any of the other thinkers named in the article. But I'd be interested to read any of them who at least made a utility like that central to their discussion.