
Plus Advent Calendar Door #3: Watch your middle!
Here's a little game to play. Take the interval from 0 to 1, including the end points. Now delete the middle third of this interval, from 1/3 to 2/3, but without deleting the two end points 1/3 and 2/3. Then delete the middle thirds of the two intervals that are left, [0,1/3] and [2/3,1], again without deleting the end points. Keep going, deleting middle thirds ad infinitum — well, you can't really do this in practice, but you can imagine it. What will you be left with in the end?

Seven steps towards C.
Let's call the set of left-over points C. Clearly, C contains the end points of middle third intervals because these don't get removed: 0, 1, 1/3, 2/3, 1,9, 2/9, 7/9, 8/9, and so on. But other points are left too. For example, 1/4 does not get removed at the first step because it's less than 1/3, it does not get removed at the second step either because it's bigger than 2/9, and so on.
Does C contain any intervals at all? It's pretty easy to convince yourself that it doesn't: any line segment, no matter how tiny, will have been broken up by having its middle third removed. C is what's called totally disconnected because all its connected components are just points.
But these points are not isolated. If you draw a little circle around any point x in C, then no matter how small the circle, it will contain other points of C. For example, take the point 0. Arbitrarily close to it there is a point of the form 1/3k for some k, which is in C because it's the end point of a middle third interval. The fact that every point has other points arbitrarily close to it makes C into a perfect set.
All this means that C inhabits a strange world between dimensions. It's more than a collection of isolated points, which has dimension 0, and less than a line, which has dimension 1. If you calculate its Hausdorff dimension, which is an extension of our usual concept of dimension, the answer is ln(2)/ln(3)=0.63, which is between 0 and 1 as expected.
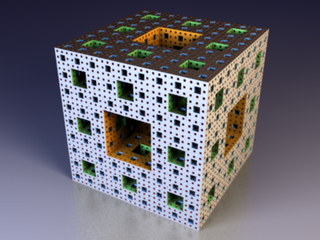
The Menger sponge. Image: Niabot.
The set C is also self-similar. For example, if you take the part of C contained in some tiny interval [0,1/3k] and blow it up, what you get looks exactly the same as C itself. Being self-similar and having a dimension that's not a whole number makes C into a fractal.
C has a name too: it's called the middle-thirds Cantor set, after Georg Cantor, who wrote about it in 1883, although it had previously been discovered by Henry John Stephen Smith. Cantor was one of the first people to give a rigorous description of a fractal, though the term "fractal" was not coined until almost 100 years later by Benoit Mandelbrot.
Cantor sets often turn up in mathematics when things are being broken up according to the same recipe ad infinitum. For example, you can create a similar construct in two dimensions by dividing a square up into nine smaller squares and repeatedly removing the middle one. The result is called the Sierpinski carpet, which has a Hausdorff dimension of ln(8)/ln(3)=1.892. The three-dimensional analogue is the Menger sponge.
You can read more about Cantor sets and fractals in
- How big is the Milky Way?
- Measure for measure
- Unveiling the Mandelbrot set and
- Non-Euclidean geometry and Indra's pearls
Return to the Plus Advent Calendar