
What's so special about special relativity?
Mention Einstein and most people will be intimidated, assuming that they would need a degree in advanced physics to understand his work. But in fact the beauty of Special Relativity lies in its simplicity, which makes it one of the best-kept secrets in all of science.
Common sense?

Now where's that ball?
Imagine you're driving along the road at 50 miles per hour. Just for fun, you decide to throw a ball out of the car. If you throw it straight forwards at 10 miles per hour, how fast will it be going?
Your answer is probably that to you in the car it would appear to be travelling at 10 miles per hour, but that to a pedestrian standing on the pavement it would appear to be travelling at 60 miles per hour.
Now suppose that, instead of driving a car at 50 miles per hour, you are flying a spaceship at 50,000 miles per hour, and instead of throwing a ball out of the window, you allow a light particle to escape, travelling in the same direction as you. (The speed of light in a vacuum is normally denoted c, and it is about 670.76 million miles per hour.)
How fast is the particle of light travelling?
Most likely you would work out the answer the same way as for the car and the ball, and say that from your point of view on the spaceship the speed of the particle of light was 670.76 million miles per hour, whereas from the point of view of someone stationary (with respect to the most distant stars) it would be travelling at c+50,000 miles per hour.
These are the answers Newton would have given, and he was one of the greatest scientists of all time. But you and Newton would both have been wrong.
Einstein versus Newton
The common-sense formula - and the one Newton would have used - for the speed of the ball relative to the bystander is
Going back to our example of the light particle escaping from the front of a spaceship, this means that the speed of the particle of light looks the same to someone in the spaceship as to a stationary observer - the considerable speed of the rocket has made no contribution whatsoever!
It seems totally counterintuitive that the speed of light remains a constant, whatever the relative speed of the source and the observer - but it is backed by enormous amounts of evidence. But even more counterintuitive are the consequences for our understanding of time...
Slowing down time
To see the consequences for our understanding of time, we need to measure time using velocity, rather than the other way around, as is more usual. Our clock will consist of two mirrors and a beam of light. If the distance between the mirrors is d, then the time it takes for the light beam to travel from one mirror to the other is
Now let's put our clock on a fast-moving train moving at speed v. Alice has volunteered to sit in the train, and Bob has volunteered to observe the clock from outside the train.

Now where's that stopwatch?
To understand what Bob sees, imagine that the two mirrors have been placed so that one is vertically above the other, and so that a straight line through them forms a right angle with the floor of the train. Now for Alice, the light beam just moves up and down along this vertical line. But for Bob, because the train keeps moving, by the time the beam of light gets to the other mirror, both mirrors have moved! So instead of seeing the beam of light moving vertically up or down, he sees it travelling along a diagonal line:
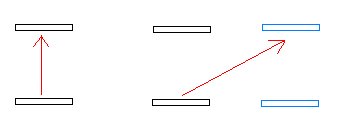
Alice's view is shown on the left. The beam travels vertically from one mirror to the other. Bob's view is shown on the right. The position of the mirrors before they have moved is indicated in black, and the position after they have moved in blue. The beam travels diagonally.
Poor old Bob decides he'll have to do his own calculation. Because the beam of light looks like it's moving diagonally, he decides that the distance the light has to travel isn't really d. He decides to call it D instead. Also, he decides that the time taken to travel between the mirrors isn't really t. He calls it T instead. But the speed of light is the same for both Bob and Alice (remember?), so Bob still calls it c.
Now because the train is moving at speed v, by the time the beam of light moves from one mirror to the other, the train has moved a total distance of vT. So, as far as Bob is concerned, the mirror has moved a distance of vT.
Bob realises that if you take the point at which the light beam started (one of the mirrors before it has moved), the point to which it would travel if the train was stationary (the second mirror before it has moved) and the point where, from Bob's point of view, the beam ends up (the second mirror after it has moved) together form a right-angled triangle:
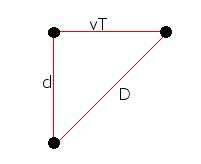
So what does this mean? Bob has seen Alice's clock, and he knows that it takes t seconds for the light to travel between the mirrors. But from his observation point outside the train, it looks to him as though it takes T seconds. Alice sees the clock working as expected, but because Bob is not with Alice on the train the clock on the train looks like it's running too slowly.
What Bob is seeing is a consequence of Special Relativity known as time dilation. To him, it appears that time on the train is running slowly. But now suppose that Alice gives him the clock, he sets it working by the side of the railway track, and she observes it from the train as she passes. All the same results hold - to Alice, it appears that the clock is going slow! In other words, both Alice and Bob observe time slowing down for the other!

How to measure time?
So what causes this madness? Are the laws of physics different for Alice in her moving train than they are for Bob in the stationary landscape outside? Surely not! Yet something happens as one looks across from his or her environment to the other. In the language of physics, the difference between Alice and Bob is that they are in different inertial frames of reference.
When we perform an experiment we usually take measurements using reference points like the top of a table or the start line of a race. The place where we do our experiment is called our frame of reference. A stationary laboratory is an example of what we call an inertial frame of reference, or inertial frame for short. It is inertial because if we choose a fixed point of reference - like the most distance stars - then the laboratory isn't accelerating with respect to this point. In fact, any frame of reference that is moving with constant velocity (ie not accelerating) with respect to an inertial frame is also an inertial frame - like Bob moving through space with the earth, with Alice moving past him at the constant speed of the train.
And the laws of physics - including the value c of the speed of light in a vacuum - are the same no matter which inertial frame you're in. So whether you're sitting on Earth, in a train or hurtling through space in a non-accelerating rocket, the laws of physics are the same in all places.
Everything's hunky-dory if we work inside one inertial frame. But all bets are off if you observe an experiment from one inertial frame when it is happening in another - as is the case for Alice and Bob, or when you look out of the window of a spinning rocket and see stars accelerating past you even though they are acted on by no forces.
All this might seem a little hard to believe, but by carrying out sophisticated experiments scientists have confirmed that this incredible thing does happen. Even Einstein found it hard to believe, because it goes against common sense.
The brief but eventful life of the Muon

Muons are created high in the Earth's atmosphere
Muons are particles created high up above the Earth's surface by cosmic rays hitting the atmosphere. They can be detected in large numbers at the Earth's surface. They travel at around 0.998 times the speed of light, but they don't live for very long - typically, they decay after only
So how come we are able to detect them at the Earth's surface? The answer is time dilation. In our frame of reference the lifetime of the muon is extended from
And in this time a muon can travel 9,500 metres - far enough to reach the surface of the Earth!
The twin paradox

Fly away Paul
This is the story of twins Peter and Paul. Paul makes a round trip in a high-speed spaceship and leaves his brother Peter on Earth. With the help of his telescope, Peter watches Paul whizzing through space. As a result of time dilation, Peter sees that Paul is aging more slowly than he.
But, just as Alice and Bob both thought that the other was experiencing time more slowly than themselves, shouldn't Paul observe Peter aging more slowly than he? This would mean that each twin thought the other one was aging more slowly.
In fact, the situation is not symmetrical: only the twin who stays at home is in an inertial frame. Paul experiences acceleration (that is, changes in velocity) as his rocket leaves Earth, turns around and comes back. In general, accelerated clocks (or clocks in gravitational fields) will go slow relative to unaccelerated clocks (or clocks feeling no gravitational fields). So the twin that feels the accelerations will age more slowly than the one who doesn't.
If Paul managed to maintain a constant velocity of 80% of the speed of light, by the time he returned home, he would be 6 years older, while Peter would have missed him for 10 years. Of course, it's unlikely that anyone could reach such a high speed, but it does make you wonder...
About the author

Comments
Anonymous
Confusing.
"Muons are particles created high up above the Earth's surface by cosmic rays hitting the atmosphere. They can be detected in large numbers at the Earth's surface. They travel at around 0.998 times the speed of light, but they don't live for very long - typically, they decay after only
"In our frame of reference the lifetime of the muon is extended from
Where did 0.000002 seconds come from/calculated or was measured in which frame.?
Marianne
The lifetime of muons (0.000002 second) can be measured in experiments, see for example http://www.particle.kth.se/~pearce/muonlab/muonlab.pdf It's because of the relativistic effects described in this article that time passes differently for the travelling muons than it does for us. Hope that answers the question!
Anonymous
It does not answer.
Did the entire lab travel at the same speed that the muon?
In which case it makes sense that they measure 0.000002 s. Otherwise they "measured" something else, strictly speeking.
Anonymous
Incredibly well explained! I've read many different author's explanation of how special relativity works (and also watched explanations in "Cosmos" and the movie "Insignificance". This is the first time I've felt even a little dint of understanding penetrate my brain. Thank You!
Aronas
Distressing to find non-SI/non-Metric units still being used in Science & Mathematics articles.
Sonjoy Premi
Excellent, easy to understand article !