
Measure for measure
Measure for Measure, or, How to make a carpet out of nothing.
"How long is a piece of string?" [1]
As long as you want it to be, of course! But when someone has asked that, either as a joke or to make a point in a conversation, did you ever stop to think that you were taking something very important for granted? Was it a question that worried you? Are you sure?
"It all depends on what you mean by..." [2]
When you are asked about the length of something - your height, the diameter of the earth, the size of an atom, the distance across our galaxy - you probably never doubt that an answer exists. It might be almost impossible for you to find, or you might not know what it is, but you are sure that an answer exists.
Aren't you?
In the real world you're (probably) right, but can you imagine objects that you can't measure? Not ones that don't exist, but real things that have no length or area or volume? Sounds weird, but they're out there. Before we meet some of them, we'll have a look at some of the strange objects you can produce just by applying a bit of (un)common sense to a line and a square.
"...and then he's gone - like that!" [3]
Start off with a line 1m long (Fig. 1) and remove the middle third, leaving the end points of the removed piece where they were - this gives you two lines, each
Figure 1
Figure 2
Next remove the middle third of each of these two lines (Fig. 3). That gives you four lines. Now remove the middle third of each of these and so on.
Imagine that you could keep on doing this indefinitely, repeating the process an infinite number of times. What would you end up with? It seems obvious that it will be a whole lot of points - the endpoints of each of the intervals; remember that we are not removing these endpoints when we take away the line segments. It might even be obvious to you that as we have repeated our procedure an infinite number of times, we will be left with an infinite number of dots. Nothing to worry about so far!
Now - how much of the line did you take away?
We can work this out by noticing that after the first piece has been removed we have two-thirds of the original length left. After the second stage we have two-thirds of that left. That means that we have
Now there might be a problem - we've removed the whole of the interval (remember that we started with a line of length one, and we've taken away lots of bits that all add up to the length one), but our common sense tells us that we should have an infinite number of points left over. How can it be that we seem to have taken the whole line away and yet there are an infinite number of points left? Don't worry - there's nothing wrong with your common sense, or even with your summing of an infinite series. What's wrong is that we're missing quite a subtle point; a point is not a little bit of a line. A point doesn't have some very small length; it has no length at all. No matter how many of them you put next to each other they will never take up any space, will never have any length. Remember that no matter how many zeros you add together, your answer will still be zero.

A Cantor Dust
(Notice that this is just the same method as that used to create a Cantor Dust in How big is the Milky Way? in Issue 15 of Plus except that we are using lines instead of squares. In fact, the set of points we have left in our example is called the Cantor Set. If you were wondering why the title of this article mentioned carpets, it's because when I first met the Cantor Dust, I heard it called Sierpinski's Carpet, and it's made out of nothing because if you add up the areas you have taken away, you find that you have removed all of the area with which you started.)
It's time to stop talking about length and get a bit more mathematically accurate. To avoid the confusion that we've seen is easy when thinking about this subject what we need is the mathematical idea of a measure.
No, not one of those metal things that you get out and unreel to check if your new curtains will fit.
In maths, a measure is a way of measuring how big something is, but it has a much more precise meaning than length or area or volume, and it can be applied in a much wider range of settings. Here (briefly) are some of the important facts about measures.
"Do you always play by the rules?"[4]
The rules for using a measure are pretty simple. You can make any way of recording a fact about something mathematical - its size, how many pieces it's in or (for a set) how many members it has - into a measure provided it follows some basic rules. Since most things in maths can be expressed in the language of sets (unions, intersections, empty sets etc.) it makes sense to use this language when we write down our rules.
- The measure of any set is a real number.
- The empty set has measure zero.
- If A and B are two sets with no elements in common (disjoint) then the measure of
I've simplified these rules a bit, but they should be enough to give you an idea of how a measure works. It should be even more useful to see what happens when we apply these rules to the Cantor Set and the sections of line which we removed to create it.
Call the Cantor Set, A, and call the set made up of all the sections of line that were removed, B.
To save us keep writing the phrase "the measure of" in every other line, we'll adopt a useful bit of notation and write
- For any set,
- If
(If you've ever done any probability, don't you think that rule 3 looks like the addition law for probabilities? This isn't a coincidence, but that's another story...)
Sets of zero measure are very useful in maths. We're going to look at one example of how they (and the idea of measure in general) let us extend calculus and allow us to integrate some very odd functions!
"It doesn't matter. Not really now not any more."[5]
Integration is (in its simplest form) a way of finding the area enclosed by a graph, the x-axis and two lines drawn up from the x-axis at x=a and x=b (a and b are called the limits of integration). Before we go on to see how measures can help us, it will be useful to see how the process of integration works out this area. What you do is to take small sections of the x-axis and draw rectangles up to the graph (Fig. 4). The sum of the areas of these rectangles then gives you an approximate value for the area under the graph, and if we let the rectangles become infinitely thin (whatever that means!), then we have the exact value - in other words we look at the limiting value as the width of the rectangles tends to zero.
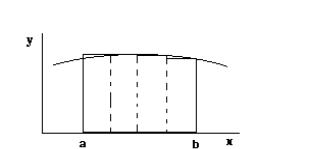
Figure 4
Now for the clever bit.
When we calculate the area of the rectangles, instead of using the length of a bit of the x-axis as the width, we can use the measure of the set made up of that bit of the axis. (For the more interested reader, what we are doing here is moving from Riemann integration to Lebesgue integration. These are two really big names in the world of pure maths - a university textbook or a good encyclopaedia or online search will tell you more. In fact, the type of measure we are using here is called Lebesgue Measure.)
Impressed? No?
Well, you should be. Using a measure means that anything that happens on a set of measure zero makes no difference to the value of the integral. That means we can integrate lots of functions that are not smooth curves, but jump about all over the place. An example should help.
Suppose that we want to integrate a simple function. We should have no trouble with the following:
Since the set of points where the function takes the value zero is just that - a set of isolated points - it has measure zero and so our theory tells us that we can ignore it, and integrating f will give us the same value as before. Similarly, we can make the function take any value we like on any set of measure zero and it will have no effect on the result of the integration. This means that we can ignore a lot of jumps in functions and integrate them as if they were nice, smooth, continuous functions.
It's worth noticing at this point that we need to be talking about a set of isolated points for the set to have measure zero. By 'isolated' I mean that each of the points must be separate from the rest. If the points were allowed to be 'touching' (and there were an infinite number of them!) then we'd get a section of a line, and that would have a measure greater than zero.
"Your mission, should you choose to accept it..."[6]
So everything's fine. We have our idea of a measure, and we've seen at least one circumstance where it's useful. Problem solved. Case closed.
Not quite.
If we were to leave it at that, it would be straightforward but dull!
Once the ideas of measures had been worked out, it occurred to someone to ask an awkward question: "Are all sets measurable?" What we mean by this question is, once we've defined what our measure is going to be, can we calculate the measure (which should be a number remember!) for every possible set? Again, I'm simplifying here, but we have captured the spirit of the question.
Fortunately (to give this article a point!) the answer is no, not all sets can be measured. Without going into too many details, what happens is that a set can be so complicated that it is impossible to measure it. Imagine a three-dimensional shape that is so jagged and crinkled that it is actually impossible to measure the volume of it, and you have a good idea of what is going on. (Naturally, the actual maths needed to make this concept precise is a bit tricky!)
However, the fact that sets that have no measure exist (we'll call them non- measurable sets, just to sound more mathematical!) mean that one of the most bizarre results in all of maths is true. Something so weird that many people in the last eighty years or so have called whole areas of pure maths rubbish just because they thought that this result couldn't possibly be right. This is the Banach-Tarski Paradox, and (translated from mathematical symbols into English) it says: It is possible to take a solid sphere, cut it up into pieces and reassemble them, without bending, stretching or distorting them, to give you two solid spheres, each of which has exactly the same volume as the original.
Go back and read that last paragraph again. If your common sense didn't bring you screeching to a halt in disbelief, you didn't understand it properly.
Think about what this means. Get yourself a lump of gold, volume
Obviously this can't happen in real life (or I'd have been out getting some old gold jewellery and a knife instead of typing this article), but there's nothing wrong with our theory. Let me explain.
What the Banach-Tarski Paradox tells you to do is to take your sphere (let's say it has a volume of 1, for convenience) and cut it up into pieces which are non-measurable. Now, because they are non-measurable, you've 'lost' the information of what volume you had to start with. This means that when you put them back together you can get any volume you want; 2, 3, 4, 97 etc. There is no volume in your pieces which has to be preserved when you reassemble them.
That's why the Paradox works, but it doesn't tell you why you can't do it in practice. The reason is that you can't actually physically create a non- measurable three-dimensional shape. It has to be infinitely complicated and so, although we can imagine and describe it, we can't actually make it.
"Your eyes can deceive you, Luke..."[7]
It might help at this point if we look at a simpler example where we take something apart, perform an operation on the bits and then put them back together to form two copies of the original. In fact, the example we are going to look at is the Banach-Tarski Paradox in action, it just doesn't seem as remarkable because we are dealing with objects that are much less familiar to us. As we go through this example, I'll point out how it corresponds to the impossible sounding version of the Paradox given above.
Suppose you have four objects,
Now split
Now put an extra
Now we glue the pieces together by finding the union of pairs of sets.
"...the end of the beginning."[8]
That's it.
We started off with a problem - not understanding the Cantor Set - and introduced the idea of a measure to help us get to grips with it. It then turned out that the measure is a powerful tool in a mathematician's armoury, letting us do all sorts of useful things like integrate some nasty functions. Finally we saw that when we carry our ideas to their logical conclusion, they give us some very strange results, (hopefully) opening up new areas for maths to move in and making the subject richer and its tools yet more applicable to real problems.
Hopefully at this point someone is asking "What real problems?" Are measures something apart from an intellectual challenge, a game for mathematicians? They certainly are. A quick search on the Internet for 'applications of Measure Theory' will produce references to probability (where probabilities can be calculated using something called the 'Probability Measure'), dynamical systems and ergodic theory (which have applications to imaging, number theory and communication theory), physics, chemistry and mathematical economics.
If reading this has whetted your appetite and you long to know more (or fill in the gaps where I've missed things out!), take a look at "The Banach-Tarski Paradox" by Stan Wagon and prepare to be amazed!
[1] Common saying.
[2] C. E. M. Joad, start of answer to questions on the BBC television programme Brains Trust.
[3] Roger 'Verbal' Kint (Kevin Spacey) in The Usual Suspects (1995).
[4] Mrs. Emma Peel (Uma Thurman) in The Avengers (1998).
[5] Closing line, Red Shift by Alan Garner.
[6] From Mission: Impossible, various TV and movie incarnations (1966-date)
[7] Obi-Wan Kenobi (Alec Guinness) in Star Wars (1977)
[8] From a speech by Winston Churchill
About the author

Andrew Davies graduated from Leeds University with a First in Mathematics in 1992. He then trained as a teacher, and has been working as a Maths (and occasional ICT) teacher since 1993. He took a career break to study more maths from 1996-9, and was a speaker at the NRICH-organised IMECT2 conference in July 2000, which he claims to have enjoyed, despite being terrified at the time! From September 2001 he will be working as Numeracy Coordinator at Whitehaven School in Cumbria.
Comments
Anonymous
Good argumets.. So, give me an example of what the "inverse" of an apples is..
Anonymous
what is the measure of adding the prefixes a-1 and b-1
it seems you are adding a prefix to an infinite set, which shifts that set, hence the doubling of the original measure comes from this non-free move (adding the prefix)
Andrew Davies
As they ARE infinite, the measure of them will not change - it remains countable infinite...
epsilon
Nice article, very well communicated.
Andrew Davies
Thank you!