50 Visions of Mathematics – fractal elephants
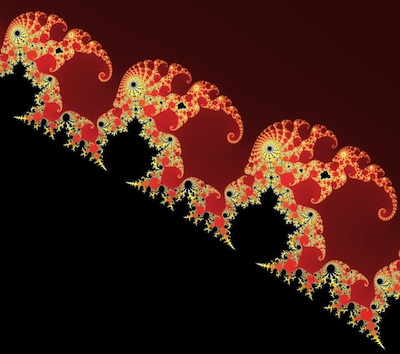
Image produced by Philip Dawid, using the program winCIG Chaos Image Generator developed by Thomas Hövel, © Darwin College, University of Cambridge, used with permission
This image appears to be a procession of elephants but is, in fact, a much-magnified small detail of one of the Mandelbrot set. The Mandelbrot set is a famous example of a fractal – mathematical objects whose structure is infinitely complex. Whether you're viewing them from afar or zooming in on them with a mathematical microscope, the same complexity is always visible. This self-similarity even extends to the some of the same structures repeating at all scales.
This unending complexity means that fractals live between dimensions. For example, there are shapes that are so crinkly, they are "more" than a one-dimensional curve, but not extensive enough to give a two-dimensional shape like a disc or square. Instead, they have a fractional, or fractal, dimension between 1 and 2, which is why Benoît Mandelbrot, the father of fractals, named them so.
Fractals famously appear in nature, from snowflakes to coastlines, and also have revolutionised mathematics by inspiring the field of chaos theory which is used in weather prediction and stock market analysis.
This beautiful image, created by Philip Dawid, is one of the images that appear in the book 50 visions of mathematics, that celebrates the 50th anniversary of the Institute of Mathematics and its Applications.
You can read more about fractals and an article by Dawid on Plus.
