
Drinking coffee in the Klein Café
This article is a runner-up of the schools category of the Plus new writers award 2006.

Dry and impenetrable?
The usual view of mathematics leaves a lot to be desired. If you don't know what I'm talking about then you're lucky you haven't got a jaded view of the subject. The picture on the right summarises the common view of maths: it's a dry subject and just belongs in a lecture-room full of equally dry adherents of this secretive and impenetrable art.
Not so! My favourite mathematical moments have been in pubs and cafés and it is not because I'm obsessed with food and hot chocolate (which I am). It is because maths is a very social subject and a subject that often requires a strong drink close to hand.

A strong drink of hot chocolate gets you doing maths.
However, you may have observed that checked shirts and pads of paper are always helpful!
In this article I'll explore what's been going on my mind for the last few days in the hope of giving a better view of the subject.

Shadow games
What strikes you about the picture on the right? That's right! The shadow.
As is clear, shadows may stretch and skew the shape of the object to which they belong. However, that wonderful pine cone has a beautiful shape that is completely annihilated by the shadow: this shadow is a vulgar view of the cone that expresses none of its beauty!
This is important to me because I want to know where all this detail goes: what would the shadow look like if it were more detailed? (And I hope you will be more enthused about this than my cat.) The question became a burning issue for me until finally I submitted and decided to do some experiments with my friends in what I'll call the Klein Café.
Shadows within shadows

Take this cake for example. If I put a light bulb in the place shown, the cake's shadow will be quite as you would expect. But the question is, how would the circle I have drawn on the cake (shown figure 1 below) appear in the shadow? Obviously you can't see the curve in the shadow but it would be fun to know where it ends up.
You can do this by eating the entire cake except for the blue loop; but it would just fall onto the plate. A better way is to poke through to see where the shadow would fall as I've done here in figure 1.

Figure 1. It takes a bit of prodding to see the shadow of this circle
What I've done is placed cocktail sticks into the cake where the loop is in line with the imaginary lamp. Then, joining the ends of the sticks into a loop on the napkin we get the original loop's shadow. As you can see the loop has simply stretched.
One might get the idea that this is all that can happen. But one example isn't enough to make such conjectures! So I had a go with an apple.

Here it is probably obvious that my shadows are only approximate. However, it is the "theme" of the change of shape that is important. The figure 8 is simply stretched unevenly; however, the circle has become a simple line which is not a loop at all.
It's interesting to try and find examples of changes in the fundamental structure of the shape (a stretch is not fundamental, but for a loop to become a line is, intuitively, a very significant change). Here are a few examples you could try with a donut:
Draw on a donut a figure 8 whose shadow is a circle ...
... and now try drawing two bits of straight line and get a P.
Given all these examples, the next question is natural: "Is every possible shape the shadow of another shape drawn on a certain surface?"
I now state the answer (but it is important to be sceptical, even if for no other reason than to have fun destroying donuts):
I emphasise (as it is the point of this article) that this wasn't a conclusion reached on a dusty blackboard and has consideration entirely free of Greek letters and even paper: it's what has come from sitting and chatting.
What next? Some rules!
This is all very nice but in maths you must always want to look for the next question and ascend to the next step of detail and interest — and you yourself have hopefully been enthralled by the entirely imaginary shadows within shadows because that's exactly what they are: interesting and very fun.
All games must be fun for people to want to play them, but to play seriously you need proper rules. So, as we want to play seriously, let us pin down a few things. Or to put it in another way: let's close a few windows to open a door. For example, if I am asked the question "do animals like to sleep", I cannot answer because animals are many and so are insomniacs; but if I am asked "do cats like to sleep", I can reply without a moment's hesitation. We are cutting down the variables so that we can say more about what is happening in these particular situations.
My rules are few. There are only two, in fact, but they help us immensely. Until now we have been looking at fruit of any shape at all. Now I will dictate that our fruit has just one highest point — with respect to the table it is sitting on — and that this highest point is not a sharp point (like the top of a cone), but quite flat (like the top of a sphere). I will also dictate that the bulb is placed atop this highest point of the object.

The Azimuthal map, image courtesy the US Geological Survey.
A commonly used example of this is the Azimuthal map of a hemisphere of the globe. This is produced with the set-up shown below on the right, where the shadows of countries appear on the outlined "sheet".

This is how you get the Azimuthal map. Earth courtesy NASA.
Of course, finding the diagram on the left using the set-up on the right would require lots of very long cocktail sticks but they seem to have managed it well.
Moving on, it's important for us to clear up what we are looking at: so far we have been looking at loops, curves or continents without actually properly defining how we view these things. A loop viewed at a certain angle becomes a line and a line becomes a curve; so we can't be sure of what we are talking about.
Let's say that a shape drawn on a fruit is a line if its shadow is a line (and by line we mean one that has unlimited length).

For example: the loop shown on this onion is a line because its shadow (if the light is placed on the highest point, which is marked) is a line. It is important that you make sure you see that this is so before moving on.
Once you understand this, you may see that with this definition of line, every line passes through the lamp. This is just one of many observations that are readily made with some experimentation: anything that does not pass through the lamp will have as its shadow something that is confined to some small area of the sheet underneath, and not something that is infinite in length, as we require from a line.
The disappearing point
I was not exaggerating when I described adding our rules as opening a door on much more mathematics. I can now show you an example of the beauty of the extremely simple set-up we have created. Have a look at this diagram:
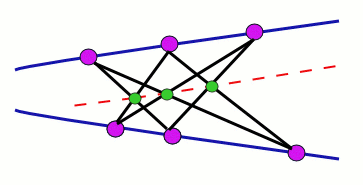
You may have seen this theorem before. It is called Pappus's theorem and it says that if you pick any two lines (shown in blue) and three points on each (shown in purple) and draw lines between them as shown, then the intersections of certain of these lines (shown in green) are in a line (that is dotted and red). This may seem like a mouthful and it is, but I advise you to simply look at the image: it is quite wonderful that this always works for any choice of blue lines and purple points.
I state it because this theorem also works on any fruit we choose to play with, with the definition of line that I gave above! But even more: let's go back to the ordinary plane and consider what happens if we start moving the top-left two purple points (now coloured yellow and red below) towards each other:
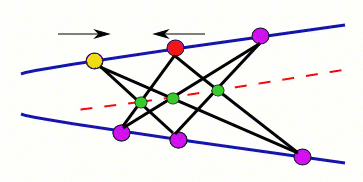
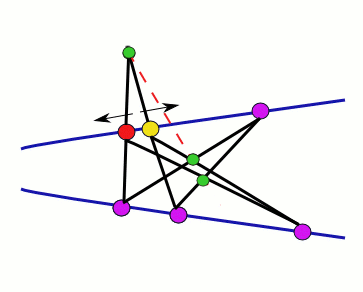
Keep on moving the red and yellow points (in the same respective directions) and you'll see that the green crossing point moves further and further out (with the theorem still working):
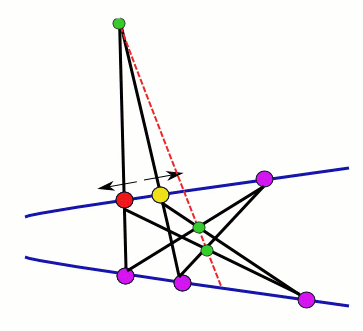
Until it disappears when the two lines that define it (which I've coloured orange for emphasis) are parallel:
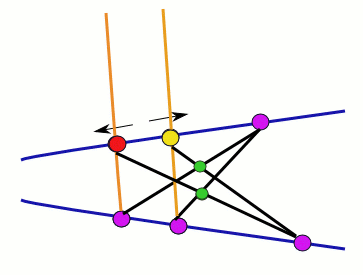
What happened to the theorem there?
But if we keep moving the two points even further away from each other, the green meeting point appears again — on the other side! The theorem also works again, as all green points lie on a line.
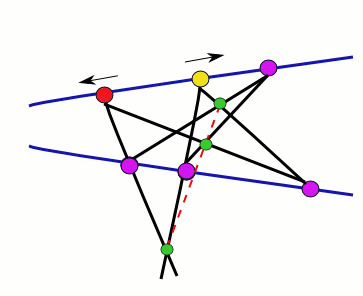
As far as the third green crossing point (and Pappus's theorem) is concerned, the simple process of moving the red and yellow points has caused a dramatic catastrophe: it has momentarily annihilated the point; erased it from existence, and then returned it in a completely different place. How can we understand this disaster?

It all becomes clear when we look at the same scenario on our fruit. Remember that we can consider our lines above to be shadows of lines on a banana, where the lamp is placed on the banana's highest point.
It turns out that on the banana, the green crossing point still exists even when the orange lines are parallel. If this seems doubtful, I have actually found the curves that make the shadows shown above (where the orange lines are parallel) on a banana. If you draw the lines on the fruit, then all three green points exist and there is a line that passes through them!
The set-up is shown in the photo above, but the picture is quite useless as it is far too confusing. With the sticks removed and the holes they have left used for guides I have highlighted the lines (coloured as above) so that we can see what is happening.
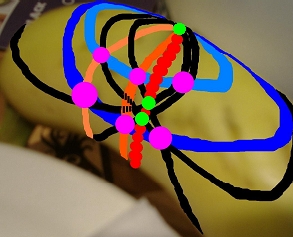
The orange lines here go right around the banana and many other lines are so large that they also loop right around the outside. However, every line meets at the highest point. Note that the dotted red line joins all three intersections, and the theorem works on our banana!
Bringing in infinity
The reason why the third green crossing point does not appear in the shadow of this set-up is that it sits at the highest point of the banana and, as you'll remember, this is also where our lamp is. Now pick some point (other than the lamp-point) on one of the orange lines (on the banana) and start moving towards the lamp-point. If you trace the shadow of your movement on the sheet, you'll find that you move further and further out — the lamp-point somehow corresponds to some point that is infinitely far away!
The catastrophe that occurs on the sheet as the orange lines become parallel is that the green crossing point has moved so far out that it is momentarily swallowed up by infinity. While this may seem dramatic on the plane, on the banana it simply means that the green point passes through the lamp-point. It then emerges on the other side of it, just like the green point on the sheet reappears on the other side of the blue lines — simple! And on the banana, Pappus's theorem continues to work all the while.
By looking at our set-up on the banana rather than the plane, we've turned the mysterious notion of infinity into an ordinary point. This trick is extremely effective and often used by mathematicians — though they usually don't use bananas.
Cutting cones
Now let's change the rules again. Remember, there are three elements to what we are doing: the lamp (with which we create shadows), the fruit (on which we draw curves), and the curve (with which we cast a shadow). Our rule specified the placement of the lamp and some characteristics of the fruit. Now let's allow the lamp's position to become arbitrary again and be more specific about the fruit and curve. Thence, we specify a new rule:
The "fruit" is always a perfectly flat surface (usually called a plane); and the curve is always a perfect circle.
These restrictions may seem extreme, but wait! I will elaborate what happens to illustrate that in mathematics whatever direction we travel in, we can find something interesting.
Supposing that there is a shadow at all (that the lamp isn't sitting somewhere "below" the circle), there are four possibilities:
- The shadow is a line. This happens if our lamp sits inside the plane that contains the circle, and you can figure out for yourself that, depending on the exact location of the lamp, the line may be finite or infinite.
- The shadow is a perfect circle, or an ellipse, a stretched circle, as illustrated below:
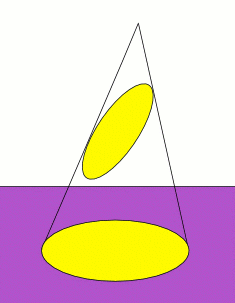
- The shadow is a shape called a parabola. It looks like a U-shape:
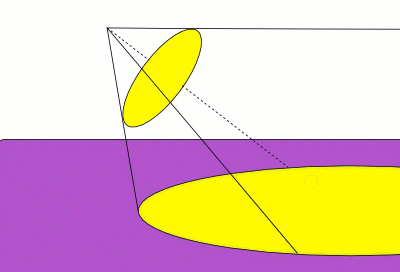
- The shadow is what is called a hyperbola:

These shapes, the parabola, the ellipse and the hyperbola, are probably familiar to you. They are called the conic sections because they are what you get from intersecting a cone, in this case the cone generated by the light rays that hit the circle, with a plane, in this case the floor, in three different ways. The ellipse arises when the intersection of the plane and the cone is finite, the parabola occurs when one of the lines that form the cone is exactly parallel to the floor, and the hyperbola happens in all other cases. The conic sections have been known to the ancient Greeks and are some of the most fundamental shapes in maths — this is where some simple shadow games can get you!
I hope that I have left the reader with questions that they find interesting and may now even share a thought for these things (and others) over coffee: be alert and look out for mathematics wherever it hides!

About the author

Jon's favourite maths areas at the moment are linear and affine spaces, but he also enjoys the little bit of projective that he has met — and this is mainly what his article is based around. At the moment he's taking stabs at topology but often he feels more like it is taking stabs at him!
He is a long-time member of Liverpool's Maths Club. Currently he is at Carmel Sixth-Form College.
He likes hiking and going to favourite pubs with friends, and then sleeping it off afterwards — the hiking, that is. Finally, he has two cats: the brown one is Sophie and the other is Dizzy (as in Gillespie).