
The Gömböc: The object that shouldn't exist
A Gömböc is a strange thing. It looks like an egg with sharp edges, and when you put it down it starts wriggling and rolling around with an apparent will of its own. Until quite recently, no-one knew whether Gömböcs even existed. Even now, Gábor Domokos, one of their discoverers, reckons that in some sense they barely exists at all. So what are Gömböcs and what makes them special?
Imagine an ordinary egg. You can put it down on its side, it will roll around a little but eventually come to rest. You can nudge it, but as long as you don't do it too hard it will settle down again. With a lot of skill you can also balance it on one of its two ends: it's not easy and if you manage, it won't last long. The slightest disturbance will make it topple over.
The two tips of the egg are known as unstable equilibrium points. "Equilibrium" because you can balance the egg on them and "unstable" because the egg will fall over at the slightest nudge. The points around the egg's body on which it balances robustly are called stable equilibrium points.
What combination of equilibrium points can an object have? It's easier to think in two dimensions. A square has four stable equilibrium points, the centres of its sides: if you trap the square between two vertical glass plates then you can balance it at those points. It also has four unstable equilibrium points at its corners. A triangle has three of each, again three stable ones at the centres of its sides and three unstable ones at its corners. An ellipse, which is a bit like a length-wise cross-section of an egg, has two stable equilibria in the centres of its long "side" and two unstable ones at its ends.
Can you find a two-dimensional shape that is convex (it doesn't bulge inwards) and homeogeneous (the material it's made of is uniform throughout without bulges or weights) that has just one stable and one unstable equilibrium point? The answer is "no". You can get some intuition as to whay that is by trying to get rid of one of the unstable equilibrium points of the ellipse. To do this, you'd have to flatten the end of the ellipse out in some way, creating another stable equilibrium in the process. There is a rigorous proof for this fact which shows that having one stable and one unstable equilibrium point would make impossible demands on a shape's centre of gravity.
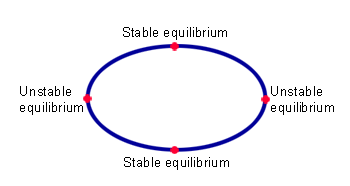
An ellipse has two stable and two unstable points of equilibrium.
Something similar seems to be true in three dimensions, so mathematicians naturally assumed that there isn't a three-dimensional convex and homogenuous shape with just one stable and one unstable point of equilibrium. Many tried to prove the result, but failed.
Others slowly came around to the idea that such an object might exist after all. These included the Hungarian Gábor Domokos who gave this object, should it exist, a name: he called it a Gömböc. Domokos tried hard to construct a Gömböc mathematically, to prove it does exist, but initially he failed just as miserably as Gömböc unbelievers failed to prove its non-existence. So he took what for a mathematician is a drastic measure. On a holiday to a Greek island he and his wife collected and inspected 2000 beach pebbles in the hope that one might behave like a Gömböc. It was a futile effort. "We learned very interesting things from a pebble point of view, but nothing from a Gömböc point of view," says Domokos. "We were tired and depressed. When you looked at these pebbles you got the feeling that even if you went to all other Greek islands, you would never find [one behaving like a Gömböc]. But why not? If this type of pebble doesn't exist, then there must be a mathematical reason for this."
This line of thought led to an important insight: Domokos and his colleague Péter Várkonyi realised that a Gömböc, if it existed, could not be very flat, or very thin. A flat object, like a frisbee, generally has two sides, and contained in each there'll be a stable equilibrium point — that's one stable equilibrium too many. A thin object, like a pencil, will generally have two unstable equilibrium points at its two tips, so it can't be a Gömböc either.
Domokos and Várkonyi made their intuition precise by giving a formal mathematical definition of what they meant by flatness and thinness, each measured by a number greater than or equal to 1. They then proved that a Gömböc's flatness and thinness both have to be equal to 1, that is, both values have to be as small as is possible.
The result gives some insight into why the Gömböc is only just teetering on the brink of existence. If you make a Gömböc just a tiny little bit thinner or flatter, then its flatness and thinness values aren't minimal anymore. So according to the result, the object stops being a Gömböc — it must have gained extra equilibrium points, or stopped being convex.
The Gömböc's sensitivity to change means that a Gömböc-like pebble can only exist for a short time on the sea shore before other pebbles, moved by the sea, start chipping away its Gömböc-ness and turn it into an ordinary pebble. "It's a fundamental question," says Domokos, "to which you expect a clear-cut answer: something should either exist or not exist. But in the case of the Gömböc, the answer is that it does exist, but barely so. If you drop it, it ceases to be what it was, so physically, it is a very fragile existence."
Having thus explained why they had not been able to find a Gömböc on the beach, Domokos and Várkonyi drew new hope and tried a new line of attack in their search for a proof of its existence. Eventually they were successful, pinpointing exactly what a Gömböc should look like. They even had a Gömböc made: when you put it down it performs a strange wriggeling dance, rolling around until it comes to rest at its single stable equilibrium point (see the video above). You can find out more on the Gömböc website. And if you find yourself close to Cambridge, you can come and admire a real Gömböc at the Whipple Museum of the History of Science, to which it was donated by Domokos and Várkonyi in 2009.
You can find out more about the maths behind the Gömböc in this article.
Comments
Anonymous
Very cool, but isn't this just Hungarian for a Weeble?
Marianne
A Weeble has a weight in it which causes it to self-right. In a Gömböc there's no weight, that's what the homogeneity condition means.
Anonymous
On Chrome I get the message "No plug-in available to display this content". Tried Firefox and can't view it there either.
plusadmin
I've updated it to show the mp4/webm versions.
Steve
Doesn't any 3 dimensional shape have an infinite number of unstable equilibrium points? How can there be only one?
Gabor Domokos
On the surface of a typical smooth 3D shape almost all points are NON-EQUILIBRIUM points. If the shape is supported on a horizontal plane at such a non-equilibrium point then it will roll off always in the same direction. Unstable equilibrium points are different: here the shape may roll off in any direction (depending on the initial push it gets from the experimenter). The Gömböc has exactly one such unstable equilibrium point (opposite to the single stable equilibrium where it is at rest).
LordPierpont
What would happen if you tried to suspend/levitate a metallic gömböc in a magnetic field? You can balance a sphere to levitate at an equilibrium point in a small magnetic field; would this be possible with a gömböc, and if so, what differences might be observed compared to the sphere? The sphere just sits there, or can be given spin when set, until air resistance slows and stops it. I'd imagine a gömböc either can't be placed at all, or would be in constant motion, possibly knocking itself off the equilibrium point at times.