Fibonacci (ca 1170 – ca 1250).
Dan Brown in his book, The Da Vinci Code, talks about the "divine proportion", an irrational number given the Greek symbol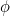 
, as having a "fundamental role in nature". Brown’s ideas are not completely without foundation. The proportion crops up in the mathematics used to describe the formation of natural structures like plants, and even in Alan Turing’s work on animal coats.
But Dan Brown does not talk about mathematics, he talks about a number,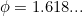 
. What is so special about this number?
From rabbits to ratios
The divine proportion appears in Fibonacci's 1202 book on financial mathematics, the Liber Abaci. The Liber is a series of increasingly difficult problems (with solutions) that generations of apprentice merchants used to learn their trade in the middle ages. It was the textbook that taught Copernicus, who wrote about money before he wrote about planets, and Simon Stevin (1548 – 1620), who made mathematics useful and helped free the Netherlands from Spain.
One of the simpler problems Fibonacci used was on the business of breeding rabbits. Given that one pair of rabbits produces one other pair of rabbits a month, how many rabbits will there be after a year, assuming that we start with one pair?
The model Fibonacci is using is that a pair of rabbits take a month to become mature. They then mate and the rabbit pregnancy lasts a month. So:
At the start (month zero): There is one pair of immature rabbits.
First month: The pair mature and mate.
Second month: A new pair is born to the original pair, so there are two pairs.
Third month: The original pair parent a new pair, the second pair reach maturity. In total there are three pairs.
Fourth month: The original and second pair have offspring, the third pair reach maturity. In all there are five pairs.
How many will there be in a year?
Carrying on this rule, Fibonacci calculated the number of rabbits at the start of each month as
| Month | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| Pairs | 1 | 1 | 2 | 3 | 5 | 8 | 13 | 21 | 34 | 55 | 89 | 144 | 233 |
This is the famous Fibonacci sequence. Each number in the sequence is calculated by adding together the preceding two numbers, for example the numbers after 233 would be 144 + 233 = 377 and then 233 + 377 = 610. What medieval merchants realised is that as you move along the sequence, the ratio of successive numbers approaches a number which had already been known to the ancient Greeks: the golden ratio.
The golden ratio first appears in proposition 30 of book VI of Euclid's Elements. Written around 300BC the Elements is the oldest maths text book and it is also the most influential one ever produced.
In book VI Euclid solves the problem of dividing a line up such that the ratio of the longer segment to the whole line is equal to the ratio of the smaller segment to the longer segment. For example, if we have a line of length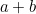 
where the length 
is bigger than the length 
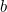, we want
![\[ \frac{a+b}{a}=\frac{a}{b}. \]](/MI/86d5e6f028fd7e594de5acb45bf0cb1f/images/img-0004.png) |
We use the symbol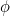 
to define this golden ratio.
It is quite easy to see that the golden ratio is the limit of successive ratios in Fibonacci's sequence (see here).
But Euclid’s golden ratio is also Brown’s divine proportion. To see this, remember we have defined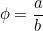 
and so 
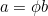. Now substitute this into the original expression
![\[ \frac{\phi b+b}{\phi b}=\frac{\phi b}{b}. \]](/MI/66e728c3e0f0d2efa7cd4af7326aac84/images/img-0003.png) |
Cancelling the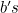 
gives
![\[ \frac{\phi + 1}{\phi }=\phi \]](/MI/66e728c3e0f0d2efa7cd4af7326aac84/images/img-0005.png) |
or
![\[ \phi ^2-\phi -1=0. \]](/MI/66e728c3e0f0d2efa7cd4af7326aac84/images/img-0006.png) |
The positive solution to this equation is
![\[ \phi =\frac{1+\sqrt{5}}{2}\approx 1.610339887... . \]](/MI/66e728c3e0f0d2efa7cd4af7326aac84/images/img-0007.png) |
Euclid would not have, could not have done this calculation, not least because the Greeks did not use decimals. Euclid would have tackled the problem in a different way. The equation
![\[ \phi ^2-\phi -1=0 \]](/MI/502fd17eb82a1aadf16879797337c37a/images/img-0001.png) |
can be re-arranged to give
![\[ \phi =1+\frac{1}{\phi }. \]](/MI/502fd17eb82a1aadf16879797337c37a/images/img-0002.png) |
Now, the denominator on the right is the same as the left hand side of the equality, so
![\[ \phi =1+\displaystyle \frac{1}{1+\displaystyle \frac{1}{\phi }} \]](/MI/502fd17eb82a1aadf16879797337c37a/images/img-0003.png) |
and again, and again, ...
![\[ \phi =1+\frac{1}{1+\displaystyle \frac{1}{1+\displaystyle \frac{1}{1+\displaystyle \frac{1}{\phi }}}}. \]](/MI/502fd17eb82a1aadf16879797337c37a/images/img-0004.png) |
Continuing in this way gives you an infinite expression involving only 1’s.
The man with the compass in Raphael's painting The school of Athens is believed to represent Euclid.
A number written in this way is called a continued fraction. The Greeks sometimes used continued fractions to write down non-integers. Euclid would have realised that 
was irrational (or incommensurable as he would have put it) because it never ended. What is more, it is a continued fraction made up only of 1’s.
This is why the Da Vinci Code is so ridiculous. If Robert Langdon, the book’s hero, was such an expert on symbols he would never present as a meaningless decimal, but as a continued fraction made up only of 1s. Why? Because 1 was highly symbolic to Christians as it represented the "one God". This is why the golden ratio is divine, because it is god’s number, not because there is a natural or super-natural significance of the ratio
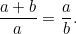
Finding the right perspective
There is an important aspect to this criticism of The Da Vinci Code. One of the significant features of mathematicians is their ability to look at things in different ways.
As an example consider any number, for example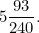 
There are many ways of writing it. Fibonacci would have written it as a composed fraction:
![\[ \frac{9 \; \; \; 7}{12 \; \; \; 20 }5. \]](/MI/e2692c459620be2bccd13640dd113a49/images/img-0002.png) |
This stands for
![\[ \frac{9}{12 \times 20}+\frac{7}{20}+5. \]](/MI/e2692c459620be2bccd13640dd113a49/images/img-0003.png) |
As a continued fraction it is:
![\[ 5+\frac{1}{2+ \displaystyle \frac{1}{1+ \displaystyle \frac{1}{1+ \displaystyle \frac{1}{2+ \displaystyle \frac{1}{1+ \displaystyle \frac{1}{1+\displaystyle \frac{1}{2 }}}}}}}. \]](/MI/e2692c459620be2bccd13640dd113a49/images/img-0004.png) |
In decimal notation, popularised by Stevin, it is
![\[ 5.3875. \]](/MI/7e5bfb7dc703758e265d71d12a201f09/images/img-0001.png) |
The craft of mathematics is essentially one of picking the best method in a particular circumstance. The history of mathematics is dominated by people who have come up with innovative solutions by changing the way problems are looked at. One of the most important examples is Newton’s approach to functions. Newton took his inspiration from decimal numbers. The digits to the right of the decimal point represent multiples of successive powers of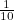 
. What we really mean when we write 5.3875 is
![\[ 5+3\left(\frac{1}{10}\right)+8\left(\frac{1}{10}\right)^2+7\left(\frac{1}{10}\right)^3+5\left(\frac{1}{10}\right)^4+0\left(\frac{1}{10}\right)^5+... \]](/MI/7e5bfb7dc703758e265d71d12a201f09/images/img-0003.png) |
Newton wondered whether a similar approach could be taken with mathematical functions of a variable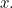 
Could they be written in terms of powers of 
? He realised that a fraction of the form
![\[ \frac{a^2}{b+x} \]](/MI/7e5bfb7dc703758e265d71d12a201f09/images/img-0006.png) |
could we written as
![\[ \frac{a^2}{b+x}=a^2\frac{1}{b}-a^2\frac{x}{b^2}+a^2\frac{x^2}{b^3}-a^2\frac{x^3}{b^4}+a^2\frac{x^4}{b^5}-... \]](/MI/7e5bfb7dc703758e265d71d12a201f09/images/img-0007.png) |
(You can work this out using long division in a similar way as you work out the decimal expansion from a fraction.)
Using an ancient technique for finding square roots, Newton also worked out that
![\[ \sqrt{1+x^2}=1+\frac{x^2}{2}-\frac{x^4}{8}+\frac{x^6}{16}-\frac{x^8}{128}+...$In general, Newton supposed that any function could be written as a \emph{power series},
\[ f(x)=a_0+a_1x+a_2x^2+a_3x^3+... \]
If the series was finite the function was analogous to a rational number, if it was infinite it was, in a sense, irrational. The branch of mathematics known as \emph{functional analysis} was born.
$ \]](/MI/7e5bfb7dc703758e265d71d12a201f09/images/img-0008.png) |
The golden ratio and art
This is a rhombicuboctahedron, by Leonardo da Vinci, from De divina proportione.
The golden ratio, or divine proportion, is most famous in an artistic context. This association with art comes from Leonardo da Vinci and the Franciscan friar, Lucca Pacioli, who produced a book, De divina proportione ("The divine proportion") together at the end of the fifteenth century. The book was written by Pacioli, the mathematician, and discusses the idea of perspective, the use of mathematics in architecture and the five perfect solids that were central in Greek thought. Leonardo, who had trained as a painter, illustrated the book and after studying mathematics with Pacioli, Leonardo went on to study engineering and anatomy, establishing a reputation today as something of a scientist. The collaboration between one of Europe's most famous artists and a some-time financial mathematician might seem odd, but in fact, it was through art Pacioli learnt his mathematics, which is odder.
Pacioli had been born in the small town of Borgo San Sepulcro, about 80 kilometres east of Florence, in 1446 and was taught the skills to become a merchant in an abaco school, based on the mathematics in the Liber Abaci. When he was around twenty he moved to Venice and worked for a wealthy merchant, certainly as tutor to his sons and possibly as his bookkeeper. In 1470 he moved to Rome and at some point in the next five years he became a Franciscan, which enabled him to work in universities. He started teaching mathematics privately, and then, between 1478–1480, at the University of Perugia. Between 1481 and 1489 he was again an itinerant maths teacher until he returned to San Sepulcro to write his most important book, the abaco textbook, Summa de arithmetica, geometria, proportioni et proportionalita ("Work on arithmetic, geometry and proportion"), which was published in Venice in 1494. In 1497 Pacioli was invited to Milan by its powerful ruler, Duke Lodovico Sforza, and it was Sforza's service that Pacioli met another of the Duke's employees, Leonardo.
Pacioli's home-town of Borgo San Sepulcro is more famous as the home of one of the Renaissance's most important painters, Piero della Francesca, famous for his use of perspective, such as in his painting The flagellation of Christ. Della Francesca had been born into a merchant family and between 1445 and 1460 was working on an altar piece for the church of San Sepulcro, Madonna della Misericordia. It is inconceivable that the young Pacioli did not know della Francesca, but scholars also believe the artist, who in 1470 published Trattato d'Abaco ("Abaco treatise"), had taught the Pacioli financial mathematics. De divina proportione, a collaboration between a financial mathematician and an artist, is sometimes seen as just an Italian translation of Piero's work Libellus de Quinque Corporibus Regularibus ("Short Book on the Five Regular Solids"). Today, we associate the divine proportion with Leonardo, but it probably came to him from Pacioli, who in turn learnt it from the financial mathematician-cum-artist, Piero della Francesca. Truth is sometimes more amazing than fiction.
Further reading
- The books The algorithmic beauty of sea shells and The algorithmic beauty of plants explore the formation of natural structures.
- Turing's paper on animal patterns is called The chemical basis of morphogenesis.
About the author
Tim Johnson was the RCUK Academic Fellow in Financial Mathematics between 2006-2011, during the credit crisis, and is active in promoting the sensible use of mathematics in finance, highlighting the need for more research into mathematics in order to better understand random and complex environments. He is based at Heriot-Watt University and the Maxwell Institute for Mathematical Sciences in Edinburgh. He teaches modern financial mathematics and undertakes research in stochastic optimal control and the rich history of the relationship between finance and science. Prior to becoming an academic, he worked for sixteen years in the oil exploration industry.
You can read more about probability and financial mathematics on Tim's blog Magic, maths and money.