
The Mandelbrot set. Image Wolfgang Beyer.
We know that there are shapes in the plane whose outline is incredibly crinkly. Examples are fractals, like the famous Mandelbrot set. But just how complex can a shape be?
One concept that captures some of a shape’s crinkliness is local connectivity, or rather a lack of it. To understand local connectivity, first think of a comparatively simple shape, like the one in the picture on the left (call it  ). Pick a point
). Pick a point  that’s part of
that’s part of  — for illustration we’ll pick one that lies on its outline. Now draw a little disc
— for illustration we’ll pick one that lies on its outline. Now draw a little disc 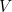 with
with  at its centre and look at the intersection of
at its centre and look at the intersection of  and
and 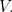

As you can see in the picture, it may happen that the intersection consists of more than one connected component. In our example this happens because the outline of 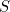 curves around and re-enters the disc
curves around and re-enters the disc 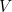 again at a place away from
again at a place away from 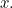 However, that’s only because we chose
However, that’s only because we chose 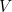 to be quite large. By making
to be quite large. By making 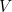 smaller we can ensure that the intersection of
smaller we can ensure that the intersection of 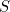 and
and 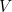 consists of only one connected component. A shape
consists of only one connected component. A shape 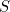 (drawn in the plane) is locally connected at
(drawn in the plane) is locally connected at  if the intersection of a disc
if the intersection of a disc 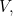 centred at
centred at 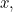 and
and  consists of a single connected component for all discs whose radii are small enough.
consists of a single connected component for all discs whose radii are small enough.
A shape  is locally connected if it’s locally connected at all its points. All the obvious shapes you can think of — circles, squares, triangles — are locally connected. (The formal definition for a general topological space
is locally connected if it’s locally connected at all its points. All the obvious shapes you can think of — circles, squares, triangles — are locally connected. (The formal definition for a general topological space  is that
is that  is locally connected if for all points
is locally connected if for all points  in
in 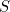 and open neighbourhoods
and open neighbourhoods 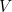 of
of  there is an open connected neighbourhood
there is an open connected neighbourhood 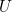 of
of  that’s contained in
that’s contained in 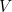 .)
.)
How can a shape not be locally connected? An example is the comb space. Think of the closed interval ![$[0,1]$](/MI/80f57dd79e30e5a8eab65f6074d1f741/images/img-0001.png) (closed means it contains its endpoints). Now at every point of the form
(closed means it contains its endpoints). Now at every point of the form 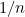 where
where 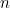 is a natural number, erect a vertical spike of length 1. Also include such a spike at the left-most point of the interval
is a natural number, erect a vertical spike of length 1. Also include such a spike at the left-most point of the interval ![$[0,1],$](/MI/80f57dd79e30e5a8eab65f6074d1f741/images/img-0004.png) that is, at the point
that is, at the point  So we have vertical spikes at
So we have vertical spikes at 
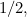
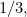 and so on.
and so on.
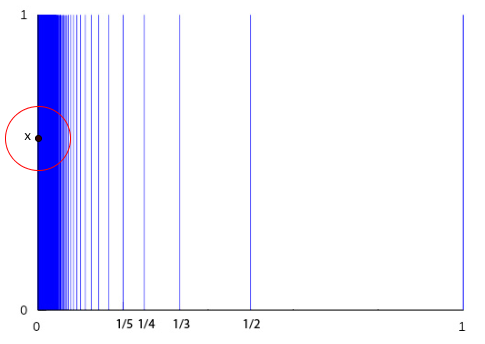
Now pick a point  somewhere on the left-most vertical spike (but not at the point where that spike meets the horizontal interval) and look at any disc
somewhere on the left-most vertical spike (but not at the point where that spike meets the horizontal interval) and look at any disc  small enough to not contain any piece of the horizontal interval. Since there are infinitely many points of the form
small enough to not contain any piece of the horizontal interval. Since there are infinitely many points of the form 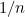 arbitrarily close to
arbitrarily close to  on the horizontal interval, the disc
on the horizontal interval, the disc  will contain a piece of each of these infinitely many separated vertical spikes. That’s true no matter how small
will contain a piece of each of these infinitely many separated vertical spikes. That’s true no matter how small  is, so the comb space is not locally connected at any point
is, so the comb space is not locally connected at any point  on the left-most vertical spike.
on the left-most vertical spike.
So is our favourite fractal, the Mandelbrot set, locally connected? The answer is that we don't know. Mathematicians believe that it is, and they have been able to show that it is locally connected at many of its points – but they haven't been able to prove that it's locally connected at all of them.
Comments
Fascinating
Very interesting read, especially considering I'm struggling with a somewhat related problem: contour offsetting (contour and not polygon because it can include arcs). Eliminating a section from between its neighbours as it shrinks away to nothing would be doable, but the problem is you never know when some other, non-local section of your contour starts interfering with the section you're trying to offset and each-with-each gets rather daunting, fast. For a poor code monkey like me it can all be a bit overwhelming... :)
Connectivity and Ratios of Rates of Change
The question can be stated as to which rate of change is the faster one. Is the rate of shrinkage of the contour or of the continuation loop (circle) the greatest.
If they are related, then the connectivity is zero because the RATE of shrinkage of the fractal (and of the comb too) depend inversely on the size of the previous change which is a logarithmic variation, whilst the rate of change of the continuation loop is a reciprocal one. Then connectivity is zero for both cases.
However if these two changes are arbitrary or unrelated, there is no answer.