The lines of intersection and metallic numbers
We are looking at an infinite family of circles. The first circle is centred on the point 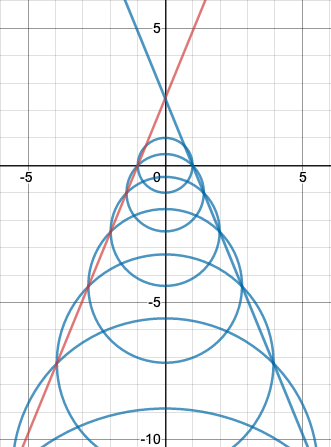 We want to show that all the intersection points that lie on the same side of the
We want to show that all the intersection points that lie on the same side of the 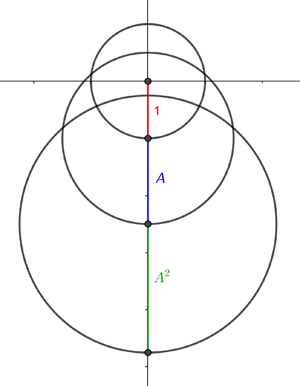 Our proof rests on the fact that there is quite a simple transformation of the plane which sends each circle in the family to the next one along. This transformation, call it
Our proof rests on the fact that there is quite a simple transformation of the plane which sends each circle in the family to the next one along. This transformation, call it 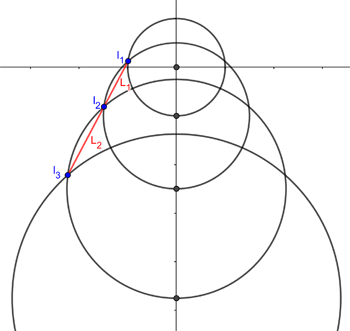 By point (2) above,
By point (2) above,

The family of circles with the two lines of intersection. The y-intercept is the silver ratio.

The centres and radii of the first three circles.
We leave the following two facts for you to prove:
- The transformation
- The transformation

Points of intersection and the line segments connecting them.
Back to the main article.