At this year's Black Heroes of Mathematics conference, Maurine Atieno Songa, a PhD student at the University of KwaZulu-Natal, South Africa, gave a brilliant introduction to category theory.
Songa explained that you can use category theory when you are trying to understand the interconnection of various systems. A category is a collection of objects, together with a morphism (often represented as an arrow) between every pair of objects.
As is the way in mathematics, these morphisms have to adhere to some rules. There must be an identity morphism that takes each object to itself, for example the morphism 
in the category illustrated below. And you can compose morphisms, so if there are two morphisms, 
that takes 
to 
, and 
that takes to 
, then there must be a third morphism 
that takes to .
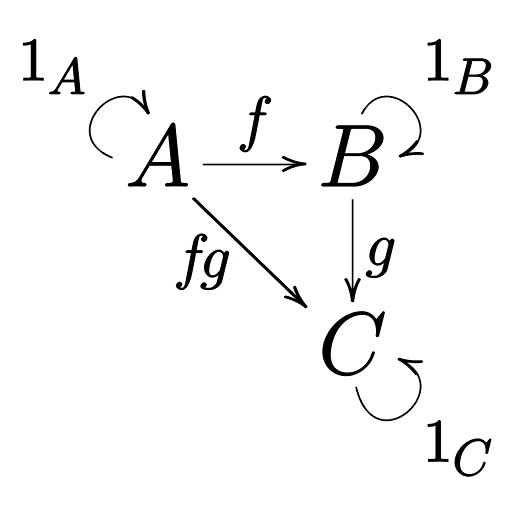
(Diagram based on one by kamusumeFan – CC BY-SA 4.0)
The identity morphism and composition of morphisms must obey the unit law axiom, which essentially says that the identity morphism behaves as you'd expect when composed with other morphisms (that if 
then 
). Composition of morphisms also needs to be associative: it doesn't matter where you put in your brackets when composing several morphisms (that is, 
).
To understand such an abstract concept, we were greatly helped by Songa's simple and more concrete example of a category whose objects are three sets: the set of all DNA sequences, the set of all RNA sequences and the set of all proteins. These form a category with the morphisms describing how these objects interact (in addition to the identity morphisms that are left out of the diagram below). Category theory can provide a formal and abstract language for formulating and tackling problems in such a complex area of science (you can see an example of how category theory can be used in this way in this paper).
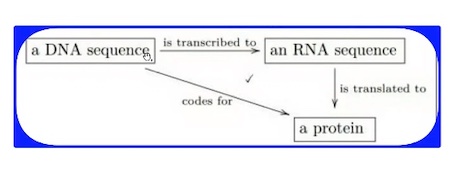
A slide from Songa's talk, with an example of a category drawn from genetics.
Category theory provides a way to describe the interconnection of objects, and that includes how categories themselves relate to each other. You can relate one category to another using something called a functor. In this way you can see which categories, and which functors, are equivalent to each other: they are the same in some important sense that tells you so much more information than the traditional notion of equality we are used to.
Categories in cosmology
The very abstract nature of categories has proved incredibly useful within mathematics. Indeed, all mathematical objects can be described in the language of category theory (similar to the way that set theory can be thought of as the foundation of mathematics). But what was most surprising in Songa's talk was the application of category theory in so many places outside of mathematics, including neuroscience, chemistry, electrical circuits and computer science.
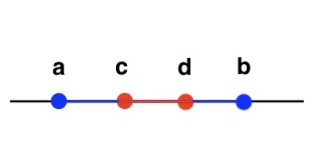
An example of an interval domain is the collection of all closed intervals on the real line, ordered by reversed set inclusion. So for the closed intervals shown in this image [a,b] comes before [c,d] in this interval domain, as [c,d] is contained within [a,b].
In particular, Songa explained the application to causal-set cosmology, the area she is researching for her PhD. General relativity explains gravity in terms of the geometry of spacetime. Causal sets are a special type of partially ordered set (or poset) which can be used to describe spacetime – for some events you can explicitly say which happened first, but there are some space-like separated events where it's impossible to say which happened before the other (you can see this in action in Violating causality).
There is a known equivalence between the category of these special types of posets, and the category of something called interval domains (see diagram on the right). "The implication is that we can construct spacetime purely in this order theoretic manner," says Songa. And the question she is thinking about for her PhD is: What are the properties of these special interval domains, and what does that mean for gravity and the description of spacetime?
Thank you very much Maurine Antieno Songa for this introduction to category theory and the amazing places it can take you!
Return to the Plus advent calendar 2021.
About this article
This article is based on Maurine Atieno Songa's lecture at the Black Heroes of Mathematics conference in October 2021.
Songa is a PhD candidate at the department of Mathematics, University of KwaZulu-Natal. Her work focuses on some applications of category theory to real life situations, applying theories of interconnected systems to that of spacetime, chemical reaction networks and machines. Songa has also a published two books that aim to inspire younger women and girls to take up STEM and to know that they too, can rise above difficult circumstances in life.
This article was produced as part of our collaboration with the Isaac Newton Institute for Mathematical Sciences (INI) – you can find all the content from the collaboration here.
The INI is an international research centre and our neighbour here on the University of Cambridge's maths campus. It attracts leading mathematical scientists from all over the world, and is open to all. Visit www.newton.ac.uk to find out more.
