A Reader's Solution

Share this page
Submitted by plusadmin on 1 September, 1999
September 1999

The first correct solution we received for Puzzle No 8 - The Gobbling Goat was this one from V. Shashidhar.

You can see the more detailed PASS Maths solution for a step-by-step explanation of how to tackle the problem.

The answer to the question is 115.8 metres.

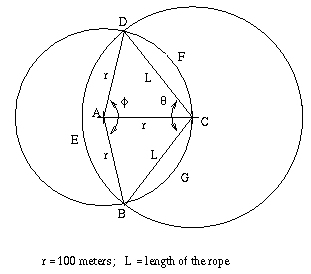
Solution diagram 1

It comes as a root to the equation

  \begin{equation} {2r^2 \arcsin (\frac{L}{2r})} - {\frac{L}{2}\sqrt{4 r^2 - L^2}} + {L^2 \arccos (\frac{L}{2r})} = {\frac{\pi r^2}{2}} \end{equation}   (1)

where $r=100$ metres and $L$ is the length of the rope used to tie the goat.

The derivation is as follows (see figure):

  $\displaystyle \theta $ $\displaystyle = $ $\displaystyle 2 \arccos \frac{L}{2 r} $    
  $\displaystyle \phi $ $\displaystyle = $ $\displaystyle 4 \arcsin \frac{L}{2 r} $    
  $\displaystyle Area(CDEB) $ $\displaystyle = $ $\displaystyle \frac{\theta L^2}{2} $    
  $\displaystyle Area(DCF) $ $\displaystyle = $ $\displaystyle Area(ADFC) - Area(ADC) $    
  $\displaystyle $ $\displaystyle = $ $\displaystyle {r^2 \arcsin \frac{L}{2 r}} - {\frac{L}{4} \sqrt{4 r^2 - L^2}} $    
  $\displaystyle Area(EDFCGB) $ $\displaystyle = $ $\displaystyle Area(CDEB) + 2 Area(DCF) $    
  $\displaystyle $ $\displaystyle = $ $\displaystyle \frac{\pi r^2}{2} $    

This implies equation (1).