Like sand through the hour glass, so is the maths of our lives.

There are more grains of sand on Earth than there are stars in sky, or so the saying goes.

Mathematician Anne Fey, from Vrije Universiteit Amsterdam, is using sand-pile models as a novel approach to calculate probabilities in fields as diverse as studies of the Earth's crust, stock market fluctuations and the formation of traffic jams (you can read more about other models of traffic jams in the Plus article Unjamming traffic).

Sand-pile models are fairly easy to picture, but the results are difficult to predict because of the fascinating self-organisation that evolves. Imagine you slowly drop sand onto grid paper through your fingers. Each point on the grid has a height of sand that must not be exceeded — if it is, the sand tumbles onto adjacent points. With each time interval, the amount of sand on the grid paper increases as does the height at each point. If this height exceeds a maximum value, the sand flows onto neighbouring points and perhaps further still if that flow caused the amount of sand on those neighbouring points to exceed their maximum. The flow continues until the amount of sand at each point is below this critical value.

The rules of Fey's model are very simple, but despite this, self-organising complex behaviour can emerge. Stable patterns can evolve and some of these exhibit the complexity of fractals. This occurs, for example, when sand is poured onto the mid-point of the pattern. Sand then spreads out around this point in highly angulated forms. If enough sand is poured into the middle, symmetrical fractals about the mid-point form.

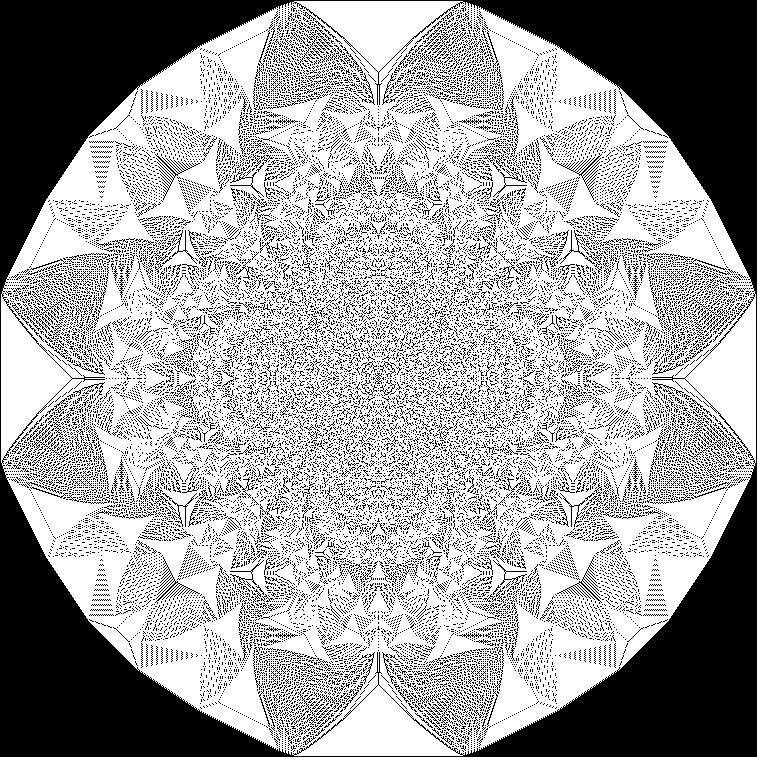
Fractal patterns can emerge from simple rules. Image courtesy Vrije Universiteit Amsterdam.

Situations that can be used to study movements in the Earth's crust and stock market movements occur when the choice of the point where the sand is initially poured is not at the centre of the grid but randomly chosen. This is when the most unpredictable behaviour emerges. Similarly complex chain reactions can ensue in stock markets, for example when one stock is considered overvalued by the market and falls down to a price the market considers more realistic, with a knock-on effect on other prices.

Mathematically, the model starts with an initial configuration $\eta$ that consists of a number of sand grains at each grid point. Each unstable site is then toppled such that each point that contains at least $2d$ grains gives one grain to each of its neighbours $-$ $2d$ being the critical height. This process may yield a final configuration in which each point is stable $-$ in that case, $\eta$ is $stabilisable$. Fey proved that stabilisable configurations, within some conditions, depend only on $\eta$ and not on the particular order in which the toppling is conducted $-$ that is, which neighbour gets the first grain of sand. $ This is an interesting conclusion: a stabilisable pattern in a complex process like the stock marked would mean that no matter how and where a chain reaction kicks off, we can be sure that it will settle eventually — something of importance to all investors!