
Symmetry rules
Everybody will recognise the inkblot in figure 1 as being symmetric, but few know that figure 2 is also considered symmetric in the precise mathematical sense. So, what is symmetry, really? And why has this concept become so pivotal that many scientists believe it to be the source from which the laws of nature originate?
 Figure 1: This inkblot is obviously symmetric... |
 Figure 2: But so is this! |
When things that could have changed, don't
Symmetry represents immunity to possible changes — those stubborn cores of shapes, phrases, laws, or mathematical expressions that remain unchanged under certain transformations. Consider for instance the phrase "Madam, I'm Adam". This phrase is symmetric with respect to back-to-front reading, letter by letter. That is, the sentence remains the same when read backwards. The title of a known NOVA documentary: A Man, a Plan, a Canal, Panama, has the same property. Phrases with this type of symmetry are known as palindromes, and palindromes play an important role in the structure of the male-defining Y chromosome. Until 2003, genome biologists believed that due to the fact that the Y lacks a partner (with which it could swap genes), the genetic cargo of this chromosome was about to dwindle away through damaging mutations. To their surprise, however, the researchers that completed the Y's genome sequencing discovered that these chromosomes fight destruction with palindromes. About 6 million (out of 50 million) of the Y's DNA letters form palindromic sequences. These "mirror" copies both provide backups in the case of bad mutations, and allow the chromosome, in some sense, to have sex with itself — strands can swap position.
For figures and shapes drawn on a flat piece of paper, there are precisely four types of "rigid" symmetries (when stretching and distortions are not allowed), known as: reflection, rotation, translation, and glide reflection.

Figure 3: A butterfly's bilateral symmetry
We encounter symmetry under reflection all around us — this is the familiar bilateral symmetry that characterises animals and numerous artifacts. Draw a line down the middle of a picture of a butterfly (figure 3). Now flip it over, while keeping the central line in place. The resulting perfect overlapping indicates that the butterfly remains unchanged under reflection about its central line. Many letters of the alphabet also have this property. If you hold a sheet of paper with the phrase
to a mirror, it looks the same.

Figure 4: A snow flake is symmetric under rotation
Symmetry under rotations is also very prevalent in nature. A snowflake (figure 4) rotated through 60, 120, 180, 240, 300, or 360 degrees about an axis through its centre (perpendicular to its plane) leads to an indistinguishable configuration. A circle rotated through any angle about a central (perpendicular) axis will remain unaltered.
Symmetry under translation is the type of immunity to change encountered in recurring, repeating motifs, such as the one in figure 2. Translation means a displacement or shift, by a certain distance, along a certain line. Many classical friezes, wallpaper designs, rows of windows in high-rise apartment buildings, and even centipedes, exhibit this type of symmetry.
Finally, the footprints generated by a left-right-left-right walk are symmetric under glide reflection (figure 5). The transformation in this case consists of a translation (or glide), followed by a reflection in a line parallel to the direction of the displacement (the dotted line in figure 5).
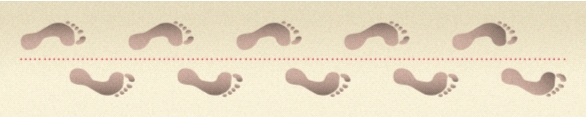
Figure 5: Footprints are preserved by glide reflection
All of the symmetries discussed so far are symmetries of shapes and forms — ones that we can actually see with our own eyes. The symmetries underlying the fundamental laws of nature are in some sense close kin to these, but instead of focusing on form or figure they address a different question: What transformations can be performed on the world around us that would leave the laws that describe all the observed phenomena unchanged?
Symmetry rules
The "laws of nature" is the name given collectively to a body of rules (usually expressed mathematically) that are supposed to literally explain (in principle at least) everything we observe in the universe. That such a grand set of "rules of the game" even exists was inconceivable before the seventeenth century. Only through the works of scientific giants such as Galileo Galilei (1564-1642), René Descartes (1596-1650), and in particular Isaac Newton (1642-1727), did it become clear that a mere handful of laws could explain a wide range of phenomena. Suddenly, things as diverse as the falling of apples, tides on the beaches, and the motion of the planets, all fell under the umbrella of Newton's law of gravitation.
Similarly, building on the impressive experimental results of Michael Faraday (1791-1867), the Scottish physicist James Clerk Maxwell (1831-1879) was able to explain all the classical electric, magnetic, and light phenomena with just four equations! Think about this for a moment — the entire world of electromagnetism in four equations. Incidentally, Faraday's revolutionary experiments in electromagnetism provided the backdrop for an amusing anecdote. A British statesman watching an experiment from which he could discern no practical result asked Faraday: "Of what use is such a discovery?" To which Faraday retorted: "Honestly I don't know, but I'm sure you will soon be able to collect taxes from it."
The laws of nature were found to obey some of the same symmetries we have already encountered, as well as a few other, more esoteric, ones. To begin with, the laws are symmetric under translations. The manifestation of this property is simple: whether you perform an experiment in New York, in Los Angeles, at the other edge of the Milky Way galaxy, or in a galaxy a billion light-years from here, you will be able to describe the results using the same laws. How do we know this to be true? Because observations of galaxies all across the universe show that not only is the law of gravity there the same as here, but also that hydrogen atoms at the edge of the observable universe obey precisely the same laws of electromagnetism and quantum mechanics that they obey here on Earth.
The laws of nature are also symmetric with respect to rotations — the laws look precisely the same whether we measure directions with respect to north, the nearest Starbucks, or the party line — physics has no preferred direction in space. Nature has been kind to us in being governed by truly universal laws, rather than by parochial bylaws. Unlike in the real estate business — where everything is location, location, location — neither our location in space nor our orientation with respect to distant stars makes any difference for the laws of nature we deduce.
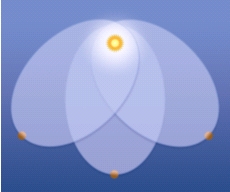
Figure 6: Newton's law of gravity may be symmetric under rotations, but this doesn't mean the orbits are.
A word of caution is needed to distinguish between symmetries of shapes and symmetries of laws. The ancient Greeks thought that the orbits of the planets around the Sun have to be symmetric with respect to rotation. This required that the orbits would be circular. In fact, it is not the shape of the orbit, but Newton's law of gravity that is symmetrical under rotations. This means that the orbits can be (and indeed are!) elliptical, but that the orbits can have any orientation in space (figure 6).
In the opening paragraph of this article I made a stronger statement than merely saying that the laws obey certain symmetries; I said that symmetry may be the source of laws. What does this mean?
The source of natural laws
Imagine that you have never heard of snowflakes before, and someone asks you to guess the shape of a snowflake. Clearly, this is an impossible task. For all you know, the snowflake may look like a teapot, like the letter S, or like Bugs Bunny.
Figure 7: Trying to reconstruct a snowflake
In the same way, the requirement that the laws of nature would be symmetric under certain transformations not only dictates the form of these laws, but also, in some cases, necessitates the existence of forces or of yet undiscovered elementary particles. Let me explain, using two interesting examples.
One of Einstein's main goals in his General Relativity was to formulate a theory in which the laws of nature would look precisely the same to all observers. That is, the laws had to be symmetric under any change in our point of view in space and time (in the physics lingo, this is known as "general covariance"). An observer sitting on the back of a giant turtle should deduce the same laws as an observer on a merry-go-round or in an accelerating rocket. Indeed, if the laws are to be "universal", why should they depend on whether the observer is accelerating or not?
While Einstein's symmetry requirement was certainly reasonable, it was by no means trivial. After all, a million whiplash injuries per year in the United States alone demonstrate that we do feel accelerations. Every time an aeroplane hits an air pocket, we feel our stomachs physically leap into our throats — there appears to be an unmistakable distinction between uniform and accelerating motion. So how can the laws of nature be the same for accelerating observers, when these observers appear to experience additional forces?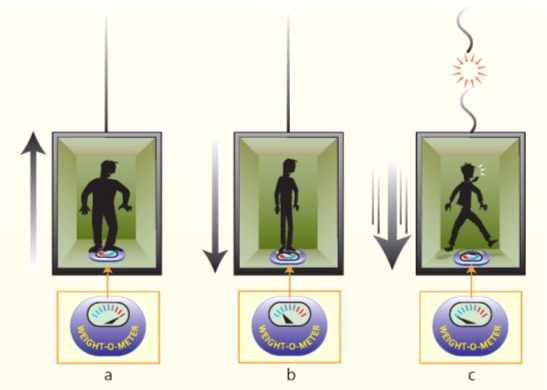
Figure 8: Weight control, elevator style - gaining weight when the elevator accelerates up (a), losing weight when it accelerates down (b) and achieving weightlessness when it free falls (c).
Consider the following situation. When you stand on a bathroom scale inside an elevator that is accelerating upward, your feet exert a greater pressure on the scale — the scale will register a higher weight (figure 8a). The same would happen, however, if somehow gravity became stronger in a static elevator. An elevator accelerating downward would feel just like a weaker gravity (figure 8b; artistic license was taken in the figure — the person would not look thinner). In fact, if the elevator's cable snaps, you and the scale would be free-falling in unison, and the scale would register zero weight (figure 8c). (This is not a recommended weight-loss procedure!) Free-fall is therefore equivalent to someone miraculously switching gravity off. This led Einstein in 1907 to a ground breaking conclusion: the force of gravity and the force resulting from acceleration are in fact one and the same. This powerful unification has been dubbed the "equivalence principle", implying that acceleration and gravity are really two facets of the same force — they are equivalent.
In a lecture delivered in Kyoto in 1922, Einstein described that moment of epiphany he had in 1907: "I was sitting in the patent office in Bern when all of a sudden a thought occurred to me: If a person falls freely, he won't feel his own weight. I was startled. This simple thought made a deep impression on me. It impelled me toward a theory of gravitation."
The equivalence principle was really a statement of a pervasive symmetry; the laws of nature — as expressed by Einstein's equations of general relativity — are the same in all systems, including accelerating ones. So why are there apparent differences between what is observed on a merry-go-round and in a laboratory at rest? General relativity provides a surprising answer. Those are differences only in the environment, not in the laws themselves. Similarly, the directions of up and down only appear to be different on Earth because of the Earth's gravity. The laws of nature themselves have no preferred direction (they are symmetric under rotation); they do not distinguish between up and down. Observers on a merry-go-round, according to general relativity, feel the centrifugal force that is equivalent to gravity. The conclusion is truly electrifying: The symmetry of the laws under any change in the spacetime coordinates necessitates the existence of gravity! This is the meaning of symmetry being the source of forces — the requirement of symmetry leaves nature no choice — gravity must exist.
Quantum symmetry
Some symmetries dictate the existence of previously undetected particles. The symmetries I have discussed so far had all to do with a change in our viewpoint in space and time. Many of the symmetries underlying the basic forces of nature and the elementary (subatomic) particles are of a different type — we change our perspective on the identity of particles. Within the fuzziness allowed in the quantum realm, even the familiar electron can be in states in which it is "mixed" with another elementary particle called a neutrino. The neutrino is a particle of almost zero mass and no electric charge. Just as the Moon can be full, dark, and anything in between, particles can carry the label "electron", "neutrino", or be a mixture of both, until we perform a measurement that can distinguish the two.
The realisation of this ability of particles to metamorphose between different states took physicists an important step towards the unification of all the forces in nature — a theory in which different forces represent different sides of the same coin. In particular, in the late 1960s, physicists Steven Weinberg, Abdus Salam, and Sheldon Glashow showed that the electromagnetic force and the weak nuclear force are nothing but different aspects of the same force.
The electromagnetic force acts on particles that have an electrical charge, and is responsible for many phenomena we experience in everyday life, including electrostatic forces and magnetic forces. The weak nuclear force is about a hundred thousand times weaker within the nucleus than the electromagnetic force and is responsible, for example, for the radioactive decay of uranium, and plays an important role in the energy-producing reactions in the core of our Sun. The force transforms a neutron into a proton, while creating in the process an electron and an antineutrino (the "antiparticle" of a neutrino). The theory of the unification of these two forces (the unified force was subsequently dubbed the electroweak force) was one of symmetry's most dramatic success stories.
The electromagnetic force affects electrons, but not neutrinos because neutrinos have no electrical charge. But since a particle can move continuously from being an electron to being an neutrino, something corresponding to the electromagnetic force should act on neutrinos, too. A neutrino only feels the weak nuclear force and gravity, so (ruling out gravity due to its very weak effect on neutrinos) the scientists deduced that the weak nuclear force is simply the electromagnetic force in another guise.
The electromagnetic force is produced by the exchange of messenger particles — bundles of energy called photons (figure 9) — between electrically charged particles.
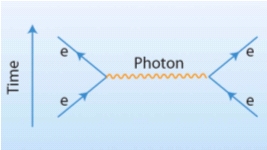 Figure 9: The messenger particles for the electromagnetic force are photons. |
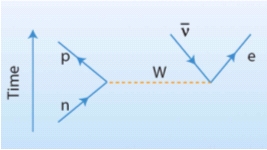 Figure 10: Symmetry dictated the existence of equivalent messenger particles for the weak nuclear force. |
By analogy, similar messenger particles should exist for the weak nuclear force, too. The electroweak theory predicted the existence of these never-before-seen particles called W and Z (figure 10 shows the equivalent of figure 9, for the weak force transforming a neutron into a proton). Symmetry's triumph was complete when these particles were discovered experimentally in 1983 and 1984, and they were found to have precisely the masses predicted by the theory (they are about ninety times more massive than the proton).
The symmetry between the two forces is perfect — the laws of nature take the same form if electrons are interchanged with neutrinos or with any mixture of the two. The same is true if photons are interchanged with the W and Z force-messengers. Just as the symmetry of the laws of nature under any change in the spacetime coordinates requires the existence of gravity, the symmetry between electrons and neutrinos dictates the existence of the photon and the W and Z messenger particles. Once again, it appears that when one starts with the symmetry, the laws practically write themselves. So, is symmetry truly fundamental in the universe, or is the human brain somehow tuned to only latch onto the symmetric aspects of the universe?
How fundamental is symmetry?
Imagine what would have happened if the human eye were sensitive only to a very narrow range of wavelengths, say, in the red part of the electromagnetic spectrum. Early scientists would have naturally concentrated on explaining why everything in the observable cosmos was red. Similarly, a fisherman using a net with holes that are 10 inches by 10 inches in size might conclude that all fish are longer than 10 inches. These are very simple examples of what are known as observational selection effects — filterings of the physical reality introduced by unrecognised biases either in the observational tools or the methods of observation. Could it be that our symmetry-based theories reflect a similar bias?

Figure 11: Does this look right to you?

Figure 12: A disconcerting experience for even the harshest critic.
Symmetry also plays a key role in the selection of mates (both for animals and humans). Biologist Randy Thornhill, psychologist Steve Gangestad and their colleagues measured symmetry on different facial and body features in about a thousand students. When they correlated these data with independent ratings for attractiveness, they found that less symmetrical people (in either face or body) were considered less attractive. More surprisingly, in a study with 86 heterosexual couples, Thornhill and Gangestad discovered that women whose partners were most symmetrical experienced a significantly higher frequency of orgasms.
One of the suggested interpretations for the attractiveness of symmetry is that symmetry is seen as a fitness indicator. The degree of symmetry of one's face, according to this hypothesis, indicates how well the genome of this person is suited to the environment. Independent research by psychologists Todd Shackelford and Randy Larsen has shown that symmetry in the human face does indeed correlate well with other fitness indicators, on both the physiological and psychological sides.
Okay, so the human mind is attracted to symmetry, and is finely tuned to detecting it. Does this mean that symmetry is perhaps not truly fundamental to the universe itself, but merely to the universe as perceived by humans? This is not an easy question. My personal conclusion is that symmetry principles always tell us something important. They often provide the most valuable clues toward deciphering the underlying principles of the cosmos, whatever those may be. In this sense, therefore, symmetry is certainly fruitful. Whether or not some all-encompassing symmetry is the grand principle that will necessitate our "theory-of-everything" is still to be determined.
About the author

Mario Livio's book, The equation that couldn't be solved: how mathematical genius discovered the language of symmetry, is reviewed in this issue of Plus.
Dr. Mario Livio is a Senior Astrophysicist at the Space Telescope Science Institute (STScI), the institute which conducts the scientific program of the Hubble Space Telescope. He received his Ph.D. in theoretical astrophysics from Tel Aviv University in Israel, was a professor in the Physics Dept. of the Technion-Israel Institute of technology from 1981 till 1991, and joined STScI in 1991. Dr Livio has published over 400 scientific papers and received numerous awards for research and for excellence in teaching. His previous book, "The Golden Ratio," won him the "Peano Prize" and the "International Pythagoras Prize" in 2004.
His interests span a broad range of topics in astrophysics, from cosmology to the emergence of intelligent life. Dr. Livio has done much fundamental work on the topic of accretion of mass onto black holes, neutron stars, and white dwarfs, as well as on the formation of black holes and the possibility to extract energy from them. During the past few years, Dr. Livio's research focused on supernova explosions and their use in cosmology to determine the nature of the "dark energy" that pushes the universe to accelerate, and on extrasolar planets.
Comments
Anonymous
I'm an art student, this is really helpfull. Thanks a lot.
Anonymous
thank you
Anonymous
Very educational. Thank You
Anonymous
thank you for your clear concept
Anonymous
Had been hearing a lot about symmetry, transformations, invariance, snow flakes, groups and finally this article describes everything so well. Nothing extra just to the point.