
Maths in a minute: Euclid's axioms

Euclid of Alexandria
Euclid of Alexandria was a Greek mathematician who lived over 2000 years ago, and is often called the father of geometry. Euclid's book The Elements is one of the most successful books ever — some say that only the bible went through more editions. It was also the earliest known systematic discussion of geometry. In it Euclid laid down the rules of geometry.
Euclid was interested in all the things you could do with a straight edge (a ruler with no marks) and a compass. He came up with his own set of five rules that described some basic things you could do with these tools, as well as some facts about angles and lines he thought were obviously true and didn't need to be explained.
- Given any two points, you can draw a straight line between them (making what's called a line segment).
- Any line segment can be made as long as you like (that is, extended indefinitely).
- Given a point and a line segment starting at the point, you can draw a circle centred on the given point with the given line segment as its radius.
- All right angles are equal to each other. (This may sound a bit strange, but you can find out more here.)
- If you draw a line segment across two straight lines and it creates two angles on the same side which add to less than two right angles, then those two straight lines intersect. (This axiom is equivalent to saying that the angles in a triangle add up to 180 degrees.)
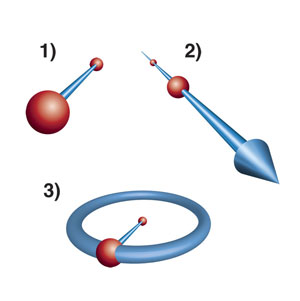
The first three axioms.
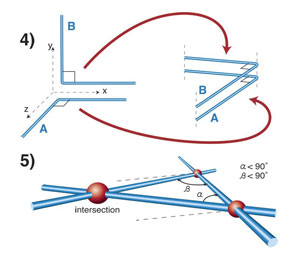
Axioms 4 and 5.
There are lots of nice things you can do with Euclid's axioms. For example, you can draw a regular hexagon:
Animation by Aldoaldoz.
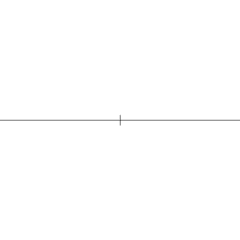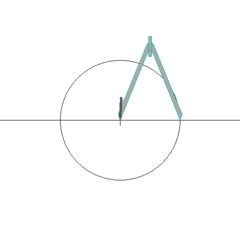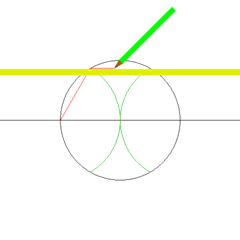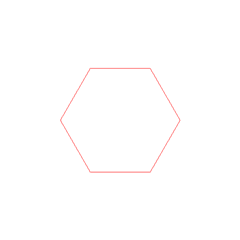
Or you can bisect an angle, that is, you can divide it into two equal angles:
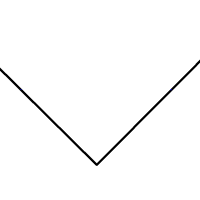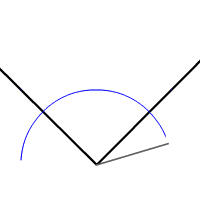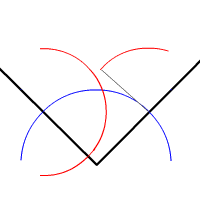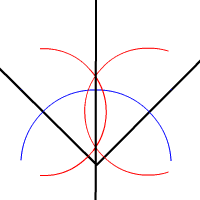
Indeed, these are the tools and methods that stone masons used to create their beautiful decorations and windows in churches and cathedrals. (You can read more here.)
The fifth axiom, however, caused mathematicians some problems: they though that it should actually be a consequence of the first four facts. Their attempt to prove it eventually guided them into the weird and wonderful world of non-Euclidean geometry. Find out more here.
Comments
Anonymous
The link
http://www.cut-the-knot.org/triangle/pythpar/PTimpliesPP.shtml
shows how the Pythagorean Theorem is equivalent to the Parallel Postulate. In my mind the parallel postulate was always linked to ideas of similarity, scaling and multiplication ( constructing similar triangles is effectively multiplying one line segment by another ). Does the equivalence to the Pythagorean theorem mean that the fifth dictates how area is to be defined in an Euclidean Geometry? Or that how area is defined determines the geometry?
Anonymous
It appears that the notion of measurement of area is slipped by Euclid through the back door. I think he needs more axioms such as: The area measure of a figure equals the sum of any subdivision into essentially disjoint figures. This "evident truth" does not follow from his postulates. [By the way, he should have done the same for lengths of lines]. Secondly, the area of a square is postulated to be equal to the square of its side. I believe that with these two additions, the problem is resolved. If you look at the usual proofs of Pythagoras theorem, they can be split into those that use the above postulates (addition of disjoint areas) and those that don't. To the second kind belong proofs that simply use similarity of triangles. But even here there is a problem, namely, the use of algebra to open brackets in a product. The way Euclid would justify opening brackets in a product is nothing but another way to assume that areas are additive. This technique was used by Moslem mathematicians, for example, to solve the quadratic equation by "completing the square". Either way, there is no escape from the conclusion that Euclid needs more axioms, namely, those pertaining to measure. I wonder why Hilbert did not point this out. I may be missing something.
M. E.