
Outer space: Some benefits of irrationality
There is more to photocopying than meets the eye. If you do it here in Europe then you will soon appreciate a feature so nice that you will have taken it for granted. Put two sheets of A4 paper side by side face-down on the copier and you will be able to reduce them so that they print out, side by side, on a single piece of A4 paper. The fit of the reduced copy to the paper is exact and there are no awkward extra margins on the page in final copy. Try to do that in the United States with two pieces of standard US Letter-sized paper and you will get a very different outcome. So what is going on here, and what has it got to do with mathematics and irrationality?
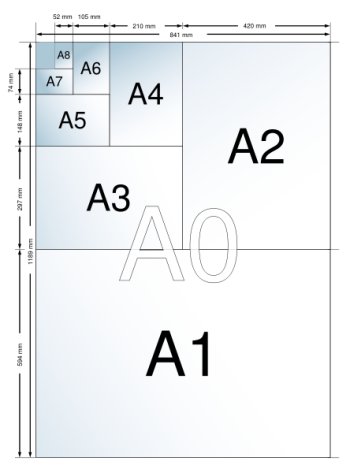
The AN paper series. Image from Wikipedia, reproduced under the GNU Free Documentation License.
The International Standard paper sizes, of which A4 is one, derive from a simple observation first made by the German physicist, Georg Lichtenberg, in 1786. Each paper size in the so called A-series has half the area of the next biggest sheet because it is half as wide but just as long. So putting two sheets side by side creates a sheet of the next size up: for example, two A4 sheets make one
A3 sheet. If the length is L and the width is W, this means that they must be chosen so that
This requires L2 = 2 W2 and the sides are in the proportion
This irrational ratio between the length and the width of every paper size, called the "aspect ratio" of the paper, is the defining feature of the A paper series. The largest sheet, called A0, is defined to have an area of 1 square metre, so it dimensions are L(A0) = 21/4 m and W(A0) = 2-1/4 m, respectively. The aspect ratio means that a sheet of A1 has
length 2-1/4 and width 2-3/4, so its area is just 1/2 square metre. Continuing this pattern, you might like to check that the dimensions of a piece of AN paper, where N = 0,1,2,3,4,5,... etc, will be
The area of a single sheet of AN paper will therefore be equal to the width times the length, which is 2-N square metres.
Problem: You might like to investigate the dimensions of the B series of paper sizes which also have an aspect ratio of √2. The area of a sheet of BN is the geometric mean of the areas of the AN and AN-1 sheets.
All sorts of aspect ratios other than √2 could have been chosen. If you were that way inclined, you might have gone for the Golden Ratio, so beloved by artists and architects in ancient times. This choice would correspond to picking paper sizes with
but it would not be a wise choice. The reason why, and the beauty of the √2 aspect ratio becomes most obvious if we return to the photocopier. It is this specific aspect ratio that ensures that you can reduce one side of A3, or two sheets of A4 side by side, down to a single sheet of A4 without leaving a gap on the final printed page. You will notice that the control panel on your copier offers you a 70% (or 71% if it is a more pedantic make) reduction of A3 to A4. The reason is that 0.71 is approximately equal to 1/√2 and is just right for reducing A3 or 2×A4 sheets to one of A4. Two dimensions, L and W, are reduced to L/√2 and W/√2, so that the area LW is reduced to LW/2, as is required if we want to reduce a sheet down to the paper size below.
Likewise, for enlargements, the number that appears on the control panel is 140% (or 141% on some) because √2 = 1.41 approximately. Another consequence of this constant aspect ratio for all reductions and magnifications is that diagrams retain the same relative shapes: squares do not become rectangles and circles do not become ellipses when their sizes are changed between A series papers
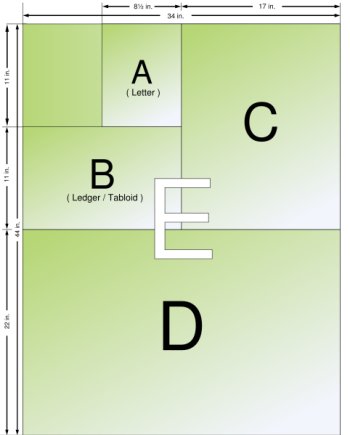
The ANSI paper sizes. Image from Wikipedia, reproduced under the GNU Free Documentation License.
Things are different in America and Canada. The American National Standards Institute (ANSI) paper sizes in use there, in inches because that is how they were defined, are A or Letter (8.5 in × 11.0 in), B or Legal (11 in × 17 in), C or Executive (17 in × 22 in), D or Ledger (22 in × 34 in) and then E Ledger (34 in × 44 in). They have two different aspect ratios: 17/11 and 22/17. So, if you want to keep the same aspect ratio when merging paper sizes you need to jump two paper sizes rather than one. As a result, you cannot reduce or magnify two sheets of one size down to one sheet of the size below or above without leaving some empty margin on the copy. When you want to make reduced or enlarged copies on a US photocopier you have to change the paper trays around in order to accommodate papers with two aspect ratios rather than using the one √2 variety as we do in the rest of the world. Sometimes a little bit of irrationality helps.
Did you manage to answer the puzzle posed in Outer space: Bridging that gap? If not, you can find the answer here!