
Secret symmetry and the Higgs boson (Part II)
In the first part of this article we explored Landau's theory of phase transitions in materials such as magnets. We now go on to see how this theory formed the basis of the Higgs mechanism, which postulates the existence of the mysterious Higgs boson and explains how the particles that make up our Universe came to have mass.
The electroweak theory

Dedication of a mural painted by Josef Kristofoletti directly above the cavern of the ATLAS Experiment at CERN, which searches for the Higgs boson. (Copyright CERN Geneva.)
Over the last 400 years physicists have steadily revealed the connections between the many and varied forces that control the motion of the bodies around us and give structure to the matter from which our Universe is formed. By the middle of the 20th century all known phenomena could be accounted for in terms of just four forces: gravity and electromagnetism, plus two forces that play important roles in nuclear physics — the weak force and the strong force.
The strong force holds subatomic particles together to form protons and neutrons and binds these protons and neutrons together to form the nuclei of atoms. The weak force is also extremely important, as it is able to transform protons into neutrons and neutrons into protons and thereby transmute the elements. The weak force is vital for the synthesis of all the atoms heavier than hydrogen within very massive stars. These atoms are dispersed into space in the supernovae explosions that end the lives of these supergiant stars. They may then be incorporated into the material that forms later generations of star systems such as our own.
Electromagnetism and the weak force have completely different characteristics. The electromagnetic force holds atoms together with negatively charged electrons bound to a positively charged nucleus. But electromagnetism also operates over very long distances. For instance, the Earth's magnetic field will attract the tip of a compass needle wherever the compass is located on Earth. By contrast, the weak force is an extremely feeble force that only operates over a very short distance — approximately one thousandth of the diameter of a proton.
In the 1950s attempts were made to construct models that united the electromagnetic and weak forces within a single theory. These elegant models were built around symmetries between fundamental particles. (When physicists talk about symmetry they mean a transformation of an object that leaves the object unchanged. For instance, if a snowflake is rotated by 60 degrees around its centre, its appearance remains the same.) Symmetry was a critical component of the electroweak models and this implied that they were rigid structures that could not be adjusted arbitrarily to fit the facts. For the models to work, the symmetry had to be exact.
But there was a problem. Particle physics tells us that the fundamental forces operate by the exchange of force carrier particles known as gauge bosons. For instance, the electromagnetic force acts by the exchange of massless units of light called photons. The weak force could be described in the same way, and its weakness would be explained if it were mediated by gauge bosons that, in stark contrast to photons, do have mass. Unfortunately, the inclusion of these massive particles seemed to completely destroy the symmetry embedded in these theories, thereby rendering them inconsistent and useless.
The physicist Peter Higgs recognised that what was required was a symmetry breaking mechanism that hid some of the symmetry of the original theories, whilst retaining all the nice features that symmetry brought to these theories. Higgs realised that Landau's model (explored in the previous article) would fit the bill nicely.
In 1964 Higgs translated Landau's ideas into the language of particle physics and constructed a model that demonstrated how, in principle, the symmetry encapsulated in theories of forces such as electromagnetism or the weak force could be spontaneously broken.
This would be the basis for a unified theory of the electromagnetic and weak forces. They could now be considered as two manifestations of a single electroweak force, thus reducing the number of independent forces from four to three. This represented the greatest unification of the forces since the unification of the electric and magnetic forces by Michael Faraday and James Clerk Maxwell in the 19th century. Higgs did not attempt to construct such a theory himself, but he came up with the theoretical mechanism behind it. Steven Weinberg took the next step and applied Higgs' idea to an electroweak model that had earlier been studied by Sheldon Glashow. Abdus Salam worked independently on a similar model and the theory is known as the Glashow-Weinberg-Salam (GWS) model.
Ripples in a quantum jelly
Peter Higgs. Notice the Mexican hat diagram on the left of the blackboard. (Copyright Peter Tuffy, The University of Edinburgh)
So how does the model constructed by Peter Higgs work? We sometimes think of fundamental particles as though they were tiny billiard balls moving around and bouncing off each other. But in the quantum field theories that describe the world at subatomic distances, this picture does not apply. Such theories associate each species of fundamental particle with a quantum field which permeates the whole of space. At each point in space the field fluctuates like a spring or simple harmonic oscillator. What we interpret as particles are excitations in the field.
You can think of this field as a sort of quantum jelly that extends through space. Where there is no particle the jelly just wobbles in a regular fashion. A particle corresponds to a ripple in the jelly, a disturbance of the regular wobble, at a particular point. So a particle travelling along corresponds to a ripple travelling through the jelly, and two particles interacting correspond to two ripples meeting.
Mathematically such a quantum field is represented by something called a scalar field: a number associated to every point in space. The number captures the effect the field would have on a particle interacting with it at that point.
Higgs constructed a model in which a quantum field
The direction of time is upwards in the diagram. At the bottom of the diagram two particles approach each other, at the centre they interact with strength β, then at the top they recede from each other again.
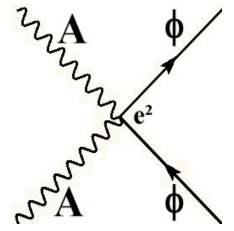
Time runs upwards in this diagram. It represents a process in which an A particle and a Φ particle approach each other, interact with strength e2 and then recede from each other again.
A massive phase transition
Higgs proposed that immediately after the Big Bang the Universe went through a dramatic phase transition. At extremely high temperatures in the very early Universe, the parameter
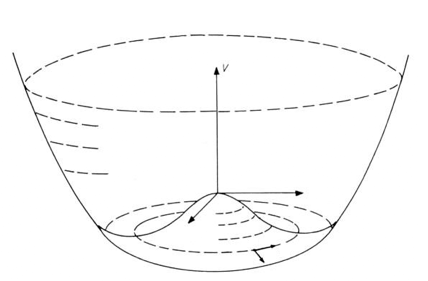
Mexican hat diagram
Below the critical temperature the lowest energy configurations of the
Acquiring mass
The quantum fieldThe state we are in today

The tunnel of the Large Hadron Collider during construction. The sealed ends of the two beam pipes can be seen side-by-side projecting from the end of the pipeline. ( Copyright CERN Geneva.)
But is the theory true? Does it represent the way that the Universe really works? Many of the predictions of the electroweak theory have now been verified in particle accelerators. The most spectacular was the discovery of the massive W-plus, W-minus and Z-nought particles at CERN in 1983. The only prediction that remains to be confirmed is the existence of the Higgs particle itself. Recent hints from the Large Hadron Collider suggest that an announcement of its discovery is just a matter of time. (see Hooray for Higgs).
About the author

Nicholas Mee studied particle physics and mathematics at the University of Cambridge. He was Senior Wrangler and went on to complete a PhD there with the title Supersymmetric Quantum Mechanics and Geometry. He is the founder of the educational software company Virtual Image.
Mee is the author of the book Higgs force: The symmetry-breaking force that makes the world an interesting place, which has been reviewed in Plus.
Comments
Anonymous
You write:
The only difference is that is expressed as a complex number so φ^2 is written as φ* φ.
This looks like the "*" is an ordinary multiplication. But it means the complex conjugation a+bi->a-bi.
So the star has to be a superscript instead.
Mark81
This is the first explanation I've seen of the Higgs field that is accessible enough to read without prior knowledge of gauge theories and all the rest, while still having enough detail to explain some of how it works. Good read!