
Abel Prize 2015: all wrapped up
John Nash and Louis Nirenberg, winners of the 2015 Abel Prize, will sympathise with anyone trying to wrap them a celebratory gift. They have been recognised for their contributions to solving mathematical versions of this problem – fitting flat space onto mathematical surfaces.
Unless you have a nice, flat-sided object to wrap, it will take some fiddling to make a flat sheet of paper wrap around an object neatly. For example, if you've every had to wrap a ball, the paper will start off sitting smoothly over a small area but will require more and more folds and gathers as you wrap more and more of the ball.
This difficulty might seem blindingly obvious – the surface of the ball is curved, the piece of paper is flat. But Nash and Nirenberg made many breakthroughs in such isometric embeddings – essentially neatly wrapping surfaces (and manifolds – their higher dimensional equivalents) in Euclidean space (which generalises the geometry of a flat piece of paper to any number of dimensions). (If you'd prefer a more technical definition, an isometric embedding is a distance preserving continuous injection from one metric space to another.)
A manifold is a mathematical object that viewed up close looks like ordinary Euclidean space. A sphere or a torus (ie. the surface of a ball or of a donut, respectively), locally look like the flat 2D plane. There are also 3D manifolds, which viewed from close up look like the familiar 3D Euclidean space given by three perpendicular coordinate axes. And since it's mathematically possible to think of Euclidean space in any dimension you like (just use n coordinates rather than just three), there are manifolds of any dimension, too. (You can read more about Euclidean geometry here.)
Although we intuitively imagine surfaces sitting within ordinary 3D Euclidean space, you can define manifolds in a more abstract way that doesn't depend upon the larger space in which they sit. In this case, distances between points in the manifold can be defined intrinsically, as the shortest path between them that is contained within the manifold. For example, we define distances between two points on the surface of the Earth as the distance between them along a great circle rather than as the distance between them in three-dimensional space. Manifolds with such an intrinsic distance measurement that is defined smoothly over the whole manifold are called Riemannian manifolds.
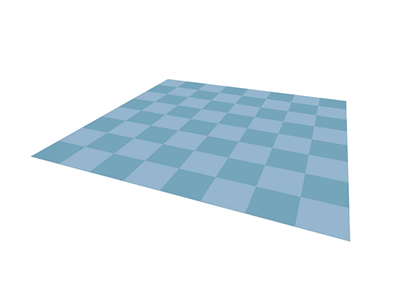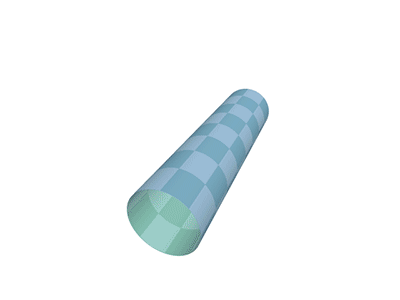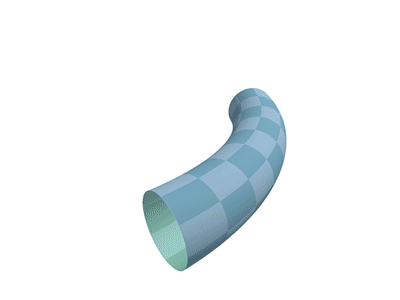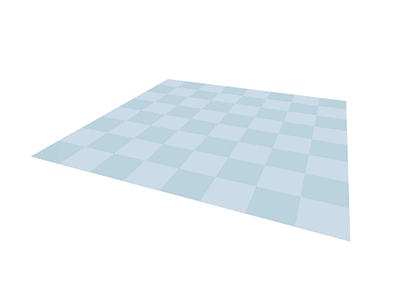
A rectangle can be bent to form a cylinder without stretching or shrinking. However, when the cylinder is bent into a torus, the lengths are no longer preserved, as seen in the distortions of the checkerboard pattern.
Two manifolds are isometric if you can turn one into another without any stretching or shrinking (note that this only makes sense for Riemannian manifolds, in order to have a sense of whether the distances are being distorted). For example, a rectangular piece of paper is isometric to a cylinder since you can join two opposite sides of the paper to create a cylinder without any stretching or shrinking. This isometry means that distances and angles measured on the two surfaces are the same. (Watch this wonderful video to see how isometry can help you eat pizza.)
Now if you join the two ends of the cylinder you will form a torus, but you'll find you'll need to stretch the material on the outside of the torus or shrink the material on the inside of the whole (as can be seen in the distortion of the checkerboard pattern in the picture on the left). This means that pairs of points that were the same distance apart on the cylinder could now be separated by different distances on the torus, if one pair was on the inside and one on the outside of the torus. So, the torus is not isometric to the cylinder, and moreover, the torus is not isometric to the square.
Similarly, although the sphere looks flat close up, you can't turn a flat piece of paper into a sphere without stretching or shrinking (which corresponds to our folds and gathers when we tried to wrap a ball with wrapping paper). As we noticed before, the sphere is curved (described as having positive curvature) and the paper is flat (it has zero curvature) – and this difference in curvature explains why they are not isometric. (You can read more about curvature here and here.) And although the total curvature of a torus is actually the same as the flat piece of paper, the local negative curvature (this is the name for curvature that is saddle-shaped) on the inner side of the torus and positive curvature on its outer side cause the shrinking and stretching that change the distances between points and break the isometry. If you tried to create a torus out of a stiff piece of paper while preserving distances, your paper would buckle into kinks and creases.
Although the sphere and the torus aren't themselves isometric to 2D Euclidean space, Nash and Nirenberg proved many results that showed you can isometrically embed these sort of surfaces (ie. Riemannian manifolds) in flat space: they found ways to bend flat space, without shrinking or stretching it, so that it formed a shape that was as close to the target curved surface as you wished, and with no sharp creases or kinks. This seems surprising but it's possible if the flat space is bunched up into many many corrugations.
The Hevea Torus (Film and images courtesy of the Hevea Project)
You can see this in the beautiful Hevea Torus, an isometric embedding of the torus into flat 2D space. This torus is formed out of corrugated flat space: the wriggles stretching out on the outside of the torus and bunching up on the inside allow the shape of the torus to be formed, while still preserving the geometry (the distances and angles) of flat space. And this embedding can be made as close to the shape of the smooth ideal torus as you like, by making the corrugations smaller in size, adding in more corrugations as necessary.
The corrugations in the Hevea Torus result from solutions to partial differential equations (PDEs for short), a mathematical tool originally associated with the rates of change in physical processes. (You can read more about PDEs in Universal Pictures.) It was Nash and Nirenberg who pioneered the use of these in these abstract geometrical settings, and the Abel committee awarded them this year's prize for both their result and the techniques they developed to analyse these geometric problems. The committee said: "Their breakthroughs have developed into versatile and robust techniques that have become essential tools for the study of nonlinear partial differential equations. Their impact can be felt in all branches of the theory."
And perhaps their work might also help those of us struggling with our present-wrapping problems – I'm off to patent softly wrinkled wrapping paper in time for Christmas!
You can find out more at the Abel Prize website
Comments
Anonymous
I'm fascinated by the animation of the chequered rectangle turning into a cylinder then into a torus, but would love to see the cylinder given a 180 degree twist before joining up its ends to form a kind of Mobius torus. The chequerboard pattern would presumably be even more distorted, and the ends would line up pale to pale and dark to dark squares presumably.
Come to think of it, can a standard Mobius band wrap anything?
Chris G
willie_2k
I think you'd be interested in the Klein Bottle - a 4-dimensional shape made by connecting two mobius strips.
In the sense presented in the article I can't imagine it wraps anything, but in the spirit of the curiosity of your comment it may be something you'd like to look into.