When physicists talk about Einstein's equation they don't usually mean the famous E=mc2, but another formula, which encapsulates the celebrated general theory of relativity. Einstein published that theory a hundred years ago, in 1915. To celebrate its centenary we asked physicist David Tong of the University of Cambridge to explain what general relativity is and how Einstein's equation expresses it. You can watch his explanation in the video below, or read on.
Start with Newton
The general theory of relativity describes the force of gravity. Einstein wasn't the first to come up with such a theory — back in 1686 Isaac Newton formulated his famous inverse square law of gravitation. Newton's law works perfectly well on small-ish scales: we can use it to calculate how fast an object dropped off a tall building will hurtle to the ground and even to send people to the Moon. But when distances and speeds are very large, or very massive objects are involved, Newton's law becomes inaccurate. It's a good place to start though, as it's easier to describe than Einstein's theory.
Suppose you have two objects, say the Sun and the Earth, with masses $m_1$ and $m_2$ respectively. Write $r$ for the distance between the two objects. Then Newton's law says that the gravitational force $F$ between them is $$F=G\frac{m_1 m_2}{r^2},$$
where $G$ is a fixed number, known as Newton's constant. The formula makes intuitive sense: it tells us that gravity gets weaker over long distances (the larger $r$ the smaller $F$) and that the gravitational force is stronger between more massive objects (the larger either of $m_1$ and $m_2$ the larger $F$).
Different force, same formula
There is another formula which looks very similar, but describes a different force. In 1785 the French physicist Charles-Augustin de Coulomb came up with an equation to capture the electrostatic force $F$ that acts between two charged particles with charges $Q_1$ and $Q_2$:
$$F = \frac{1}{4 \pi \epsilon_0} \frac{Q_1 Q_2}{r^2}.$$ Here $r$ stands for the distance between the two particles and $\epsilon_0$ is a constant which determines the strength of electromagnetism. (It has the fancy name permittivity of free space.)The problem with Newton
Newton's and Coulomb's formulas are nice and neat, but there is a problem. Going back to Newton's law, suppose you took the Earth and the Sun and very quickly moved them further apart. This would make the force acting between them weaker, but, according to the formula, the weakening of the force would happen straight away, the instant you move the two bodies apart. The same goes for Coulomb's law: moving the charged particles apart very quickly would result in an immediate weakening of the electrostatic force between them.
But this can't be true. Einstein's special theory of relativity, proposed ten years before the general theory in 1905, says that nothing in the Universe can travel faster than light — not even the "signal" that communicates that two objects have moved apart and the force should become weaker.
Why we need fields
This is one reason why the classical idea of a force needs replacing in modern physics. Instead, we need to think in terms of something — new objects — that transmit the force between one object and another. This was the great contribution of the British scientist Michael Faraday to theoretical physics. Faraday realised that spread throughout the Universe there are objects we today call fields, which are involved in transmitting a force. Examples are the electric and magnetic fields you are probably familiar with from school.
Albert Einstein (1879-1955) in 1921.
A charged particle gives rise to an electric field, which is "felt" by another particle (which has its own electric field). One particle will move in response to the other's electric field — that's what we call a force. When one particle is quickly moved away from the other, then this causes ripples in the first particle's electric field. The ripples travel through space, at the speed of light, and eventually affect the other particle. In fact, the particle that is moved also generates a magnetic field and emits electromagnetic radiation. The end result is a complex interaction of rippling fields — but the point is that the force is really one particle being affected by ripples propagating through the field of the other.
It took scientists a long time to fully develop this field picture of electromagnetism. The main credit goes to the Scottish scientist James Clerk Maxwell, who not only realised that the electric and magnetic forces were two aspects of a unified force of electromagnetism, but also replaced Coulomb's simple law of electrostatics with four equations that describe how electric and magnetic fields respond to moving charged particles. Maxwell's four formulas are some of the most amazing equations in physics because they capture all there is to know about electricity and magnetism.
Gravity and spacetime
So what about gravity? Just as with electromagnetism there needs to be a field giving rise to what we perceive as the gravitational force acting between two bodies. Einstein's great insight was that this field is made of something we already know about: space and time. Imagine a heavy body, like the Sun, sitting in space. Einstein realised that space isn't just a passive by-stander, but responds to the heavy object by bending. Another body, like the Earth, moving into the dent created by the heavier object will be diverted by that dent. Rather than carrying on moving along a straight line, it will start orbiting the heavier object. Or, if it is sufficiently slow, will crash into it. (It took Einstein many years of struggle to arrive at his theory — see this article to find out more.)
Another lesson of Einstein's theory is that space and time can warp into each other — they are inextricable linked and time, too, can be distorted by massive objects. This is why we talk, not just about the curvature of space, but about the curvature of spacetime.
The equation
The general theory of relativity is captured by a deceptively simple-looking equation: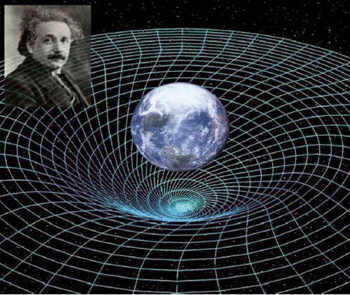 $$R_{\mu \nu} - \frac{1}{2}Rg_{\mu \nu} = \frac{8 \pi G}{ c^4}T_{\mu \nu}.$$ Essentially the equation tells us how a given amount of mass and energy warps spacetime. The left-hand side of the equation, $$R_{\mu \nu} - \frac{1}{2}Rg_{\mu \nu},$$ describes the curvature of spacetime whose effect we perceive as the gravitational force. It's the analogue of the term $F$ on the left-hand side of Newton's equation.
Massive objects bend spacetime. Image courtesy NASA.
Not just one equation
In Einstein's equation the Greek letters $\mu$ and $\nu$ are labels, which can each take on the values 0, 1, 2 or 3. So really, the equation above conceals a whole collection of equations corresponding to the possible combinations of values the $\mu$ and $\nu$ can take: $$R_{0 0} - \frac{1}{2}Rg_{0 0} = \frac{8 \pi G}{c^4}T_{0 0}$$ $$R_{0 1} - \frac{1}{2}Rg_{0 1} = \frac{8 \pi G}{ c^4}T_{0 1}$$ $$R_{1 1} - \frac{1}{2}Rg_{1 1} = \frac{8 \pi G}{ c^4}T_{1 1}$$ and so on. The value of 0 corresponds to time and the values 1,2 and 3 to the three dimensions of space. The equation $$R_{0 1} - \frac{1}{2}Rg_{0 1} = \frac{8 \pi G}{ c^4}T_{0 1}$$ therefore relates to time and the 1-direction of space. The $T$ term on the right-hand side describes the momentum (speed and mass) of matter moving in the 1-direction of space. The motion causes time and the 1-direction of space to mix and warp into each other — that effect is described by the left-hand side of the equation. (The analogue goes for an equation with $\mu=0$ and $\nu$ equal to 2 or 3.) If the equation only has 1s, 2s or 3s, for example $$R_{1 1} - \frac{1}{2}Rg_{1 1} = \frac{8 \pi G}{c^4}T_{1 1},$$ then it relates only to space. The $T$ term on the right-hand side now measures the pressure that matter causes in the corresponding direction of space. The left-hand side tells you how that matter causes space in that direction to stretch. If $\mu$ and $\nu$ both take the value 0, then the equation $$R_{0 0} - \frac{1}{2}Rg_{0 0} = \frac{8 \pi G}{ c^4}T_{0 0}$$ only relates to time. The term $T_{0 0}$ now stands for energy, which causes time to speed up or slow down. The left-hand side of the equation describes that change in the flow of time. Since each of $\mu$ and $\nu$ can take on four values, this gives a total of 4 x 4 =16 equations. However, it turns out that the equation with $\mu = i$ and $\nu=j$ (for $i$ and $j$ each equal to one of 0, 1, 2 or 3) is the same as the equation with $\mu = j$ and $\nu=i.$ This reduces the total number of equations to ten.
An artist's impression of a black hole. Image: Robert Hurt, NASA/JPL-Caltech.
In theory Einstein's equations allow you to work out exactly how massive objects, such as planets, stars, galaxies, or even black holes affect the spacetime they sit in. In practice though, things aren't quite as straight-forward. Einstein's equations are incredibly difficult to solve — supercomputers are needed to find solutions and coming up with new solutions is an active field of theoretical physics. One of the big current challenges is to figure out what happens to space-time when two very heavy objects, like black holes, collide.
How do we know that Einstein's theory is correct? In the hundred years since its publication, the theory has passed every test it has been subjected to. Despite its slightly esoteric nature, it's crucial in things most of us rely on every day, such as the GPS features in our smartphones and the Satnav devices in our cars. The theory does open up some new questions, which is why some physicists think it needs to be modified (see this article). But whether or not this really turns out to be necessary, there's no doubt that general relativity is one of the most amazing achievements in the history of science.
About this article
David Tong is a theoretical physicist at the Universiy of Cambridge. He works on quantum theory and general relativity.
Comments
presentation skill
I really enjoyed this presentation...primarily because it introduces new concepts by a hand-waving, non-rigorous description. I'd really like David Tong to consider creating (or just recommending) a great online course on this subject. Thanks Plus!
MOOC on General relativity
I strongly support the suggestion and urge Dr. Tong to run a MOOC on this subject. Thanks
Yes. This presentation broadend my understanding
Yes; David Tong could enlighten our understanding of Einstein's equations. May be One of us
out of 10,000 can do the thought experiment and, hopefully, understand the math. ; ^)
video
He does a wonderful job explaining things in a way that are easier to understand. I agree, he should have his own online video courses. Excellent teacher! Thanks! Angie
Einstein ignored 3D celestial sphere and refraction of light
General theory of relativity is made based on 4D Minkovski's spacetime, that is violates the basic principle of the modern astronomy (3D celestial sphere), and Einstein ignored the refraction of light.
This can be seen clearly in the Einstein's proving method of deflection of light by the Sun that isn't scientific and deeply wrong:
“Einstein proposed therefore, that photographs be taken of the stars immediately bordering the darkened face of the sun during an eclipse and compared with photographs of those same stars made at another time.” (LincolnBarnett, The Universe and Dr. Einstein, London, June 1949, Preface by Albert Einstein Himself, page 78).
Einstein GR equation on this website
I am confused about the equations you have presented here - especially the General Relativity equations. All other sources in the literature have "8pi" in the numerator ... you write the terms as being in the denominator (on the right hand side). Comments?
Thank you very much for
Thank you very much for spotting this mistake. We have corrected it.
general relativity’s
One of general relativity’s most striking predictions arises if we consider what happens to the universe as a whole.
Shortly after Einstein published his theory, Russian meteorologist and mathematician Alexander Friedmann and Belgian priest Georges Lemaître showed that it predicted that the universe should evolve in response to all the energy it contains. They argued that the universe should start off small and dense, and expand and dilute with time. As a result, galaxies should drift away from each other.
Einstein was initially sceptical of Friedmann and Lemaître’s conclusion, favouring a static universe. But a discovery by the American astronomer Edwin Hubble changed his mind.
Hubble analysed how galaxies recede from the Milky Way. He found that distant galaxies move away faster than those that are relatively nearby. Hubble’s observations showed that the universe was indeed expanding. This model of the cosmos later became known as the big bang.
Over the past 20 years, a plethora of powerful observations by satellites and large telescopes have further firmed up the evidence for an expanding and evolving universe. We have obtained an accurate measure of the expansion rate of the universe and of the temperature of the “relic radiation” left over from the big bang, and we have been able to observe young galaxies when the universe was in its infancy. It is now accepted that the universe is about 13.7 billion years old.
nice
Awesome blog David tong sir can u describe all equations explained by Einstein with examples
theory of relativity
I'm not student of physics, but I'm einstinophillic person and since 1 year I have been in search of simple explanation of theory of relativity, thanks to you because you have completed my search and also you have given backgrounds of STR also due to which your explanation became clear thank you again
Thank you so much for this
Thank you so much for this explanation! I really enjoyed your enthusiasm for physics and how the universe functions altogether. I'm researching about Relativity for my science fair project, hoping to get more insight on the topic. Nonetheless, I appreciate this! It gives me motivation and information.
relativity
The principle of equivalence…
The absence of a gravitational field (true weightlessness) is indistinguishable from free fall acceleration in a gravitational field (apparent weightlessness).
Accelerated motion in the absence of a gravitational field (apparent weight) is indistinguishable from unaccelerated motion in the presence of a gravitational field (true weight). The local effects of gravity are the same as those of being in an accelerating reference frame.
Basically…
Mass-energy curves space-time — a new version of Hooke's law.
Objects trace out world lines that are geodesics (paths of least action in curved space-time) unless acted upon by a net external force — a new version of the law of inertia.
Gravity isn't a force, it's the curvature of space-time caused by the presence of mass-energy.
ut tensio, sic vis
strain ∝ stress
space-time
curvature ∝ mass-energy
stress
The Einstein field equations…
Rμν − 1 Rgμν = 8πG Tμν
2 c4
where…
Rμν = Ricci tensor curvature
R = Ricci scalar curvature
gμν = metric tensor
Tμν = stress-energy tensor
8πG/c4 = a constant of proportionality (something to make the units work out)
That's right, I used the plural form — equations. What looks like one equation is actually a set of ten coupled nonlinear partial differential equations. In reverse adjective order these equations are differential because they deal with rates of change (rates of differing), partial because there are multiple variables involved (multiple parts), nonlinear because some of the operations are repeated (a rate of change of a rate of change), and coupled because they cannot be solved separately (every equation has at least one feature found in another).
Statement of the obvious: Solving these equations turns out to be hard.
Statement of the awesome: These equations can be broken down into simpler equations by those with a lot of skill. Some of these simpler equations are appropriate to the level of this book, which means you can learn how to do some general relativity. They will be derived with minimal to no proof, however.
cosmological constant
Space-time is more than just a set of values for identifying events. Space-time is a thing unto itself. The cosmological constant is a quantity used in general relativity to describe some properties of space-time. Here's how it goes.
Maybe gravity is the curvature of space-time caused by the mass-energy of stuff within it plus the energy of space itself.
Rμν − 1 Rgμν
2
=
8πG Tμ
c4
− Λgμν
space-time
curvature = stress from stuff
in space-time − stress from empty
space-time itself
Or maybe gravity is the curvature of space-time caused by mass-energy on top of the curvature of space-time itself.
Rμν − 1 Rgμν
2
+ Λgμν =
8πG Tμν
c4
curvature from stuff
in space-time + curvature of
space-time itself = mass-energy
stress
Einstein's odd choice of sign might make more sense if you factor out the metric tensor on the left side of the equation. The cosmological constant was invented as a way to hold back gravity so that a static universe wouldn't collapse. (This line of reasoning turns out to be faulty, by the way, but it's a mistake that pays off in the end.)
Rμν − ⎛
⎝ 1 R − Λ ⎞
⎠ gμν = 8πG Tμν
2 c4
Einstein assumed that the universe was static and unchanging. He thought this was true because that was what astronomers at the time thought they saw when they looked out into their telescopes. A static universe would be unstable if gravity was only attractive. Every piece of matter would attract to every other and any slight imbalance in distribution would would force the whole thing to eventually contract down into itself. Einstein added the cosmological constant to his equations (technically, he subtracted it from the scalar curvature) to hold back gravity so that his equations would have a solution that agreed with the static model.
Write more.
Dark energy is spread absolutely smoothly across the universe.
unorganized thoughts
precession of closed (and open) orbits
In 1859 Urbain Le Verrier (1811–1877) France, director of the Paris Observatory published his observations of an anomaly in mercury's orbit. The precession of mercury's perihelion (point of closest approach to the sun) had been precessing at 574 seconds of arc per century. Thinking that this was due to the effects of the other planets he calculated the precession rate using Newton's laws at 531 seconds per century, leaving 43 seconds unaccounted for. Can you say "tiny".
gravitational bending of light
Confirmed by Arthur Eddington (1882–1944) England in 1919. General relativity replaces Newton's theory of universal gravitation as the most complete theory of gravitation. Newton and Eddington were English. Einstein was German. 1919 was the first year after World War I. Anti-German sentiment was still high in Europe. Eddington's confirmation of Einstein's theory showed that science was above culture and politics. Einstein became a celebrity.
Einstein cross
gravitational lensing
magnification of distant objects
Gravity Probe A (1976)
Fly an atomic hydrogen maser on a Scout rocket launched to a height of 10,000 km. A maser is like a laser for microwaves. It produces microwaves of a precise frequency. Measure the doppler shift due to gravity and motion and compare to predicted values (error = 70 ppm = 0.007%)
Gravity Probe B (2004–2005)
Tested for frame dragging.
evolution of the universe
The Friedman equation (1923). The standard model of cosmology. A single ordinary differential equation that comes out of the ten coupled nonlinear partial differential equations of Einstein.
1 ⎛
⎝ da ⎞2
⎠ = 8πG ρa2 − k
c2 dt 3c2
where…
a = scale factor (size of a characteristic piece of the universe, can be any size)
da/dt = rate of change of scale factor (measured by the redshift)
ρ = mass-energy density of the universe (matter-radiation density of the universe)
k = curvature of the universe? geometry of the universe?
+1 closed
+0 flat
−1 open
Λ = cosmological constant (energy density of space itself, empty space)
c = speed of light in a vacuum
G = universal gravitational constant
π = the famous constant from geometry
Hubble constant, Hubble parameter, expansion rate
H = da/dt
a
The Friedman equation again.
1 ⎛
⎝ da ⎞2
⎠ = 8πGρa2 − k
c2 dt 3c2
⎛
⎝ da/dt ⎞2
⎠ = 8πGρ − kc2
a 3 a2
H2 = 8πGρ − kc2 + Λ
3 a2 3
Critical density.
ρc = 3H2
8πG
Density parameter.
Ω = ρ
ρc
Big bang. Georges Lemaître.
time dilation
Time runs slower for a moving object than a stationary one. This consequence of Einstein's theory of special relativity is known as time dilation and it works like this…
t' = t
√(1 − v2/c2)
where…
t = duration of an event in a moving reference frame
t' = duration of the same event relative to a stationary reference frame
v = speed of the moving moving reference frame
c = speed of light in a vacuum (a universal, and apparently unchanging constant)
The greater the speed of the moving observer, the closer the ratio v2/c2 is to one, the closer the denominator √(1 − v2/c2) is to zero, the more the time dilates, stretches, enlarges, or expands. From the point of view of a stationary observer, all events in a frame of reference moving at the speed of light take an infinite amount of time to occur. No events can transpire. Nothing can happen. Time ceases to exist.
Time also runs slower in a gravitational field. This is a consequence of Einstein's general theory of relativity and is known as gravitational time dilation. It works like this…
t' = t
√(1 − 2Vg/c2)
Where Vg is the gravitational potential associated with the gravitational field at some location. If you read the section in this book on gravitational potential energy, you may recall that…
Vg = − Gm
r
If you didn't read that section just hear me when I say that, because of that equation (and ignoring the minus sign), gravitational time dilation works like this…
t' = t
√(1 − 2Gm/rc2)
where…
t = duration of an event in the gravitational field of some object (a planet, a sun, a black hole)
t' = duration of the same event when viewed from infinitely far away (from a location where the gravitational field from the object is zero)
m = mass of the gravitating object
r = distance from the gravitating object to where the event is occurring (their separation)
c = speed of light in a vacuum (a universal, and apparently unchanging constant)
G = universal gravitational constant (another universal, and apparently unchanging constant)
This equation says that the closer an event occurs to a gravitating body, the slower time runs; the greater the mass of the gravitating body, the slower time runs; the stronger gravity is, the slower time runs.
For small distance changes, this approximation works pretty well…
t' ≈ t
√(1 − 2g∆h/c2)
where…
t = duration of an event in the gravitational field of some object (a planet, a sun, a black hole)
t' = duration of the same event when viewed from slightly higher up
g = local gravitational field (local acceleration due to gravity)
∆h = height difference between the event and the observer
c = speed of light in a vacuum
Clocks on planes experiment
Prediction Abstract: During October 1971, four cesium beam atomic clocks were flown on regularly scheduled commercial jet flights around the world twice, once eastward and once westward, to test Einstein's theory of relativity with macroscopic clocks. From the actual flight paths of each trip, the theory predicts that the flying clocks, compared with reference clocks at the US Naval Observatory, should have lost 40 ± 23 nanoseconds during the eastward trip, and should have gained 275 ± 21 nanoseconds during the westward trip. Results Abstract: Four cesium beam clocks flown around the world on commercial jet flights during October 1971, once eastward and once westward, recorded directionally dependent time differences which are in good agreement with predictions of conventional relativity theory. Relative to the atomic time scale of the U.S. Naval Observatory, the flying clocks lost 59 ± 10 nanoseconds during the eastward trip and gained 273 ± 7 nanoseconds during the westward trip, where the errors are the corresponding standard deviations. These results provide an unambiguous empirical resolution of the famous clock "paradox" with macroscopic clocks.
GPS
event horizon
Whatever makes 2Gm/rc2 approach one, makes the dominator √(1 − 2Gm/rc2) approach zero, and makes the time of an event stretch out to infinity. That happens when an event is approaches the following distance from a gravitating body…
rs = 2Gm
c2
This distance is known as the Schwarzschild radius. Another way to write the equation for gravitational time dilation is in terms of this number.
t' = t
√(1 − rs/r)
The Schwarzschild radius divides space-time into two regions separated by an event horizon. The horizon on the earth divides the surface of the Earth into two regions — one that can be seen and one that cannot. The event horizon divides space-time up into two regions — an outside where information flows in any direction and an inside where information can flow in but not out. On the Earth, a horizon is associated with an observer. In space-time, an event horizon is associated with a source of extreme gravity.
The Schwarzschild radius divides space-time
space time name description
r > rs t' > t outside time slows down, events at this distance take longer to occur when viewed from locations further outside
r = rs t' = ∞ event horizon time stops, all events take an infinite amount of time to occur when viewed from outside
r < rs t' = bi t inside time is mathematically imaginary, time becomes space-like, space becomes time-like (bi is an imaginary number composed of a real coefficient b multiplied by the imaginary unit i where i2 = −1)
r = 0 t' = 0 singularity time has no meaning, all events happen simultaneously, new physics is needed
Most objects do not have an event horizon. It is a distance that can not exist. All objects that we encounter in our daily lives and most of the objects in the universe are significantly bigger than their Schwarzschild radius. You cannot get so close to the Earth that time would stop. Its Schwarzschild radius is 9 mm, while its actual radius is 6,400 km. Don't think you could stop time by tunneling down to the Earth's core. Gravity within the Earth decreases to zero at its center. You're not closer to the Earth at its center, you're inside it. When you're on the surface of the Earth like you are now, gravity overall pulls you one way — down. If you could go to the center of the Earth, gravity would pull you outward in all directions, which is the same as no direction. Gravity that doesn't pull in any direction can't be strong.
Let's try a bigger object with bigger gravity — the sun. The Schwarzschild radius of the sun is 3 km, but its actual radius is 700,000 km. That's not much better. Try the heaviest star known — RMC 136a1. It's 315 times more massive but only 30 times bigger across. Its Schwarzschild radius is 930 km, which is still much smaller than its radius.
The problem (which really isn't a problem) is that the all objects around us and the majority of celestial bodies like planets, moons, asteroids, comets, nebulae, and stars can't be made sufficiently small enough. The sun will die one day and its core will shrink down over billions of years to the size of the Earth, but that's where it will end. The Earth might be blown to smithereens by escaping gas from the dying sun, but it will never be crushed symmetrically into a ball bearing. There essentially is no way to get the sun's radius to 3 km or the Earth's to 9 mm. RMC 136a1 is a different story, however.
Stars are miasmas of incandescent plasma as the song goes. They're heated from within by the fusion of light elements into heavier ones. That heat keeps them inflated, in a certain sense. When they exhaust their fuel, they lose that heat and start to shrink. For stars like the sun, hydrogen fuses into helium in the core where pressures are high enough. When all of the core has turned into helium, the star loses the energy needed to keep it pumped up and it starts to shrink.
The sun will shrink until the spaces between atoms are as small as they can get. Such a star is called a white dwarf. Imagine the sun shrunk down to the size of the Earth. We're still 1,000 times or 3 orders of magnitude too big for an event horizon to form.
In the process of shrinking, the sun will also shed a good portion of its outer layers. That produces a nebulous cloud of incandescent gas surrounding the white dwarf core called a planetary nebula. That's an unfortunate term since it has nothing to directly to do with planetary formation.
Bigger stars have more complicated lifestyles. Some of them can go on extracting nuclear energy by fusing three helium nuclei to form one carbon nucleus. Some will tack additional helium nuclei on to this carbon to form oxygen, neon, magnesium, silicon, sulfur, argon and so on all the way up to iron. Such stars can die in one of two ways. Both involve collapse of the core and the shedding of outer layers. Such a dying star is called a supernova and its a process that happens much more quickly than the death of stars like the sun — in hours rather than millennia. The remnant core could form a white dwarf if too much of the surface material was ejected, but the more likely outcome is a neutron star or a black hole.
A neutron star is a remnant stellar core with enough mass that its gravitational field is strong enough to overcome electron degeneracy pressure — the quantum mechanical equivalent of the repulsive electrostatic force between electrons. This crushes the orbiting electrons down into the nucleus where they join with protons to form neutrons. Such a star is effectively a giant ball of neutrons. Imagine a stellar core 2 or 3 times the mass of the sun crushed down to the size of a city, say 10 km in radius. The Schwarzschild radius of a 3 solar mass object is 9 km. We're almost there.
When some really large stars collapse, their remnant cores contain enough mass that gravity will eventually overcome neutron degeneracy pressure — the aspect of the strong nuclear force that keeps neutrons and protons a respectable distance apart. Now there is nothing left to act against gravity and the core crushes itself to zero radius and volume. Not just very small, but actual mathematical zero. Such an object is called a black hole because nothing, not even light, can escape its gravitational hold.
Back to RMC 136a1?
Recall that in the section of this book dealing with gravitational potential energy, that was how the Schwarzschild radius was derived — as the distance from a massive compact object where the escape velocity would equal the speed of light. To this we just added another feature. It's the place where time stops.
gravitational doppler effect
Motional doppler (special relativity)
λ = f0 = √ ⎛
⎝ 1 + v/c ⎞
⎠
λ0 f 1 − v/c
Gravitational doppler (general relativity)
f = f0 ⎛
⎝ 1 + Vg ⎞
⎠ = f0 ⎛
⎝ 1 + Gm ⎞
⎠ ≈ f0 ⎛
⎝ 1 + gΔh ⎞
⎠
c2 rc2 c2
1959 Harvard Tower Experiment. Pound, Rebka, and Snyder. Jefferson Physical Laboratory, Harvard. Confirmed in an experiment conducted in an elevator(?) shaft at Harvard University by Robert Pound (1919–2010) and Glen Rebka (1931–0000) in 1959. A source of gamma rays was placed at the top of the shaft and a detector at the bottom. The source produced gamma rays of a precise frequency and the detector was designed to detect only gamma rays with that particular frequency. In the process of "falling" down the shaft, the gamma rays were blue shifted to a higher frequency. Pound and Rebka placed the source on a vibrating speaker. When the speaker moved up at the right velocity, the gravitational blue shift was canceled by the motional red shift and the detector would detect the gamma rays. Move with any other velocity and noting is detected. Measure the speed of the source, the local gravitational field, height of detector above emitter, and the speed of light; put numbers into equation; check to see if both sides equal to within the limits of experimental error (~10%, Pound and Snider reduced this to ~1% in 1964).
1976 Scout Rocket Experiment. Smithsonian Astrophysical Observatory. The first such experiment was the National Aeronautics and Space Administration/Smithsonian Astrophysical Observatory (NASA-SAO) Rocket Redshift Experiment that took place in June 1976. A hydrogen-maser clock was flown on a rocket to an altitude of about 10,000 km and its frequency compared to a similar clock on the ground. At this height, a clock should run 4.5 parts in 1010 faster than one on the Earth. During two hours of free fall from its maximum height, the rocket transmitted timing pulses from a maser oscillator which acted as a clock and which was compared with a similar clock on the ground. This result confirmed the gravitational time dilation relationship to within 0.01%.
gravitational waves
binary pulsars spiraling into one another
indirect evidence
Joseph Taylor and Russell Hulse
suspended aluminum cylinder
false positive
discovered for real in 2015, reported in 2016
interferometer
LIGO (Laser Interferometer Gravitational Wave Observatory), Advanced LIGO
The Laser Interferometer Gravitational-Wave Observatory (LIGO) is a facility dedicated to the detection of cosmic gravitational waves and the harnessing of these waves for scientific research. It consists of two widely separated installations within the United States — one in Hanford Washington and the other in Livingston, Louisiana — operated in unison as a single observatory
Virgo, Advanced Virgo
The Virgo detector for gravitational waves consists mainly in a Michelson laser interferometer made of two orthogonal arms being each 3 kilometers long. Multiple reflections between mirrors located at the extremities of each arm extend the effective optical length of each arm up to 120 kilometers. Virgo is located within the site of EGO, European Gravitational Observatory, based at Cascina, near Pisa on the river Arno plain. The frequency range of Virgo extends from 10 to 6,000 Hz. This range as well as the high sensitivity should allow detection of gravitational radiation produced by supernovae and coalescence of binary systems in the milky way and in outer galaxies, for instance from the Virgo cluster.
LISA (Laser Interferometer Space Antenna) proposed launch date 2018~2020
LISA consists of three identical spacecraft whose positions mark the vertices of an equilateral triangle five million km on a side, in orbit around the Sun. LISA can be thought of as a giant Michelson interferometer in space. The spacecraft separation sets the range of GW frequencies LISA can observe (from 0.03 milliHertz to above 0.1 Hertz). The center of the LISA triangle traces an Earth-like orbit in the ecliptic plane, one astronomical unit from the Sun, but 20 degrees behind Earth. The plane of the triangle is inclined at 60 degrees to the ecliptic. The natural free-fall orbits of the three spacecraft around the Sun maintain this triangular formation, with the triangle appearing to rotate about its center once per year.